
Question Number 143635 by SOMEDAVONG last updated on 16/Jun/21
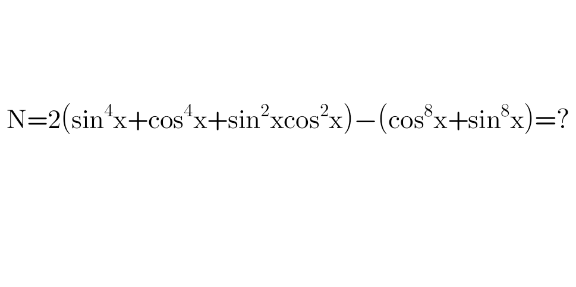
Answered by TheHoneyCat last updated on 16/Jun/21
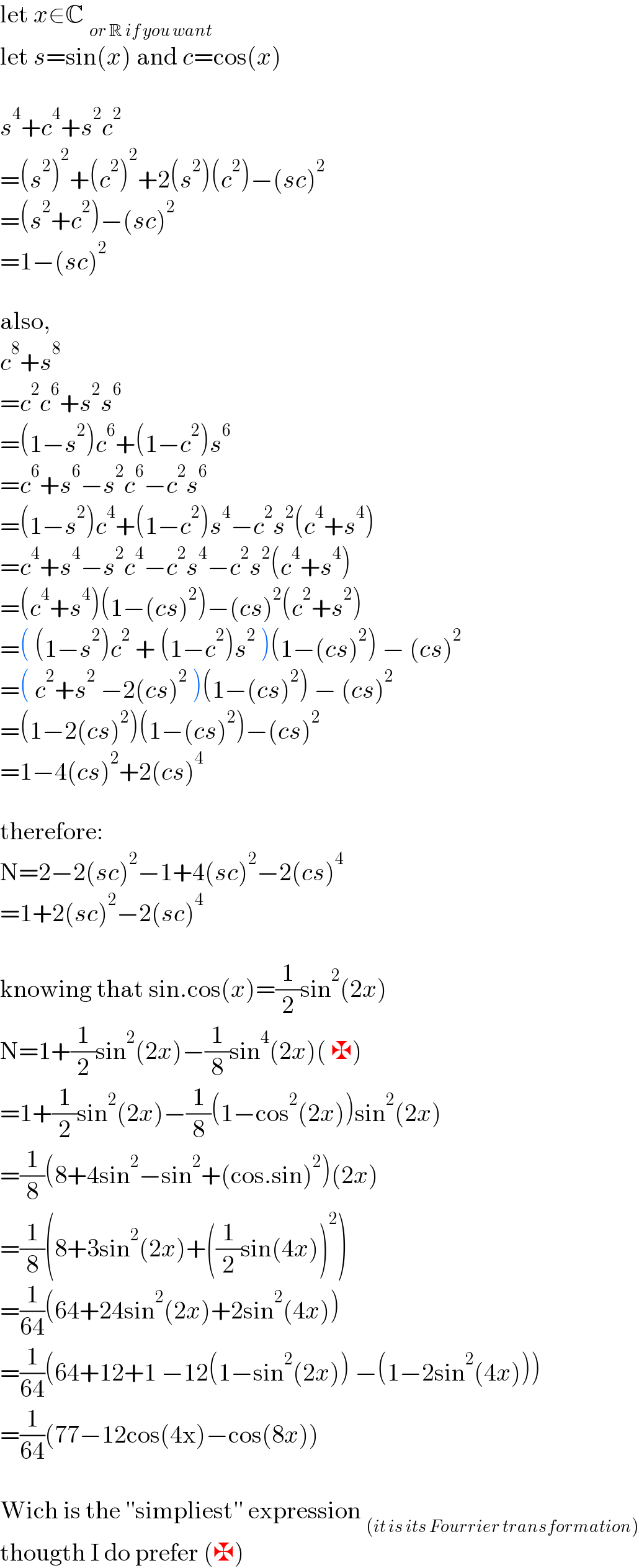
$$\mathrm{let}\:{x}\in\mathbb{C}\:_{{or}\:\mathbb{R}\:{if}\:{you}\:{want}} \\ $$$$\mathrm{let}\:{s}=\mathrm{sin}\left({x}\right)\:\mathrm{and}\:{c}=\mathrm{cos}\left({x}\right) \\ $$$$ \\ $$$${s}^{\mathrm{4}} +{c}^{\mathrm{4}} +{s}^{\mathrm{2}} {c}^{\mathrm{2}} \\ $$$$=\left({s}^{\mathrm{2}} \right)^{\mathrm{2}} +\left({c}^{\mathrm{2}} \right)^{\mathrm{2}} +\mathrm{2}\left({s}^{\mathrm{2}} \right)\left({c}^{\mathrm{2}} \right)−\left({sc}\right)^{\mathrm{2}} \\ $$$$=\left({s}^{\mathrm{2}} +{c}^{\mathrm{2}} \right)−\left({sc}\right)^{\mathrm{2}} \\ $$$$=\mathrm{1}−\left({sc}\right)^{\mathrm{2}} \\ $$$$ \\ $$$$\mathrm{also}, \\ $$$${c}^{\mathrm{8}} +{s}^{\mathrm{8}} \\ $$$$={c}^{\mathrm{2}} {c}^{\mathrm{6}} +{s}^{\mathrm{2}} {s}^{\mathrm{6}} \\ $$$$=\left(\mathrm{1}−{s}^{\mathrm{2}} \right){c}^{\mathrm{6}} +\left(\mathrm{1}−{c}^{\mathrm{2}} \right){s}^{\mathrm{6}} \\ $$$$={c}^{\mathrm{6}} +{s}^{\mathrm{6}} −{s}^{\mathrm{2}} {c}^{\mathrm{6}} −{c}^{\mathrm{2}} {s}^{\mathrm{6}} \\ $$$$=\left(\mathrm{1}−{s}^{\mathrm{2}} \right){c}^{\mathrm{4}} +\left(\mathrm{1}−{c}^{\mathrm{2}} \right){s}^{\mathrm{4}} −{c}^{\mathrm{2}} {s}^{\mathrm{2}} \left({c}^{\mathrm{4}} +{s}^{\mathrm{4}} \right) \\ $$$$={c}^{\mathrm{4}} +{s}^{\mathrm{4}} −{s}^{\mathrm{2}} {c}^{\mathrm{4}} −{c}^{\mathrm{2}} {s}^{\mathrm{4}} −{c}^{\mathrm{2}} {s}^{\mathrm{2}} \left({c}^{\mathrm{4}} +{s}^{\mathrm{4}} \right) \\ $$$$=\left({c}^{\mathrm{4}} +{s}^{\mathrm{4}} \right)\left(\mathrm{1}−\left({cs}\right)^{\mathrm{2}} \right)−\left({cs}\right)^{\mathrm{2}} \left({c}^{\mathrm{2}} +{s}^{\mathrm{2}} \right) \\ $$$$=\left(\:\left(\mathrm{1}−{s}^{\mathrm{2}} \right){c}^{\mathrm{2}} \:+\:\left(\mathrm{1}−{c}^{\mathrm{2}} \right){s}^{\mathrm{2}} \:\right)\left(\mathrm{1}−\left({cs}\right)^{\mathrm{2}} \right)\:−\:\left({cs}\right)^{\mathrm{2}} \\ $$$$=\left(\:{c}^{\mathrm{2}} +{s}^{\mathrm{2}} \:−\mathrm{2}\left({cs}\right)^{\mathrm{2}} \:\right)\left(\mathrm{1}−\left({cs}\right)^{\mathrm{2}} \right)\:−\:\left({cs}\right)^{\mathrm{2}} \\ $$$$=\left(\mathrm{1}−\mathrm{2}\left({cs}\right)^{\mathrm{2}} \right)\left(\mathrm{1}−\left({cs}\right)^{\mathrm{2}} \right)−\left({cs}\right)^{\mathrm{2}} \\ $$$$=\mathrm{1}−\mathrm{4}\left({cs}\right)^{\mathrm{2}} +\mathrm{2}\left({cs}\right)^{\mathrm{4}} \\ $$$$ \\ $$$$\mathrm{therefore}: \\ $$$$\mathrm{N}=\mathrm{2}−\mathrm{2}\left({sc}\right)^{\mathrm{2}} −\mathrm{1}+\mathrm{4}\left({sc}\right)^{\mathrm{2}} −\mathrm{2}\left({cs}\right)^{\mathrm{4}} \\ $$$$=\mathrm{1}+\mathrm{2}\left({sc}\right)^{\mathrm{2}} −\mathrm{2}\left({sc}\right)^{\mathrm{4}} \\ $$$$ \\ $$$$\mathrm{knowing}\:\mathrm{that}\:\mathrm{sin}.\mathrm{cos}\left({x}\right)=\frac{\mathrm{1}}{\mathrm{2}}\mathrm{sin}^{\mathrm{2}} \left(\mathrm{2}{x}\right) \\ $$$$\mathrm{N}=\mathrm{1}+\frac{\mathrm{1}}{\mathrm{2}}\mathrm{sin}^{\mathrm{2}} \left(\mathrm{2}{x}\right)−\frac{\mathrm{1}}{\mathrm{8}}\mathrm{sin}^{\mathrm{4}} \left(\mathrm{2}{x}\right)\left(\:\maltese\right) \\ $$$$=\mathrm{1}+\frac{\mathrm{1}}{\mathrm{2}}\mathrm{sin}^{\mathrm{2}} \left(\mathrm{2}{x}\right)−\frac{\mathrm{1}}{\mathrm{8}}\left(\mathrm{1}−\mathrm{cos}^{\mathrm{2}} \left(\mathrm{2}{x}\right)\right)\mathrm{sin}^{\mathrm{2}} \left(\mathrm{2}{x}\right) \\ $$$$=\frac{\mathrm{1}}{\mathrm{8}}\left(\mathrm{8}+\mathrm{4sin}^{\mathrm{2}} −\mathrm{sin}^{\mathrm{2}} +\left(\mathrm{cos}.\mathrm{sin}\right)^{\mathrm{2}} \right)\left(\mathrm{2}{x}\right) \\ $$$$=\frac{\mathrm{1}}{\mathrm{8}}\left(\mathrm{8}+\mathrm{3sin}^{\mathrm{2}} \left(\mathrm{2}{x}\right)+\left(\frac{\mathrm{1}}{\mathrm{2}}\mathrm{sin}\left(\mathrm{4}{x}\right)\right)^{\mathrm{2}} \right) \\ $$$$=\frac{\mathrm{1}}{\mathrm{64}}\left(\mathrm{64}+\mathrm{24sin}^{\mathrm{2}} \left(\mathrm{2}{x}\right)+\mathrm{2sin}^{\mathrm{2}} \left(\mathrm{4}{x}\right)\right) \\ $$$$=\frac{\mathrm{1}}{\mathrm{64}}\left(\mathrm{64}+\mathrm{12}+\mathrm{1}\:−\mathrm{12}\left(\mathrm{1}−\mathrm{sin}^{\mathrm{2}} \left(\mathrm{2}{x}\right)\right)\:−\left(\mathrm{1}−\mathrm{2sin}^{\mathrm{2}} \left(\mathrm{4}{x}\right)\right)\right) \\ $$$$=\frac{\mathrm{1}}{\mathrm{64}}\left(\mathrm{77}−\mathrm{12cos}\left(\mathrm{4x}\right)−\mathrm{cos}\left(\mathrm{8}{x}\right)\right) \\ $$$$ \\ $$$$\mathrm{Wich}\:\mathrm{is}\:\mathrm{the}\:''\mathrm{simpliest}''\:\mathrm{expression}\:_{\left({it}\:{is}\:{its}\:{Fourrier}\:{transformation}\right)} \\ $$$$\mathrm{thougth}\:\mathrm{I}\:\mathrm{do}\:\mathrm{prefer}\:\left(\maltese\right) \\ $$
