
Question Number 140346 by mathsuji last updated on 06/May/21
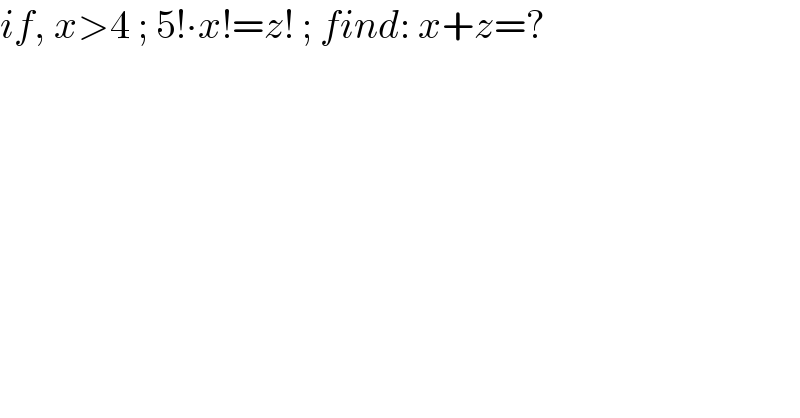
$${if},\:{x}>\mathrm{4}\:;\:\mathrm{5}!\centerdot{x}!={z}!\:;\:{find}:\:{x}+{z}=? \\ $$
Answered by hknkrc46 last updated on 06/May/21
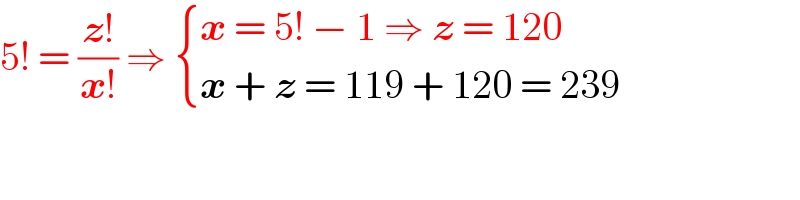
$$\mathrm{5}!\:=\:\frac{\boldsymbol{{z}}!}{\boldsymbol{{x}}!}\:\Rightarrow\:\begin{cases}{\boldsymbol{{x}}\:=\:\mathrm{5}!\:−\:\mathrm{1}\:\Rightarrow\:\boldsymbol{{z}}\:=\:\mathrm{120}}\\{\boldsymbol{{x}}\:+\:\boldsymbol{{z}}\:=\:\mathrm{119}\:+\:\mathrm{120}\:=\:\mathrm{239}}\end{cases} \\ $$
Commented bymathsuji last updated on 07/May/21
$${thankyou}\:{sir}\:{cool} \\ $$
Answered by mr W last updated on 06/May/21
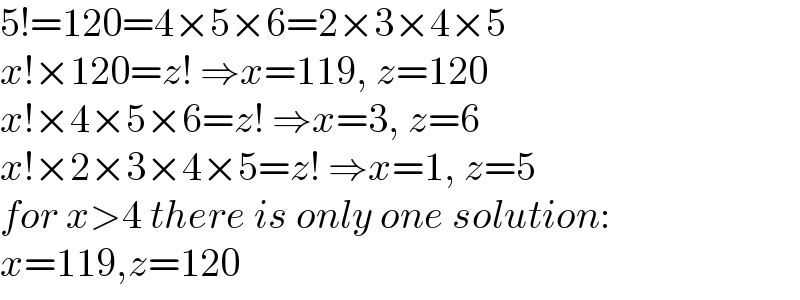
$$\mathrm{5}!=\mathrm{120}=\mathrm{4}×\mathrm{5}×\mathrm{6}=\mathrm{2}×\mathrm{3}×\mathrm{4}×\mathrm{5} \\ $$ $${x}!×\mathrm{120}={z}!\:\Rightarrow{x}=\mathrm{119},\:{z}=\mathrm{120} \\ $$ $${x}!×\mathrm{4}×\mathrm{5}×\mathrm{6}={z}!\:\Rightarrow{x}=\mathrm{3},\:{z}=\mathrm{6} \\ $$ $${x}!×\mathrm{2}×\mathrm{3}×\mathrm{4}×\mathrm{5}={z}!\:\Rightarrow{x}=\mathrm{1},\:{z}=\mathrm{5} \\ $$ $${for}\:{x}>\mathrm{4}\:{there}\:{is}\:{only}\:{one}\:{solution}: \\ $$ $${x}=\mathrm{119},{z}=\mathrm{120} \\ $$
Commented bymathsuji last updated on 07/May/21

$${thakyou}\:{sir}\:{cool} \\ $$
