
Question Number 139544 by melanie last updated on 28/Apr/21
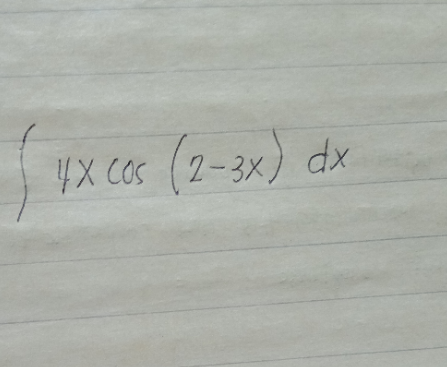
Answered by bemath last updated on 28/Apr/21
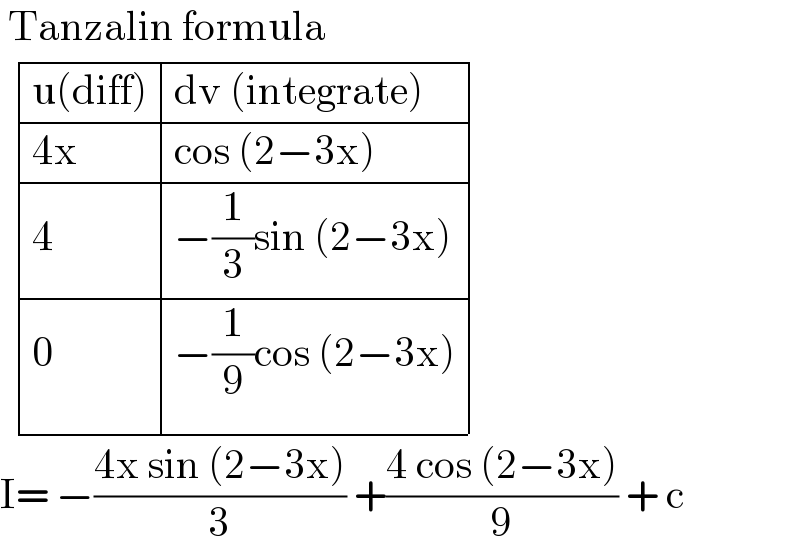
$$\:\mathrm{Tanzalin}\:\mathrm{formula} \\ $$$$\:\begin{array}{|c|c|c|c|}{\mathrm{u}\left(\mathrm{diff}\right)}&\hline{\mathrm{dv}\:\left(\mathrm{integrate}\right)}\\{\mathrm{4x}}&\hline{\mathrm{cos}\:\left(\mathrm{2}−\mathrm{3x}\right)}\\{\mathrm{4}}&\hline{−\frac{\mathrm{1}}{\mathrm{3}}\mathrm{sin}\:\left(\mathrm{2}−\mathrm{3x}\right)}\\{\mathrm{0}}&\hline{−\frac{\mathrm{1}}{\mathrm{9}}\mathrm{cos}\:\left(\mathrm{2}−\mathrm{3x}\right)}\\\hline\end{array} \\ $$$$\mathrm{I}=\:−\frac{\mathrm{4x}\:\mathrm{sin}\:\left(\mathrm{2}−\mathrm{3x}\right)}{\mathrm{3}}\:+\frac{\mathrm{4}\:\mathrm{cos}\:\left(\mathrm{2}−\mathrm{3x}\right)}{\mathrm{9}}\:+\:\mathrm{c}\: \\ $$
Answered by MJS_new last updated on 28/Apr/21
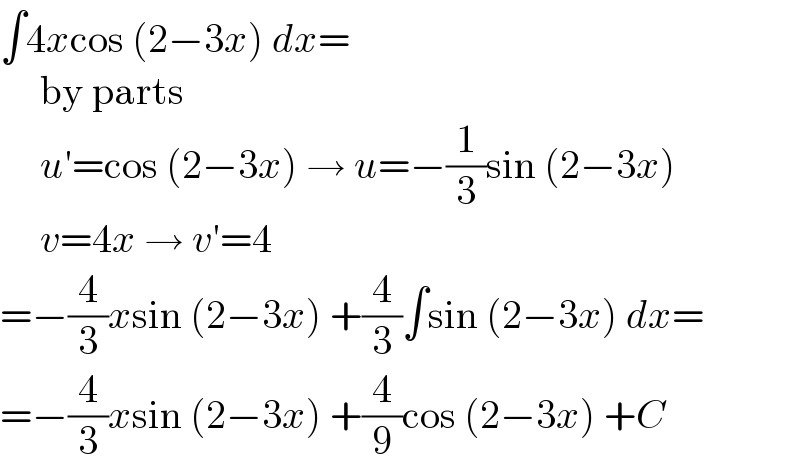
$$\int\mathrm{4}{x}\mathrm{cos}\:\left(\mathrm{2}−\mathrm{3}{x}\right)\:{dx}= \\ $$$$\:\:\:\:\:\mathrm{by}\:\mathrm{parts} \\ $$$$\:\:\:\:\:{u}'=\mathrm{cos}\:\left(\mathrm{2}−\mathrm{3}{x}\right)\:\rightarrow\:{u}=−\frac{\mathrm{1}}{\mathrm{3}}\mathrm{sin}\:\left(\mathrm{2}−\mathrm{3}{x}\right) \\ $$$$\:\:\:\:\:{v}=\mathrm{4}{x}\:\rightarrow\:{v}'=\mathrm{4} \\ $$$$=−\frac{\mathrm{4}}{\mathrm{3}}{x}\mathrm{sin}\:\left(\mathrm{2}−\mathrm{3}{x}\right)\:+\frac{\mathrm{4}}{\mathrm{3}}\int\mathrm{sin}\:\left(\mathrm{2}−\mathrm{3}{x}\right)\:{dx}= \\ $$$$=−\frac{\mathrm{4}}{\mathrm{3}}{x}\mathrm{sin}\:\left(\mathrm{2}−\mathrm{3}{x}\right)\:+\frac{\mathrm{4}}{\mathrm{9}}\mathrm{cos}\:\left(\mathrm{2}−\mathrm{3}{x}\right)\:+{C} \\ $$
