
Question Number 138973 by mathmax by abdo last updated on 20/Apr/21

$$\mathrm{calculate}\:\int_{\mathrm{0}} ^{\infty\:} \:\frac{\mathrm{dx}}{\mathrm{x}^{\mathrm{4}} −\mathrm{x}^{\mathrm{2}} +\mathrm{2}} \\ $$
Answered by mathmax by abdo last updated on 22/Apr/21

$$\Phi=\int_{\mathrm{0}} ^{\infty} \:\frac{\mathrm{dx}}{\mathrm{x}^{\mathrm{4}} −\mathrm{x}^{\mathrm{2}} \:+\mathrm{2}} \\ $$$$\mathrm{let}\:\mathrm{f}\left(\mathrm{x}\right)=\frac{\mathrm{1}}{\mathrm{x}^{\mathrm{4}} −\mathrm{x}^{\mathrm{2}} +\mathrm{2}} \\ $$$$\mathrm{x}^{\mathrm{4}} −\mathrm{x}^{\mathrm{2}} +\mathrm{2}=\mathrm{0}\:\rightarrow\mathrm{t}^{\mathrm{2}} −\mathrm{t}+\mathrm{2}=\mathrm{0}\:\rightarrow\Delta=\mathrm{1}−\mathrm{8}=−\mathrm{7}\:\Rightarrow\mathrm{t}_{\mathrm{1}} =\frac{\mathrm{1}+\mathrm{i}\sqrt{\mathrm{7}}}{\mathrm{2}} \\ $$$$\mathrm{ant}\:\mathrm{t}_{\mathrm{2}} =\frac{\mathrm{1}−\mathrm{i}\sqrt{\mathrm{7}}}{\mathrm{2}}\:\Rightarrow\mathrm{f}\left(\mathrm{x}\right)=\frac{\mathrm{1}}{\left(\mathrm{x}^{\mathrm{2}} −\mathrm{t}_{\mathrm{1}} \right)\left(\mathrm{x}^{\mathrm{2}} −\mathrm{t}_{\mathrm{2}} \right)}\:=\frac{\mathrm{1}}{\mathrm{t}_{\mathrm{1}} −\mathrm{t}_{\mathrm{2}} }\left(\frac{\mathrm{1}}{\mathrm{x}^{\mathrm{2}} −\mathrm{t}_{\mathrm{1}} }−\frac{\mathrm{1}}{\mathrm{x}^{\mathrm{2}} −\mathrm{t}_{\mathrm{2}} }\right) \\ $$$$=\frac{\mathrm{1}}{\mathrm{i}\sqrt{\mathrm{7}}}\left(\frac{\mathrm{1}}{\mathrm{x}^{\mathrm{2}} −\mathrm{t}_{\mathrm{1}} }−\frac{\mathrm{1}}{\mathrm{x}^{\mathrm{2}} −\mathrm{t}_{\mathrm{2}} }\right)\:\Rightarrow\Phi=\frac{\mathrm{1}}{\mathrm{i}\sqrt{\mathrm{7}}}\int_{\mathrm{0}} ^{\infty} \:\:\frac{\mathrm{dx}}{\mathrm{x}^{\mathrm{2}} −\mathrm{t}_{\mathrm{1}} }−\frac{\mathrm{1}}{\mathrm{i}\sqrt{\mathrm{7}}}\int_{\mathrm{0}} ^{\infty} \:\frac{\mathrm{dx}}{\mathrm{x}^{\mathrm{2}} −\mathrm{t}_{\mathrm{2}} } \\ $$$$=\Phi_{\mathrm{1}} −\Phi_{\mathrm{2}} \\ $$$$\Phi_{\mathrm{1}} =\frac{\mathrm{1}}{\mathrm{2i}\sqrt{\mathrm{7}}}\int_{−\infty} ^{+\infty} \:\frac{\mathrm{dx}}{\mathrm{x}^{\mathrm{2}} −\mathrm{t}_{\mathrm{1}} }\:\mathrm{let}\:\mathrm{w}\left(\mathrm{z}\right)=\frac{\mathrm{1}}{\mathrm{z}^{\mathrm{2}} −\frac{\mathrm{1}+\mathrm{i}\sqrt{\mathrm{7}}}{\mathrm{2}}} \\ $$$$\mathrm{we}\:\mathrm{have}\:\mid\frac{\mathrm{1}+\mathrm{i}\sqrt{\mathrm{7}}}{\mathrm{2}}\mid=\frac{\mathrm{1}}{\mathrm{2}}\sqrt{\mathrm{1}+\mathrm{7}}=\frac{\mathrm{2}\sqrt{\mathrm{2}}}{\mathrm{2}}=\sqrt{\mathrm{2}}\:\Rightarrow\frac{\mathrm{1}+\mathrm{i}\sqrt{\mathrm{7}}}{\mathrm{2}}=\sqrt{\mathrm{2}}\mathrm{e}^{\mathrm{iarctan}\left(\sqrt{\mathrm{7}}\right)} \\ $$$$\Rightarrow\mathrm{w}\left(\mathrm{z}\right)=\frac{\mathrm{1}}{\left(\mathrm{z}−\left(^{\mathrm{4}} \sqrt{\mathrm{2}}\right)\mathrm{e}^{\frac{\mathrm{i}}{\mathrm{2}}\mathrm{arctan}\left(\sqrt{\mathrm{7}}\right)} \right)\left(\mathrm{z}+\left(^{\mathrm{4}} \sqrt{\mathrm{2}}\right)\mathrm{e}^{\frac{\mathrm{i}}{\mathrm{2}}\mathrm{arctan}\left(\sqrt{\mathrm{7}}\right)} \right)} \\ $$$$\int_{−\infty} ^{+\infty} \:\mathrm{w}\left(\mathrm{z}\right)\mathrm{dx}\:=\mathrm{2i}\pi\:\mathrm{Res}\left(\mathrm{w},\left(^{\mathrm{4}} \sqrt{\mathrm{2}}\mathrm{e}^{\frac{\mathrm{i}}{\mathrm{2}}\mathrm{arctan}\left(\sqrt{\mathrm{7}}\right)} \right)\right. \\ $$$$=\mathrm{2i}\pi.\frac{\mathrm{1}}{\mathrm{2}\left(^{\mathrm{4}} \sqrt{\mathrm{2}}\right)\mathrm{e}^{\frac{\mathrm{i}}{\mathrm{2}}\mathrm{arctan}\left(\sqrt{\mathrm{7}}\right)} }\:=\frac{\mathrm{i}\pi}{\left(^{\mathrm{4}} \sqrt{\mathrm{2}}\right)}\mathrm{e}^{−\frac{\mathrm{i}}{\mathrm{2}}\mathrm{arctan}\left(\sqrt{\mathrm{7}}\right)} \:\Rightarrow \\ $$$$\Phi_{\mathrm{1}} =\frac{\mathrm{1}}{\mathrm{2i}\sqrt{\mathrm{7}}}×\frac{\mathrm{i}\pi}{\left(^{\mathrm{4}} \sqrt{\mathrm{2}}\right)}\mathrm{e}^{−\frac{\mathrm{i}}{\mathrm{2}}\mathrm{arctan}\left(\sqrt{\mathrm{7}}\right)} \:=\frac{\pi}{\mathrm{2}\sqrt{\mathrm{7}}\left(^{\mathrm{4}} \sqrt{\mathrm{2}}\right)}\mathrm{e}^{−\frac{\mathrm{i}}{\mathrm{2}}\mathrm{arctan}\left(\sqrt{\mathrm{7}}\right)} \\ $$$$\Phi_{\mathrm{2}} =\frac{\mathrm{1}}{\mathrm{i}\sqrt{\mathrm{7}}}\int_{\mathrm{0}} ^{\infty} \frac{\mathrm{dx}}{\mathrm{x}−\mathrm{t}_{\mathrm{2}} }\:=\frac{\mathrm{1}}{\mathrm{2i}\sqrt{\mathrm{7}}}×\mathrm{conj}\left(\int_{−\infty} ^{\infty} \:\frac{\mathrm{dx}}{\mathrm{x}^{\mathrm{2}} −\mathrm{t}_{\mathrm{1}} }\right) \\ $$$$=\frac{\mathrm{1}}{\mathrm{2i}\sqrt{\mathrm{7}}}×\left(−\frac{\mathrm{i}\pi}{\left(^{\mathrm{4}} \sqrt{\mathrm{2}}\right)}\mathrm{e}^{\frac{\mathrm{i}}{\mathrm{2}}\mathrm{arctan}\left(\sqrt{\mathrm{7}}\right)} \right)\:=−\frac{\pi}{\mathrm{2}\sqrt{\mathrm{7}}\left(^{\mathrm{4}} \sqrt{\mathrm{2}}\right)}\mathrm{e}^{\frac{\mathrm{i}}{\mathrm{2}}\mathrm{arctan}\left(\sqrt{\mathrm{7}}\right)} \:\Rightarrow \\ $$$$\Phi=\Phi_{\mathrm{1}} −\Phi_{\mathrm{2}} =\frac{\pi}{\mathrm{2}\sqrt{\mathrm{7}}\left(^{\mathrm{4}} \sqrt{\mathrm{2}}\right)}\left(\mathrm{e}^{\frac{\mathrm{i}}{\mathrm{2}}\mathrm{arctan}\left(\sqrt{\mathrm{7}}\right)} +\mathrm{e}^{−\frac{\mathrm{i}}{\mathrm{2}}\mathrm{arctan}\left(\sqrt{\mathrm{7}}\right)} \right) \\ $$$$=\frac{\pi}{\mathrm{2}\sqrt{\mathrm{7}}\left(^{\mathrm{4}} \sqrt{\mathrm{2}}\right)}\left(\mathrm{2cos}\left(\mathrm{arctan}\left(\sqrt{\mathrm{7}}\right)\right)\:=\frac{\pi}{\:\sqrt{\mathrm{7}}\left(^{\mathrm{4}} \sqrt{\mathrm{2}}\right)}\mathrm{cos}\left(\mathrm{arctan}\left(\sqrt{\mathrm{7}}\right)\right)\right. \\ $$$$\mathrm{we}\:\mathrm{have}\:\mathrm{cos}\left(\mathrm{arctanx}\right)\:=\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}+\mathrm{x}^{\mathrm{2}} }}\:\Rightarrow\mathrm{cos}\left(\mathrm{arctan}\left(\sqrt{\mathrm{7}}\right)\right) \\ $$$$=\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}+\mathrm{7}}}\:=\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{2}}}\:\Rightarrow\Phi\:=\frac{\pi}{\:\sqrt{\mathrm{7}}\left(^{\mathrm{4}} \sqrt{\mathrm{2}}\right)}×\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{2}}} \\ $$$$=\frac{\pi}{\mathrm{2}\sqrt{\mathrm{14}}\left(^{\mathrm{4}} \sqrt{\mathrm{2}}\right)} \\ $$
Commented by mathmax by abdo last updated on 23/Apr/21
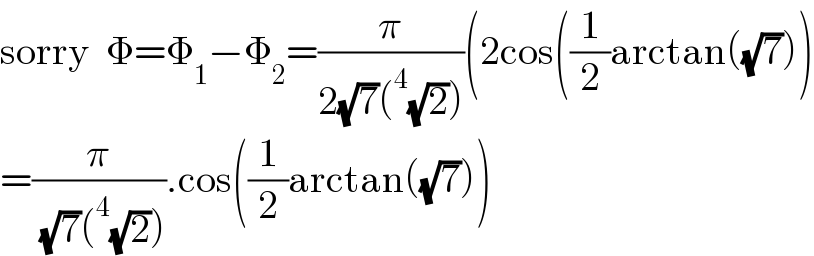
$$\mathrm{sorry}\:\:\Phi=\Phi_{\mathrm{1}} −\Phi_{\mathrm{2}} =\frac{\pi}{\mathrm{2}\sqrt{\mathrm{7}}\left(^{\mathrm{4}} \sqrt{\mathrm{2}}\right)}\left(\mathrm{2cos}\left(\frac{\mathrm{1}}{\mathrm{2}}\mathrm{arctan}\left(\sqrt{\mathrm{7}}\right)\right)\right. \\ $$$$=\frac{\pi}{\:\sqrt{\mathrm{7}}\left(^{\mathrm{4}} \sqrt{\mathrm{2}}\right)}.\mathrm{cos}\left(\frac{\mathrm{1}}{\mathrm{2}}\mathrm{arctan}\left(\sqrt{\mathrm{7}}\right)\right) \\ $$
