
Question Number 138703 by mathocean1 last updated on 16/Apr/21
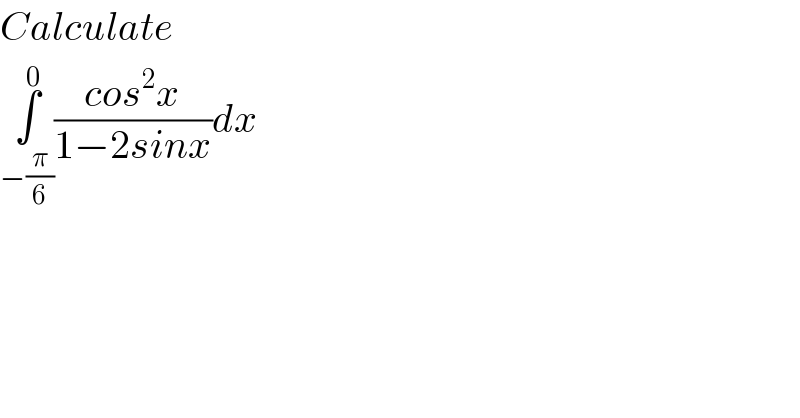
$${Calculate} \\ $$$$\underset{−\frac{\pi}{\mathrm{6}}} {\overset{\mathrm{0}} {\int}}\frac{{cos}^{\mathrm{2}} {x}}{\mathrm{1}−\mathrm{2}{sinx}}{dx} \\ $$
Commented by SanyamJoshi last updated on 17/Apr/21

$${Calculate} \\ $$$$\underset{−\frac{\pi}{\mathrm{6}}} {\overset{\mathrm{0}} {\int}}\frac{{cos}^{\mathrm{2}} {x}}{\mathrm{1}−\mathrm{2}{sinx}}{dx} \\ $$
Answered by Mathspace last updated on 16/Apr/21
![I=∫_(−(π/6)) ^0 ((cos^2 x)/(1−2sinx))dx =_(x=−t) ∫_0 ^(π/6) ((cos^2 t)/(1+2sint))dt =∫_0 ^(π/6) ((1−sin^2 t)/(1+2sint))dt we have ((1−x^2 )/(2x+1))=−(1/4)((4x^2 −4)/(2x+1)) =−(1/4)(((4x^2 −1)/(2x+1))−(3/(2x+1))) =−(1/4)(2x−1)+(3/(4(2x+1))) ⇒ I=−(1/4)∫_0 ^(π/6) (2sint−1)dt +(3/4)∫_0 ^(π/6) (dt/(2sint +1))(=J) =−(1/2)[−cost]_0 ^(π/6) +(1/4).(π/6) +(3/4)J =−(1/2)(1−((√3)/2))+(π/(24))+(3/4)J J=∫_0 ^(π/6) (dt/(2sint +1)) =_(tan((t/2))=y) ∫_0 ^(2−(√3)) ((2dy)/((1+y^2 )(2((2y)/(1+y^2 ))+1))) =2∫_0 ^(2−(√3)) (dy/(4y+y^2 +1)) =2∫_0 ^(2−(√3)) (dy/(y^2 +4y+1)) Δ^′ =2^2 −1=3 ⇒y_1 =−2+(√3) y_2 =−2−(√3) ⇒ 2∫_0 ^(2−(√3)) (dy/((y−y_1 )(y−y_2 ))) =2(1/(2(√3)))∫_0 ^(2−(√3)) ((1/(y−y_1 ))−(1/(y−y_2 )))dy =(1/( (√3)))[log∣((y+2−(√3))/(y+2+(√3)))∣]_0 ^(2−(√3)) =(1/( (√3))){log(((4−2(√3))/4))−log(((2−(√3))/(2+(√3))))} I=−(1/2)+((√3)/4)+(π/(24))+((√3)/4){log(1−((√3)/2))−log(((2−(√3))/(2+(√3))))}](Q138705.png)
$${I}=\int_{−\frac{\pi}{\mathrm{6}}} ^{\mathrm{0}} \:\frac{{cos}^{\mathrm{2}} {x}}{\mathrm{1}−\mathrm{2}{sinx}}{dx} \\ $$$$=_{{x}=−{t}} \int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{6}}} \:\frac{{cos}^{\mathrm{2}} {t}}{\mathrm{1}+\mathrm{2}{sint}}{dt} \\ $$$$=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{6}}} \:\frac{\mathrm{1}−{sin}^{\mathrm{2}} {t}}{\mathrm{1}+\mathrm{2}{sint}}{dt} \\ $$$${we}\:{have}\:\frac{\mathrm{1}−{x}^{\mathrm{2}} }{\mathrm{2}{x}+\mathrm{1}}=−\frac{\mathrm{1}}{\mathrm{4}}\frac{\mathrm{4}{x}^{\mathrm{2}} −\mathrm{4}}{\mathrm{2}{x}+\mathrm{1}} \\ $$$$=−\frac{\mathrm{1}}{\mathrm{4}}\left(\frac{\mathrm{4}{x}^{\mathrm{2}} −\mathrm{1}}{\mathrm{2}{x}+\mathrm{1}}−\frac{\mathrm{3}}{\mathrm{2}{x}+\mathrm{1}}\right) \\ $$$$=−\frac{\mathrm{1}}{\mathrm{4}}\left(\mathrm{2}{x}−\mathrm{1}\right)+\frac{\mathrm{3}}{\mathrm{4}\left(\mathrm{2}{x}+\mathrm{1}\right)}\:\Rightarrow \\ $$$${I}=−\frac{\mathrm{1}}{\mathrm{4}}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{6}}} \left(\mathrm{2}{sint}−\mathrm{1}\right){dt} \\ $$$$+\frac{\mathrm{3}}{\mathrm{4}}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{6}}} \:\frac{{dt}}{\mathrm{2}{sint}\:+\mathrm{1}}\left(={J}\right) \\ $$$$=−\frac{\mathrm{1}}{\mathrm{2}}\left[−{cost}\right]_{\mathrm{0}} ^{\frac{\pi}{\mathrm{6}}} +\frac{\mathrm{1}}{\mathrm{4}}.\frac{\pi}{\mathrm{6}} \\ $$$$+\frac{\mathrm{3}}{\mathrm{4}}{J} \\ $$$$=−\frac{\mathrm{1}}{\mathrm{2}}\left(\mathrm{1}−\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\right)+\frac{\pi}{\mathrm{24}}+\frac{\mathrm{3}}{\mathrm{4}}{J} \\ $$$${J}=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{6}}} \:\frac{{dt}}{\mathrm{2}{sint}\:+\mathrm{1}} \\ $$$$=_{{tan}\left(\frac{{t}}{\mathrm{2}}\right)={y}} \:\:\:\:\int_{\mathrm{0}} ^{\mathrm{2}−\sqrt{\mathrm{3}}} \:\:\:\frac{\mathrm{2}{dy}}{\left(\mathrm{1}+{y}^{\mathrm{2}} \right)\left(\mathrm{2}\frac{\mathrm{2}{y}}{\mathrm{1}+{y}^{\mathrm{2}} }+\mathrm{1}\right)} \\ $$$$=\mathrm{2}\int_{\mathrm{0}} ^{\mathrm{2}−\sqrt{\mathrm{3}}} \:\:\:\:\frac{{dy}}{\mathrm{4}{y}+{y}^{\mathrm{2}} \:+\mathrm{1}} \\ $$$$=\mathrm{2}\int_{\mathrm{0}} ^{\mathrm{2}−\sqrt{\mathrm{3}}} \:\:\:\frac{{dy}}{{y}^{\mathrm{2}} +\mathrm{4}{y}+\mathrm{1}} \\ $$$$\Delta^{'} =\mathrm{2}^{\mathrm{2}} −\mathrm{1}=\mathrm{3}\:\Rightarrow{y}_{\mathrm{1}} =−\mathrm{2}+\sqrt{\mathrm{3}} \\ $$$${y}_{\mathrm{2}} =−\mathrm{2}−\sqrt{\mathrm{3}}\:\Rightarrow \\ $$$$\mathrm{2}\int_{\mathrm{0}} ^{\mathrm{2}−\sqrt{\mathrm{3}}} \:\frac{{dy}}{\left({y}−{y}_{\mathrm{1}} \right)\left({y}−{y}_{\mathrm{2}} \right)} \\ $$$$=\mathrm{2}\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{3}}}\int_{\mathrm{0}} ^{\mathrm{2}−\sqrt{\mathrm{3}}} \:\:\left(\frac{\mathrm{1}}{{y}−{y}_{\mathrm{1}} }−\frac{\mathrm{1}}{{y}−{y}_{\mathrm{2}} }\right){dy} \\ $$$$=\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}\left[{log}\mid\frac{{y}+\mathrm{2}−\sqrt{\mathrm{3}}}{{y}+\mathrm{2}+\sqrt{\mathrm{3}}}\mid\right]_{\mathrm{0}} ^{\mathrm{2}−\sqrt{\mathrm{3}}} \\ $$$$=\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}\left\{{log}\left(\frac{\mathrm{4}−\mathrm{2}\sqrt{\mathrm{3}}}{\mathrm{4}}\right)−{log}\left(\frac{\mathrm{2}−\sqrt{\mathrm{3}}}{\mathrm{2}+\sqrt{\mathrm{3}}}\right)\right\} \\ $$$${I}=−\frac{\mathrm{1}}{\mathrm{2}}+\frac{\sqrt{\mathrm{3}}}{\mathrm{4}}+\frac{\pi}{\mathrm{24}}+\frac{\sqrt{\mathrm{3}}}{\mathrm{4}}\left\{{log}\left(\mathrm{1}−\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\right)−{log}\left(\frac{\mathrm{2}−\sqrt{\mathrm{3}}}{\mathrm{2}+\sqrt{\mathrm{3}}}\right)\right\} \\ $$
