
Question Number 138611 by liberty last updated on 15/Apr/21

$${Given}\:{a}\:{function}\:{f}\:{where}\: \\ $$$${f}\left({x}\right)\geqslant\:\mathrm{0}{for}\:\forall{x}\in\mathbb{R}.\:{If}\:{the}\:{area} \\ $$$${U}\:=\:\left\{\:\left({x},{y}\right)\mid\mathrm{0}\leqslant\mathrm{2}{y}\leqslant{f}\left({x}\right),\:−\mathrm{6}\leqslant{x}\leqslant−\mathrm{2}\right\} \\ $$$${is}\:{u}\:{and}\:{the}\:{area}\:{V}=\left\{\left({x},{y}\right)\mid\mathrm{0}\leqslant{y}\leqslant{f}\left({x}\right),−\mathrm{2}\leqslant{x}\leqslant\mathrm{0}\right\} \\ $$$${is}\:{v}\:{then}\:{what}\:{the}\:{value}\:{of} \\ $$$$\underset{\mathrm{1}} {\overset{\mathrm{2}} {\int}}\:\mathrm{4}{x}\:{f}\left(\mathrm{2}{x}^{\mathrm{2}} −\mathrm{8}\right)\:{dx}\:. \\ $$$$\left({A}\right)\:\mathrm{5}{u}+\mathrm{4}{v}\:\:\:\:\:\left({D}\right)\mathrm{2}{u}+{v} \\ $$$$\left({B}\right)\:\mathrm{4}{u}+\mathrm{3}{v}\:\:\:\:\left({E}\right)\:{u}+{v} \\ $$$$\left({C}\right)\:\mathrm{3}{u}+\mathrm{2}{v} \\ $$
Answered by ajfour last updated on 15/Apr/21
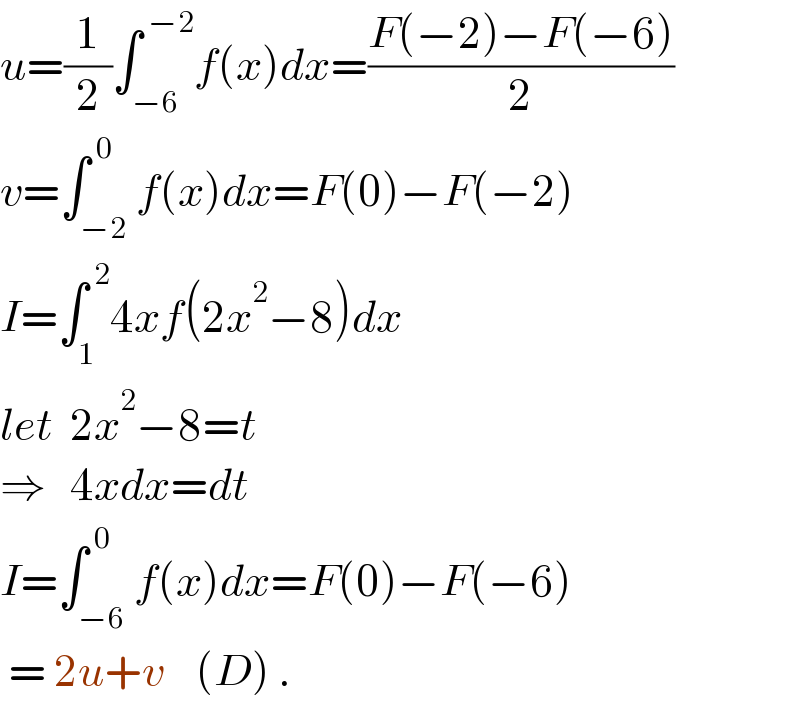
$${u}=\frac{\mathrm{1}}{\mathrm{2}}\int_{−\mathrm{6}} ^{\:−\mathrm{2}} {f}\left({x}\right){dx}=\frac{{F}\left(−\mathrm{2}\right)−{F}\left(−\mathrm{6}\right)}{\mathrm{2}} \\ $$$${v}=\int_{−\mathrm{2}} ^{\:\mathrm{0}} {f}\left({x}\right){dx}={F}\left(\mathrm{0}\right)−{F}\left(−\mathrm{2}\right) \\ $$$${I}=\int_{\mathrm{1}} ^{\:\mathrm{2}} \mathrm{4}{xf}\left(\mathrm{2}{x}^{\mathrm{2}} −\mathrm{8}\right){dx} \\ $$$${let}\:\:\mathrm{2}{x}^{\mathrm{2}} −\mathrm{8}={t} \\ $$$$\Rightarrow\:\:\:\mathrm{4}{xdx}={dt} \\ $$$${I}=\int_{−\mathrm{6}} ^{\:\mathrm{0}} {f}\left({x}\right){dx}={F}\left(\mathrm{0}\right)−{F}\left(−\mathrm{6}\right) \\ $$$$\:=\:\mathrm{2}{u}+{v}\:\:\:\:\left({D}\right)\:. \\ $$
Answered by tepebea last updated on 16/Apr/21

$${mantap}... \\ $$
Commented by ajfour last updated on 16/Apr/21
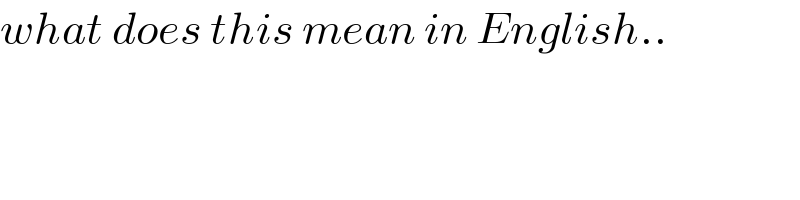
$${what}\:{does}\:{this}\:{mean}\:{in}\:{English}.. \\ $$
Commented by tepebea last updated on 16/Apr/21
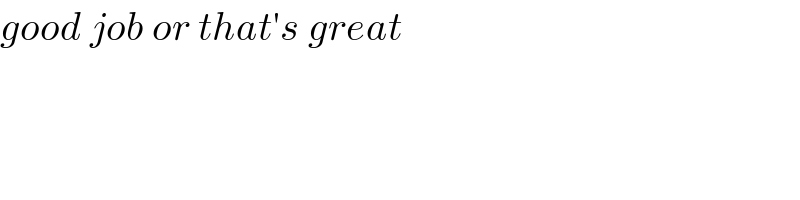
$${good}\:{job}\:{or}\:{that}'{s}\:{great} \\ $$
