
Question Number 138519 by mr W last updated on 14/Apr/21
Commented by mr W last updated on 14/Apr/21
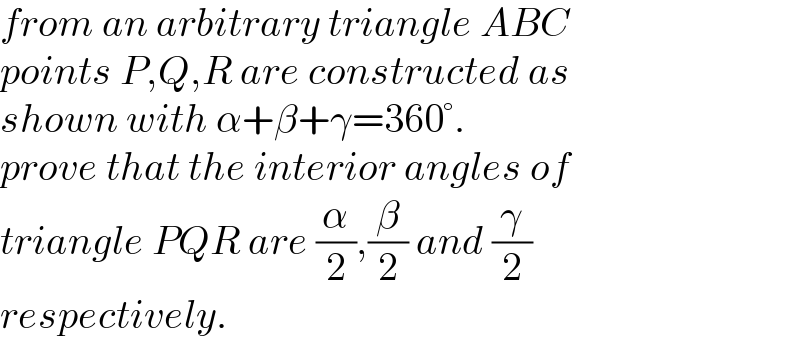
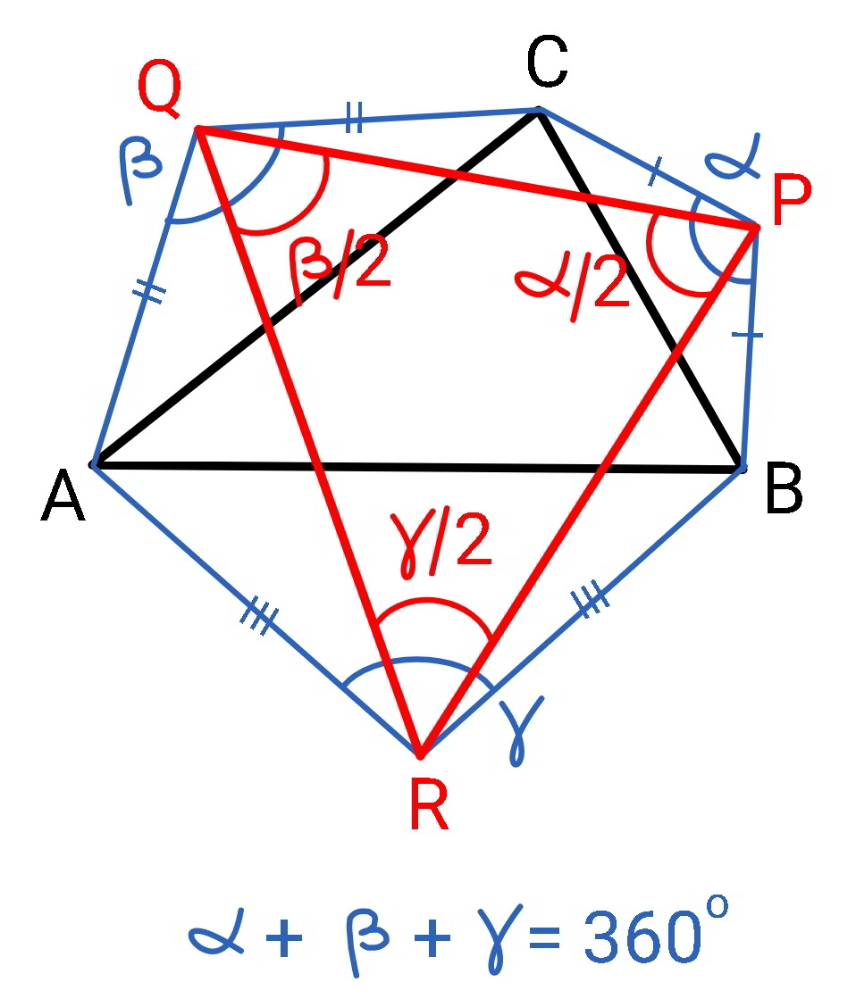
$${from}\:{an}\:{arbitrary}\:{triangle}\:{ABC} \\ $$$${points}\:{P},{Q},{R}\:{are}\:{constructed}\:{as} \\ $$$${shown}\:{with}\:\alpha+\beta+\gamma=\mathrm{360}°. \\ $$$${prove}\:{that}\:{the}\:{interior}\:{angles}\:{of} \\ $$$${triangle}\:{PQR}\:{are}\:\frac{\alpha}{\mathrm{2}},\frac{\beta}{\mathrm{2}}\:{and}\:\frac{\gamma}{\mathrm{2}} \\ $$$${respectively}. \\ $$
Commented by mr W last updated on 16/Apr/21

$${proof}\:{see}\:{Q}\mathrm{138661} \\ $$
