
Question Number 137473 by mr W last updated on 03/Apr/21
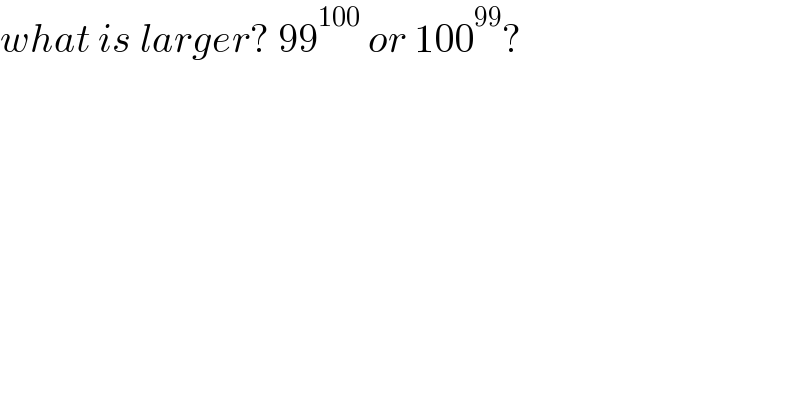
$${what}\:{is}\:{larger}?\:\mathrm{99}^{\mathrm{100}} \:{or}\:\mathrm{100}^{\mathrm{99}} ? \\ $$
Answered by MJS_new last updated on 03/Apr/21
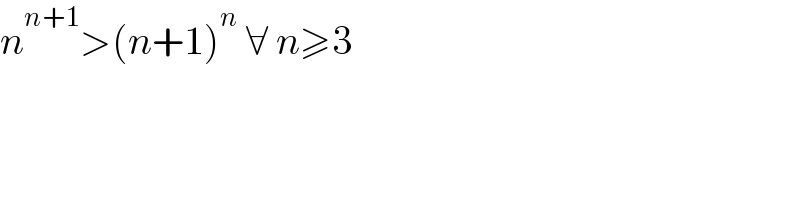
$${n}^{{n}+\mathrm{1}} >\left({n}+\mathrm{1}\right)^{{n}} \:\forall\:{n}\geqslant\mathrm{3} \\ $$
Commented by mr W last updated on 03/Apr/21

$${yes}\:{sir}. \\ $$
Answered by mr W last updated on 03/Apr/21
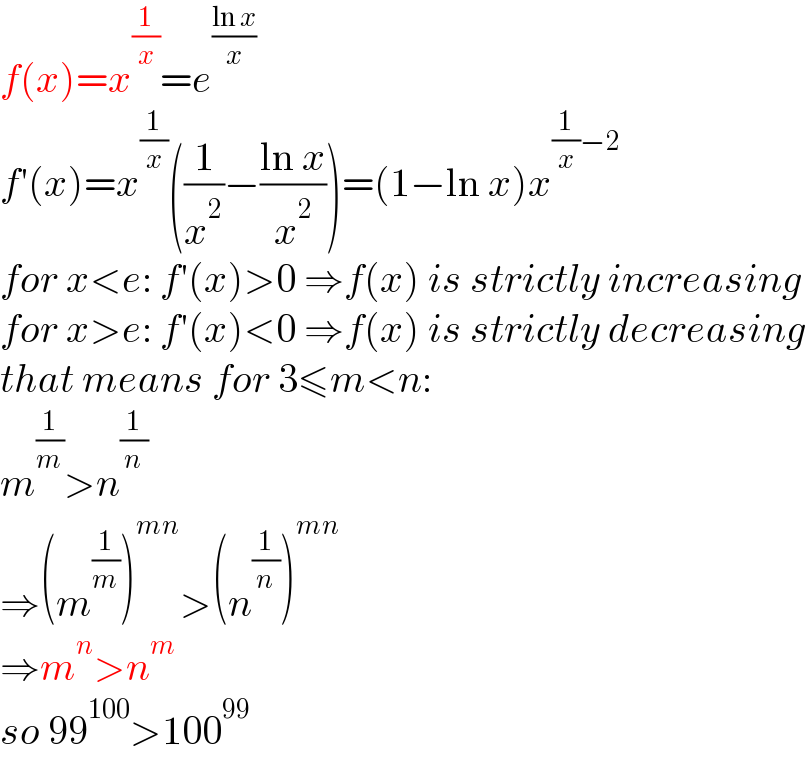
$${f}\left({x}\right)={x}^{\frac{\mathrm{1}}{{x}}} ={e}^{\frac{\mathrm{ln}\:{x}}{{x}}} \\ $$$${f}'\left({x}\right)={x}^{\frac{\mathrm{1}}{{x}}} \left(\frac{\mathrm{1}}{{x}^{\mathrm{2}} }−\frac{\mathrm{ln}\:{x}}{{x}^{\mathrm{2}} }\right)=\left(\mathrm{1}−\mathrm{ln}\:{x}\right){x}^{\frac{\mathrm{1}}{{x}}−\mathrm{2}} \\ $$$${for}\:{x}<{e}:\:{f}'\left({x}\right)>\mathrm{0}\:\Rightarrow{f}\left({x}\right)\:{is}\:{strictly}\:{increasing} \\ $$$${for}\:{x}>{e}:\:{f}'\left({x}\right)<\mathrm{0}\:\Rightarrow{f}\left({x}\right)\:{is}\:{strictly}\:{decreasing} \\ $$$${that}\:{means}\:{for}\:\mathrm{3}\leqslant{m}<{n}: \\ $$$${m}^{\frac{\mathrm{1}}{{m}}} >{n}^{\frac{\mathrm{1}}{{n}}} \\ $$$$\Rightarrow\left({m}^{\frac{\mathrm{1}}{{m}}} \right)^{{mn}} >\left({n}^{\frac{\mathrm{1}}{{n}}} \right)^{{mn}} \\ $$$$\Rightarrow{m}^{{n}} >{n}^{{m}} \\ $$$${so}\:\mathrm{99}^{\mathrm{100}} >\mathrm{100}^{\mathrm{99}} \\ $$
