
Question Number 136243 by Khalmohmmad last updated on 19/Mar/21

$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{sin}{x}−{x}}{\mathrm{tan}{x}−{x}}=? \\ $$
Answered by EDWIN88 last updated on 20/Mar/21
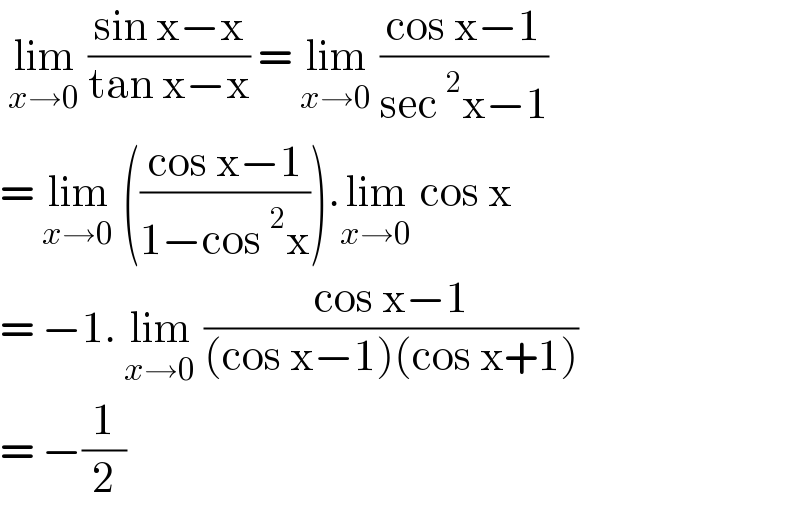
$$\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{sin}\:\mathrm{x}−\mathrm{x}}{\mathrm{tan}\:\mathrm{x}−\mathrm{x}}\:=\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{cos}\:\mathrm{x}−\mathrm{1}}{\mathrm{sec}\:^{\mathrm{2}} \mathrm{x}−\mathrm{1}} \\ $$$$=\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\left(\frac{\mathrm{cos}\:\mathrm{x}−\mathrm{1}}{\mathrm{1}−\mathrm{cos}\:^{\mathrm{2}} \mathrm{x}}\right).\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\mathrm{cos}\:\mathrm{x} \\ $$$$=\:−\mathrm{1}.\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{cos}\:\mathrm{x}−\mathrm{1}}{\left(\mathrm{cos}\:\mathrm{x}−\mathrm{1}\right)\left(\mathrm{cos}\:\mathrm{x}+\mathrm{1}\right)} \\ $$$$=\:−\frac{\mathrm{1}}{\mathrm{2}} \\ $$
Answered by liberty last updated on 20/Mar/21
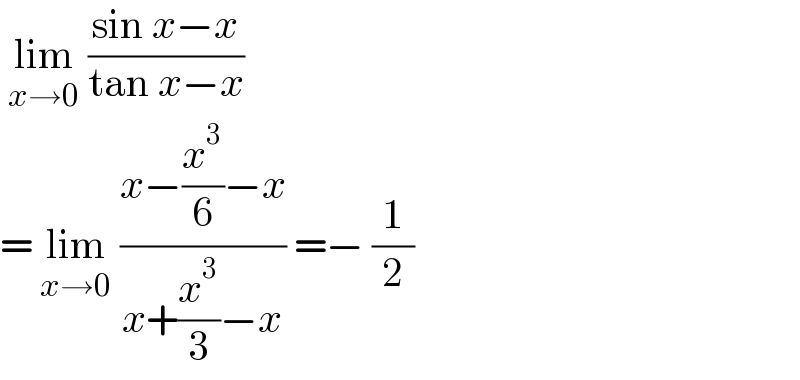
$$\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{sin}\:{x}−{x}}{\mathrm{tan}\:{x}−{x}} \\ $$$$=\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{{x}−\frac{{x}^{\mathrm{3}} }{\mathrm{6}}−{x}}{{x}+\frac{{x}^{\mathrm{3}} }{\mathrm{3}}−{x}}\:=−\:\frac{\mathrm{1}}{\mathrm{2}} \\ $$
