
Question Number 134758 by rs4089 last updated on 07/Mar/21
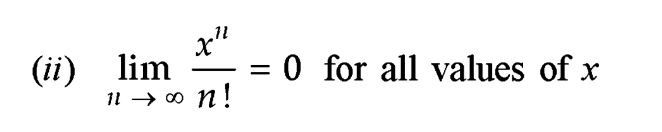
Commented by rs4089 last updated on 07/Mar/21

$${prove}\:{it} \\ $$
Answered by mnjuly1970 last updated on 07/Mar/21
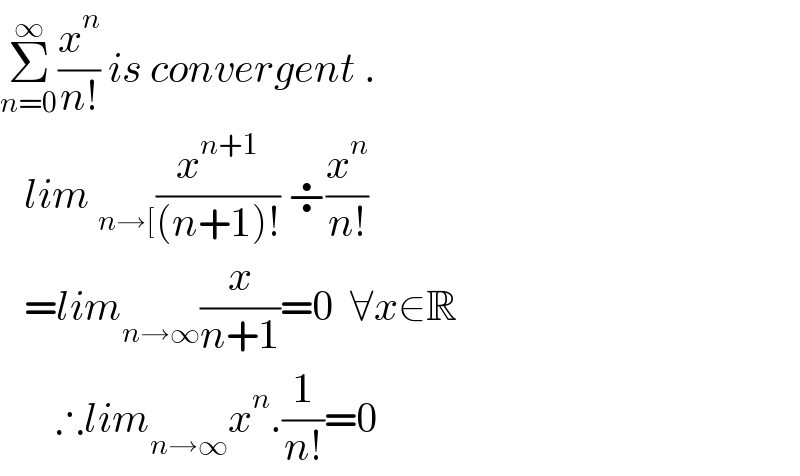
$$\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{{x}^{{n}} }{{n}!}\:{is}\:{convergent}\:. \\ $$$$\:\:\:{lim}\:_{{n}\rightarrow\left[\right.} \frac{{x}^{{n}+\mathrm{1}} }{\left({n}+\mathrm{1}\right)!}\:\boldsymbol{\div}\frac{{x}^{{n}} }{{n}!} \\ $$$$\:\:\:={lim}_{{n}\rightarrow\infty} \frac{{x}}{{n}+\mathrm{1}}=\mathrm{0}\:\:\forall{x}\in\mathbb{R} \\ $$$$\:\:\:\:\:\:\:\therefore{lim}_{{n}\rightarrow\infty} {x}^{{n}} .\frac{\mathrm{1}}{{n}!}=\mathrm{0} \\ $$
