
Question Number 135570 by bemath last updated on 14/Mar/21

$${Geometry} \\ $$ If the area of the triangle formed by the positive x-axis, the normal and the tangent to the circle (x-2) ² +(y-3) ² =25 at the point (5,7) is A, then 24A is equal to______?\\n
Answered by EDWIN88 last updated on 14/Mar/21
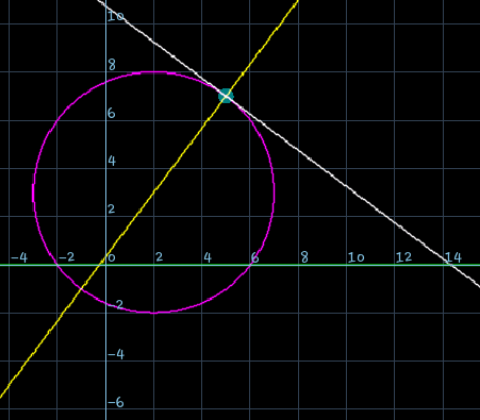
Commented byEDWIN88 last updated on 14/Mar/21
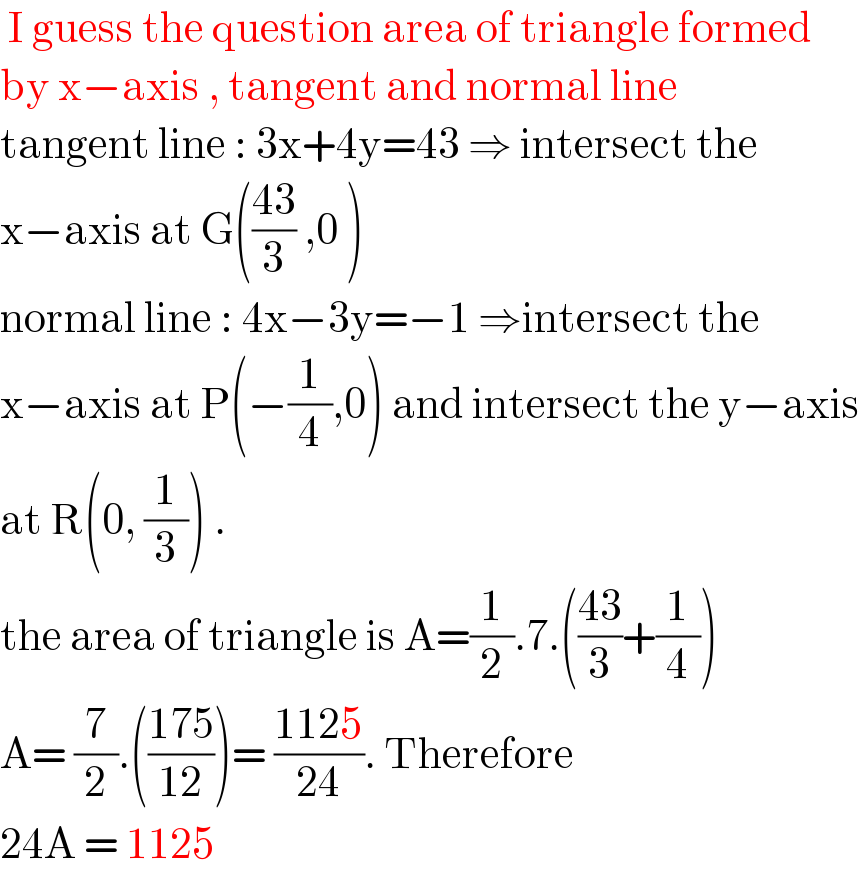
$$\:\mathrm{\color{mathred}{I}}\color{mathred}{\:}\mathrm{\color{mathred}{g}\color{mathred}{u}\color{mathred}{e}\color{mathred}{s}\color{mathred}{s}}\color{mathred}{\:}\mathrm{\color{mathred}{t}\color{mathred}{h}\color{mathred}{e}}\color{mathred}{\:}\mathrm{\color{mathred}{q}\color{mathred}{u}\color{mathred}{e}\color{mathred}{s}\color{mathred}{t}\color{mathred}{i}\color{mathred}{o}\color{mathred}{n}}\color{mathred}{\:}\mathrm{\color{mathred}{a}\color{mathred}{r}\color{mathred}{e}\color{mathred}{a}}\color{mathred}{\:}\mathrm{\color{mathred}{o}\color{mathred}{f}}\color{mathred}{\:}\mathrm{\color{mathred}{t}\color{mathred}{r}\color{mathred}{i}\color{mathred}{a}\color{mathred}{n}\color{mathred}{g}\color{mathred}{l}\color{mathred}{e}}\color{mathred}{\:}\mathrm{\color{mathred}{f}\color{mathred}{o}\color{mathred}{r}\color{mathred}{m}\color{mathred}{e}\color{mathred}{d}} \\ $$ $$\mathrm{\color{mathred}{b}\color{mathred}{y}}\color{mathred}{\:}\mathrm{\color{mathred}{x}}\color{mathred}{−}\mathrm{\color{mathred}{a}\color{mathred}{x}\color{mathred}{i}\color{mathred}{s}}\color{mathred}{\:}\color{mathred}{,}\color{mathred}{\:}\mathrm{\color{mathred}{t}\color{mathred}{a}\color{mathred}{n}\color{mathred}{g}\color{mathred}{e}\color{mathred}{n}\color{mathred}{t}}\color{mathred}{\:}\mathrm{\color{mathred}{a}\color{mathred}{n}\color{mathred}{d}}\color{mathred}{\:}\mathrm{\color{mathred}{n}\color{mathred}{o}\color{mathred}{r}\color{mathred}{m}\color{mathred}{a}\color{mathred}{l}}\color{mathred}{\:}\mathrm{\color{mathred}{l}\color{mathred}{i}\color{mathred}{n}\color{mathred}{e}} \\ $$ $$\mathrm{tangent}\:\mathrm{line}\::\:\mathrm{3x}+\mathrm{4y}=\mathrm{43}\:\Rightarrow\:\mathrm{intersect}\:\mathrm{the} \\ $$ $$\mathrm{x}−\mathrm{axis}\:\mathrm{at}\:\mathrm{G}\left(\frac{\mathrm{43}}{\mathrm{3}}\:,\mathrm{0}\:\right) \\ $$ $$\mathrm{normal}\:\mathrm{line}\::\:\mathrm{4x}−\mathrm{3y}=−\mathrm{1}\:\Rightarrow\mathrm{intersect}\:\mathrm{the} \\ $$ $$\mathrm{x}−\mathrm{axis}\:\mathrm{at}\:\mathrm{P}\left(−\frac{\mathrm{1}}{\mathrm{4}},\mathrm{0}\right)\:\mathrm{and}\:\mathrm{intersect}\:\mathrm{the}\:\mathrm{y}−\mathrm{axis} \\ $$ $$\mathrm{at}\:\mathrm{R}\left(\mathrm{0},\:\frac{\mathrm{1}}{\mathrm{3}}\right)\:. \\ $$ $$\mathrm{the}\:\mathrm{area}\:\mathrm{of}\:\mathrm{triangle}\:\mathrm{is}\:\mathrm{A}=\frac{\mathrm{1}}{\mathrm{2}}.\mathrm{7}.\left(\frac{\mathrm{43}}{\mathrm{3}}+\frac{\mathrm{1}}{\mathrm{4}}\right) \\ $$ $$\mathrm{A}=\:\frac{\mathrm{7}}{\mathrm{2}}.\left(\frac{\mathrm{175}}{\mathrm{12}}\right)=\:\frac{\mathrm{112\color{mathred}{5}}}{\mathrm{24}}.\:\mathrm{Therefore} \\ $$ $$\mathrm{24A}\:=\:\mathrm{\color{mathred}{1}\color{mathred}{1}\color{mathred}{2}\color{mathred}{5}} \\ $$
