
Question Number 132866 by bramlexs22 last updated on 17/Feb/21

$$\mathrm{A}\:\mathrm{certain}\:\mathrm{person}'\mathrm{s}\:\mathrm{blood}\:\mathrm{preassure} \\ $$$$\mathrm{is}\:\mathrm{modelled}\:\mathrm{by}\:\mathrm{the}\:\mathrm{function} \\ $$$$\mathrm{p}\left(\mathrm{t}\right)=\mathrm{115}+\mathrm{25}\:\mathrm{sin}\:\left(\mathrm{160}\pi\mathrm{t}\right) \\ $$$$\mathrm{where}\:\mathrm{p}\left(\mathrm{t}\right)\:\mathrm{is}\:\mathrm{the}\:\mathrm{pressure}\:\mathrm{in}\:\mathrm{mmHg} \\ $$$$\mathrm{at}\:\mathrm{time}\:\mathrm{t}\:\mathrm{measured}\:\mathrm{in}\:\mathrm{minutes}. \\ $$$$\left(\mathrm{a}\right)\:\mathrm{Find}\:\mathrm{the}\:\mathrm{number}\:\mathrm{of}\:\mathrm{heartbeats}\:\mathrm{per}\:\mathrm{minute} \\ $$$$\left(\mathrm{b}\right)\:\mathrm{Find}\:\mathrm{the}\:\mathrm{blood}\:\mathrm{preassure} \\ $$$$\mathrm{reading}.\:\mathrm{How}\:\mathrm{does}\:\mathrm{this}\:\mathrm{compare}\: \\ $$$$\mathrm{to}\:\mathrm{normal}\:\mathrm{blood}\:\mathrm{preassure}? \\ $$
Answered by TheSupreme last updated on 17/Feb/21
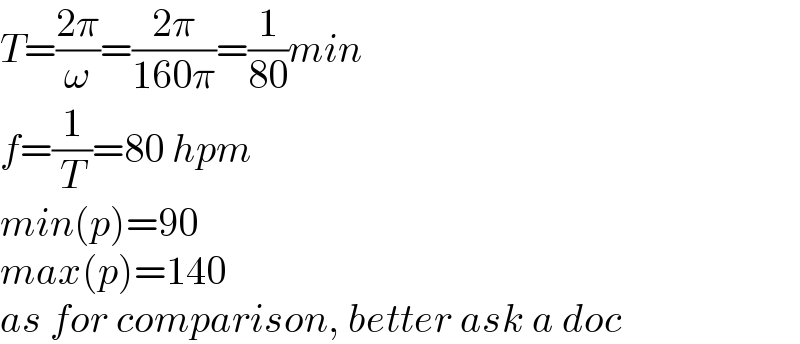
$${T}=\frac{\mathrm{2}\pi}{\omega}=\frac{\mathrm{2}\pi}{\mathrm{160}\pi}=\frac{\mathrm{1}}{\mathrm{80}}{min} \\ $$$${f}=\frac{\mathrm{1}}{{T}}=\mathrm{80}\:{hpm} \\ $$$${min}\left({p}\right)=\mathrm{90} \\ $$$${max}\left({p}\right)=\mathrm{140} \\ $$$${as}\:{for}\:{comparison},\:{better}\:{ask}\:{a}\:{doc} \\ $$
Commented by bramlexs22 last updated on 17/Feb/21

$$\mathrm{love}\:\mathrm{doctor} \\ $$
