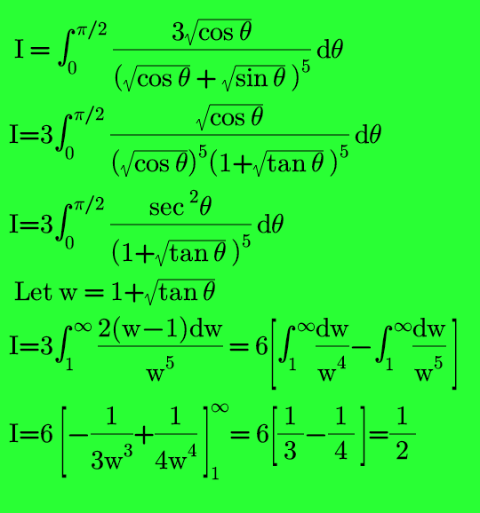Question Number 129839 by liberty last updated on 20/Jan/21
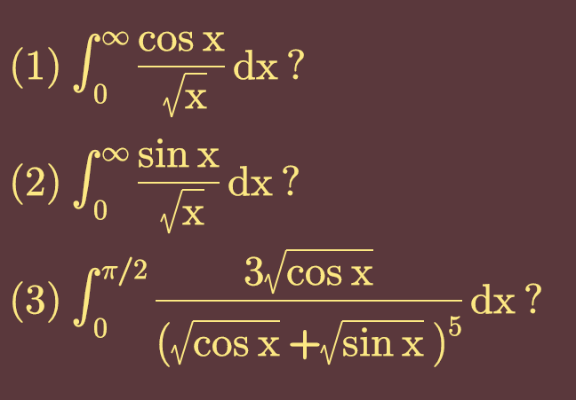
Answered by EDWIN88 last updated on 20/Jan/21
![J=∫_( 0) ^( π/2) ((3(√(cos x)))/(((√(cos x)) +(√(sin x)) )^5 )) dx let x=(π/2)−t ⇒J=∫_(π/2) ^( 0) ((3(√(sin t)))/(((√(sin t)) +(√(cos t)))^5 ))(−dt) or J = ∫_0 ^(π/2) ((3(√(sin x)))/(((√(sin x)) +(√(cos x)) )^5 )) dx we get 2J = ∫_0 ^( π/2) ((3((√(sin x)) +(√(cos x)) ))/(((√(sin x)) +(√(cos x)) )^5 )) dx 2J = ∫_0 ^( π/2) ((3 dx)/(((√(cos x)) (1+(√(tan x)) ))^4 )) 2J= 3∫_0 ^( π/2) ((sec^2 x)/((1+(√(tan x)))^4 )) dx 2J = 3∫_0 ^( ∞) (dj/((1+(√j) )^4 )) 2J=3∫_1 ^( ∞) (z^(−3) −z^(−4) )dz ; with z=1+(√j) J=(3/2)lim_(q→∞) [ −(z^(−2) /2)+(z^(−3) /3) ]_1 ^q = (1/2)](Q129840.png)
$$\mathrm{J}=\int_{\:\mathrm{0}} ^{\:\pi/\mathrm{2}} \:\frac{\mathrm{3}\sqrt{\mathrm{cos}\:\mathrm{x}}}{\left(\sqrt{\mathrm{cos}\:\mathrm{x}}\:+\sqrt{\mathrm{sin}\:\mathrm{x}}\:\right)^{\mathrm{5}} }\:\mathrm{dx}\: \\ $$$$\:\mathrm{let}\:\mathrm{x}=\frac{\pi}{\mathrm{2}}−\mathrm{t}\:\Rightarrow\mathrm{J}=\int_{\frac{\pi}{\mathrm{2}}} ^{\:\mathrm{0}} \:\frac{\mathrm{3}\sqrt{\mathrm{sin}\:\mathrm{t}}}{\left(\sqrt{\mathrm{sin}\:\mathrm{t}}\:+\sqrt{\mathrm{cos}\:\mathrm{t}}\right)^{\mathrm{5}} }\left(−\mathrm{dt}\right) \\ $$$$\:\mathrm{or}\:\mathrm{J}\:=\:\int_{\mathrm{0}} ^{\pi/\mathrm{2}} \:\frac{\mathrm{3}\sqrt{\mathrm{sin}\:\mathrm{x}}}{\left(\sqrt{\mathrm{sin}\:\mathrm{x}}\:+\sqrt{\mathrm{cos}\:\mathrm{x}}\:\right)^{\mathrm{5}} }\:\mathrm{dx} \\ $$$$\mathrm{we}\:\mathrm{get}\:\mathrm{2J}\:=\:\int_{\mathrm{0}} ^{\:\pi/\mathrm{2}} \:\:\frac{\mathrm{3}\left(\sqrt{\mathrm{sin}\:\mathrm{x}}\:+\sqrt{\mathrm{cos}\:\mathrm{x}}\:\right)}{\left(\sqrt{\mathrm{sin}\:\mathrm{x}}\:+\sqrt{\mathrm{cos}\:\mathrm{x}}\:\right)^{\mathrm{5}} }\:\mathrm{dx} \\ $$$$\mathrm{2J}\:=\:\int_{\mathrm{0}} ^{\:\pi/\mathrm{2}} \:\frac{\mathrm{3}\:\mathrm{dx}}{\left(\sqrt{\mathrm{cos}\:\mathrm{x}}\:\left(\mathrm{1}+\sqrt{\mathrm{tan}\:\mathrm{x}}\:\right)\right)^{\mathrm{4}} } \\ $$$$\mathrm{2J}=\:\mathrm{3}\int_{\mathrm{0}} ^{\:\pi/\mathrm{2}} \:\frac{\mathrm{sec}\:^{\mathrm{2}} \mathrm{x}}{\left(\mathrm{1}+\sqrt{\mathrm{tan}\:\mathrm{x}}\right)^{\mathrm{4}} }\:\mathrm{dx} \\ $$$$\mathrm{2J}\:=\:\mathrm{3}\int_{\mathrm{0}} ^{\:\infty} \:\frac{\mathrm{dj}}{\left(\mathrm{1}+\sqrt{\mathrm{j}}\:\right)^{\mathrm{4}} } \\ $$$$\mathrm{2J}=\mathrm{3}\int_{\mathrm{1}} ^{\:\infty} \left(\mathrm{z}^{−\mathrm{3}} −\mathrm{z}^{−\mathrm{4}} \right)\mathrm{dz}\:;\:\mathrm{with}\:\mathrm{z}=\mathrm{1}+\sqrt{\mathrm{j}} \\ $$$$\mathrm{J}=\frac{\mathrm{3}}{\mathrm{2}}\underset{\mathrm{q}\rightarrow\infty} {\mathrm{lim}}\:\left[\:−\frac{\mathrm{z}^{−\mathrm{2}} }{\mathrm{2}}+\frac{\mathrm{z}^{−\mathrm{3}} }{\mathrm{3}}\:\right]_{\mathrm{1}} ^{\mathrm{q}} =\:\frac{\mathrm{1}}{\mathrm{2}} \\ $$$$ \\ $$
Answered by Lordose last updated on 20/Jan/21

$$\mathrm{1}.\:\Omega\:=\:\int_{\mathrm{0}} ^{\:\infty} \frac{\mathrm{cos}\:\mathrm{x}}{\:\sqrt{\mathrm{x}}}\mathrm{dx}\:=\:\mathrm{Re}\int_{\mathrm{0}} ^{\:\infty} \mathrm{x}^{−\frac{\mathrm{1}}{\mathrm{2}}} \mathrm{e}^{−\mathrm{ix}} \mathrm{dx}\:\overset{\mathrm{u}=\mathrm{ix}} {=}\mathrm{Re}\left(\frac{\mathrm{1}}{\:\sqrt{\mathrm{i}}}\int_{\mathrm{0}} ^{\:\infty} \mathrm{u}^{\frac{\mathrm{1}}{\mathrm{2}}−\mathrm{1}} \mathrm{e}^{−\mathrm{u}} \mathrm{du}\right)\:\:\:\:\:\:\:\:\:\:\:\: \\ $$$$\:\:\:\:\:\Omega\:=\:\mathrm{Re}\left(\frac{\mathrm{1}}{\:\sqrt{\mathrm{i}}}\boldsymbol{\Gamma}\left(\frac{\mathrm{1}}{\mathrm{2}}\right)\right)\:=\:\mathrm{Re}\left(\sqrt{\pi}\left(\frac{\mathrm{1}−\mathrm{i}}{\:\sqrt{\mathrm{2}}}\right)\right)\:=\:\sqrt{\frac{\pi}{\mathrm{2}}} \\ $$$$ \\ $$
Answered by Lordose last updated on 20/Jan/21
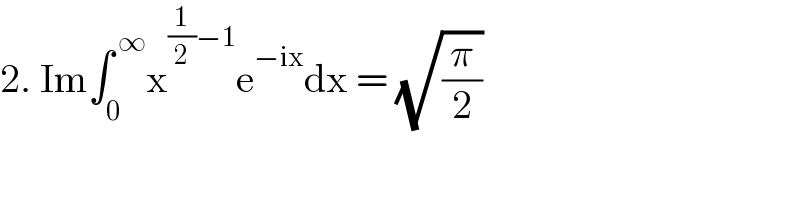
$$\mathrm{2}.\:\mathrm{Im}\int_{\mathrm{0}} ^{\:\infty} \mathrm{x}^{\frac{\mathrm{1}}{\mathrm{2}}−\mathrm{1}} \mathrm{e}^{−\mathrm{ix}} \mathrm{dx}\:=\:\sqrt{\frac{\pi}{\mathrm{2}}} \\ $$
Answered by Olaf last updated on 20/Jan/21
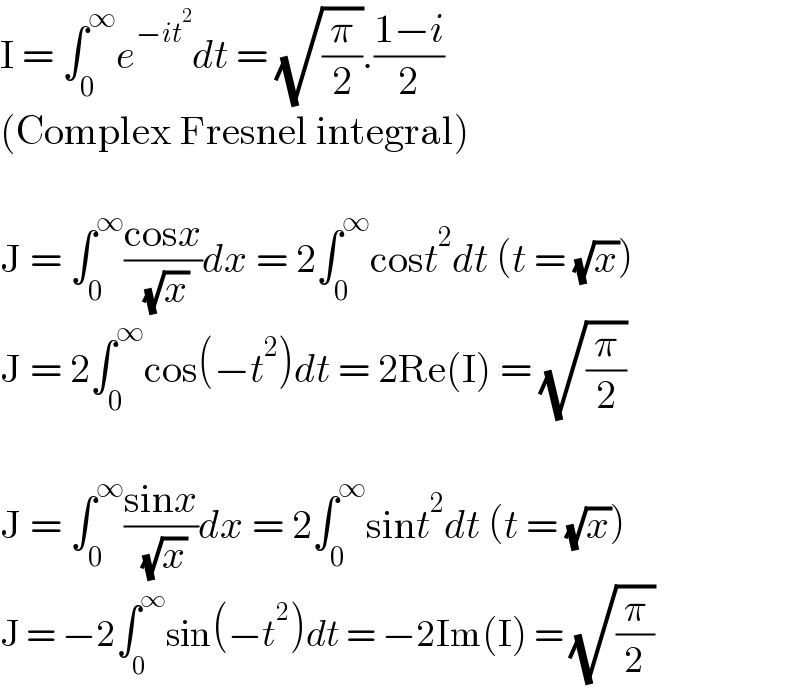
$$\mathrm{I}\:=\:\int_{\mathrm{0}} ^{\infty} {e}^{−{it}^{\mathrm{2}} } {dt}\:=\:\sqrt{\frac{\pi}{\mathrm{2}}}.\frac{\mathrm{1}−{i}}{\mathrm{2}} \\ $$$$\left(\mathrm{Complex}\:\mathrm{Fresnel}\:\mathrm{integral}\right) \\ $$$$ \\ $$$$\mathrm{J}\:=\:\int_{\mathrm{0}} ^{\infty} \frac{\mathrm{cos}{x}}{\:\sqrt{{x}}}{dx}\:=\:\mathrm{2}\int_{\mathrm{0}} ^{\infty} \mathrm{cos}{t}^{\mathrm{2}} {dt}\:\left({t}\:=\:\sqrt{{x}}\right) \\ $$$$\mathrm{J}\:=\:\mathrm{2}\int_{\mathrm{0}} ^{\infty} \mathrm{cos}\left(−{t}^{\mathrm{2}} \right){dt}\:=\:\mathrm{2Re}\left(\mathrm{I}\right)\:=\:\sqrt{\frac{\pi}{\mathrm{2}}} \\ $$$$ \\ $$$$\mathrm{J}\:=\:\int_{\mathrm{0}} ^{\infty} \frac{\mathrm{sin}{x}}{\:\sqrt{{x}}}{dx}\:=\:\mathrm{2}\int_{\mathrm{0}} ^{\infty} \mathrm{sin}{t}^{\mathrm{2}} {dt}\:\left({t}\:=\:\sqrt{{x}}\right) \\ $$$$\mathrm{J}\:=\:−\mathrm{2}\int_{\mathrm{0}} ^{\infty} \mathrm{sin}\left(−{t}^{\mathrm{2}} \right){dt}\:=\:−\mathrm{2Im}\left(\mathrm{I}\right)\:=\:\sqrt{\frac{\pi}{\mathrm{2}}} \\ $$
Answered by EDWIN88 last updated on 20/Jan/21
![one approach to solving this would be to use the Gaussian Integral ∫_0 ^( ∞) e^(−t^2 ) dt = ((√π)/2) let t =y(√x) where x is constant (2/( (√π)))∫_0 ^( ∞) e^(−xy^2 ) dy = (1/( (√x))) I=∫_0 ^( ∞) cos x (2/( (√π))) ∫_0 ^( ∞) e^(−xy^2 ) dydx = (2/( (√π))) ∫_0 ^∞ ∫_0 ^( ∞) e^(−xy^2 ) cos x dx dy = (2/( (√π))) ∫_0 ^( ∞) L(cos t)∣_(s=y^2 ) dy = (2/( (√π))) ∫_0 ^( ∞) (y^2 /(y^4 +1)) dy similarly to J=∫_0 ^( ∞) ((sin x)/( (√x))) dx = (2/( (√π))) ∫_0 ^( ∞) (dy/(y^4 +1)) I=J ⇒2I=(2/( (√π))) ∫_0 ^( ∞) ((y^2 +1)/(y^4 +1)) dy 2I= (2/( (√π))) ∫_(−∞) ^( ∞) (1/(u^2 +2)) du [ with u = y−(1/y) ] 2I=(2/( (√π))).(1/( (√2))) (arctan ((u/( (√2)))) )∣_(−∞) ^∞ I= (√(π/2)) → { ((∫_0 ^∞ ((cos x)/( (√x))) dx = (√(π/2)))),((∫_0 ^( ∞) ((sin x)/( (√x))) dx = (√(π/2)))) :}](Q129850.png)
$$\mathrm{one}\:\mathrm{approach}\:\mathrm{to}\:\mathrm{solving}\:\mathrm{this}\:\mathrm{would}\:\mathrm{be}\:\mathrm{to}\:\mathrm{use} \\ $$$$\mathrm{the}\:\mathrm{Gaussian}\:\mathrm{Integral}\:\int_{\mathrm{0}} ^{\:\infty} \mathrm{e}^{−\mathrm{t}^{\mathrm{2}} } \:\mathrm{dt}\:=\:\frac{\sqrt{\pi}}{\mathrm{2}} \\ $$$$\mathrm{let}\:\mathrm{t}\:=\mathrm{y}\sqrt{\mathrm{x}}\:\mathrm{where}\:\mathrm{x}\:\mathrm{is}\:\mathrm{constant}\: \\ $$$$\:\frac{\mathrm{2}}{\:\sqrt{\pi}}\int_{\mathrm{0}} ^{\:\infty} \mathrm{e}^{−\mathrm{xy}^{\mathrm{2}} } \:\mathrm{dy}\:=\:\frac{\mathrm{1}}{\:\sqrt{\mathrm{x}}}\: \\ $$$$\mathrm{I}=\int_{\mathrm{0}} ^{\:\infty} \mathrm{cos}\:\mathrm{x}\:\frac{\mathrm{2}}{\:\sqrt{\pi}}\:\int_{\mathrm{0}} ^{\:\infty} \mathrm{e}^{−\mathrm{xy}^{\mathrm{2}} } \:\mathrm{dydx} \\ $$$$\:\:=\:\frac{\mathrm{2}}{\:\sqrt{\pi}}\:\int_{\mathrm{0}} ^{\infty} \int_{\mathrm{0}} ^{\:\infty} \mathrm{e}^{−\mathrm{xy}^{\mathrm{2}} } \mathrm{cos}\:\mathrm{x}\:\mathrm{dx}\:\mathrm{dy} \\ $$$$\:\:=\:\frac{\mathrm{2}}{\:\sqrt{\pi}}\:\int_{\mathrm{0}} ^{\:\infty} \:\mathcal{L}\left(\mathrm{cos}\:\mathrm{t}\right)\mid_{\mathrm{s}=\mathrm{y}^{\mathrm{2}} } \:\mathrm{dy} \\ $$$$\:\:=\:\frac{\mathrm{2}}{\:\sqrt{\pi}}\:\int_{\mathrm{0}} ^{\:\infty} \frac{\mathrm{y}^{\mathrm{2}} }{\mathrm{y}^{\mathrm{4}} +\mathrm{1}}\:\mathrm{dy}\: \\ $$$$\mathrm{similarly}\:\mathrm{to}\:\mathrm{J}=\int_{\mathrm{0}} ^{\:\infty} \frac{\mathrm{sin}\:\mathrm{x}}{\:\sqrt{\mathrm{x}}}\:\mathrm{dx}\:=\:\frac{\mathrm{2}}{\:\sqrt{\pi}}\:\int_{\mathrm{0}} ^{\:\infty} \:\frac{\mathrm{dy}}{\mathrm{y}^{\mathrm{4}} +\mathrm{1}} \\ $$$$\mathrm{I}=\mathrm{J}\:\Rightarrow\mathrm{2I}=\frac{\mathrm{2}}{\:\sqrt{\pi}}\:\int_{\mathrm{0}} ^{\:\infty} \:\frac{\mathrm{y}^{\mathrm{2}} +\mathrm{1}}{\mathrm{y}^{\mathrm{4}} +\mathrm{1}}\:\mathrm{dy} \\ $$$$\mathrm{2I}=\:\frac{\mathrm{2}}{\:\sqrt{\pi}}\:\int_{−\infty} ^{\:\infty} \:\frac{\mathrm{1}}{\mathrm{u}^{\mathrm{2}} +\mathrm{2}}\:\mathrm{du}\:\left[\:\mathrm{with}\:\mathrm{u}\:=\:\mathrm{y}−\frac{\mathrm{1}}{\mathrm{y}}\:\right] \\ $$$$\mathrm{2I}=\frac{\mathrm{2}}{\:\sqrt{\pi}}.\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\:\left(\mathrm{arctan}\:\left(\frac{\mathrm{u}}{\:\sqrt{\mathrm{2}}}\right)\:\right)\mid_{−\infty} ^{\infty} \\ $$$$\mathrm{I}=\:\sqrt{\frac{\pi}{\mathrm{2}}}\:\rightarrow\begin{cases}{\int_{\mathrm{0}} ^{\infty} \:\frac{\mathrm{cos}\:\mathrm{x}}{\:\sqrt{\mathrm{x}}}\:\mathrm{dx}\:=\:\sqrt{\frac{\pi}{\mathrm{2}}}}\\{\int_{\mathrm{0}} ^{\:\infty} \:\frac{\mathrm{sin}\:\mathrm{x}}{\:\sqrt{\mathrm{x}}}\:\mathrm{dx}\:=\:\sqrt{\frac{\pi}{\mathrm{2}}}}\end{cases}\: \\ $$
Answered by Bird last updated on 20/Jan/21
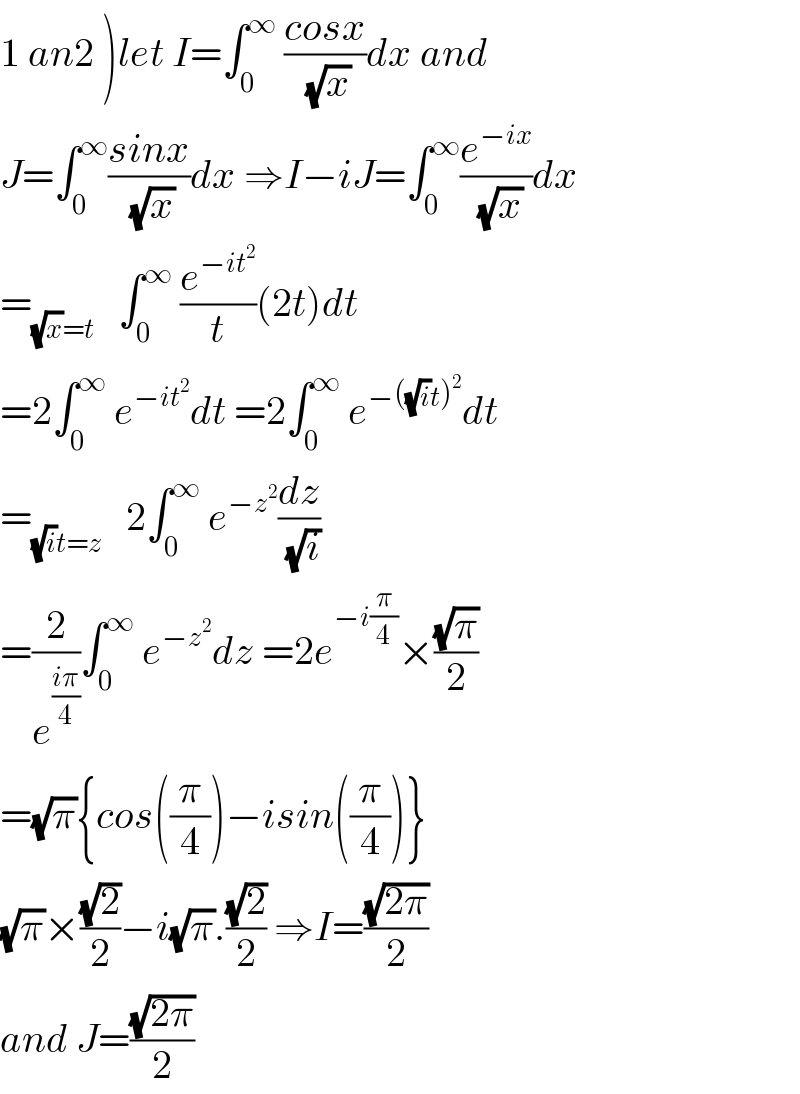
$$\left.\mathrm{1}\:{an}\mathrm{2}\:\right){let}\:{I}=\int_{\mathrm{0}} ^{\infty} \:\frac{{cosx}}{\:\sqrt{{x}}}{dx}\:{and} \\ $$$${J}=\int_{\mathrm{0}} ^{\infty} \frac{{sinx}}{\:\sqrt{{x}}}{dx}\:\Rightarrow{I}−{iJ}=\int_{\mathrm{0}} ^{\infty} \frac{{e}^{−{ix}} }{\:\sqrt{{x}}}{dx} \\ $$$$=_{\sqrt{{x}}={t}} \:\:\:\int_{\mathrm{0}} ^{\infty} \:\frac{{e}^{−{it}^{\mathrm{2}} } }{{t}}\left(\mathrm{2}{t}\right){dt} \\ $$$$=\mathrm{2}\int_{\mathrm{0}} ^{\infty} \:{e}^{−{it}^{\mathrm{2}} } {dt}\:=\mathrm{2}\int_{\mathrm{0}} ^{\infty} \:{e}^{−\left(\sqrt{{i}}{t}\right)^{\mathrm{2}} } {dt} \\ $$$$=_{\sqrt{{i}}{t}={z}} \:\:\:\mathrm{2}\int_{\mathrm{0}} ^{\infty} \:{e}^{−{z}^{\mathrm{2}} } \frac{{dz}}{\:\sqrt{{i}}} \\ $$$$=\frac{\mathrm{2}}{{e}^{\frac{{i}\pi}{\mathrm{4}}} }\int_{\mathrm{0}} ^{\infty} \:{e}^{−{z}^{\mathrm{2}} } {dz}\:=\mathrm{2}{e}^{−{i}\frac{\pi}{\mathrm{4}}} ×\frac{\sqrt{\pi}}{\mathrm{2}} \\ $$$$=\sqrt{\pi}\left\{{cos}\left(\frac{\pi}{\mathrm{4}}\right)−{isin}\left(\frac{\pi}{\mathrm{4}}\right)\right\} \\ $$$$\sqrt{\pi}×\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}−{i}\sqrt{\pi}.\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}\:\Rightarrow{I}=\frac{\sqrt{\mathrm{2}\pi}}{\mathrm{2}} \\ $$$${and}\:{J}=\frac{\sqrt{\mathrm{2}\pi}}{\mathrm{2}} \\ $$
Answered by bemath last updated on 21/Jan/21