
Question Number 128821 by benjo_mathlover last updated on 10/Jan/21

$$\:\mathrm{Nice}\:\mathrm{limit}\:! \\ $$ $$\:\mathrm{For}\:−\mathrm{1}<{a}\:<\mathrm{1}\:\mathrm{the}\:\mathrm{value}\:\mathrm{of}\:\underset{{x}\rightarrow−{a}} {\mathrm{lim}}\:\frac{\left({x}^{\mathrm{2}} +\mathrm{2}{ax}+{a}^{\mathrm{2}} \right)\left({x}^{\mathrm{2}} +{ax}+{a}^{\mathrm{2}} \right)}{\left(\sqrt{\mathrm{1}−{x}^{\mathrm{2}} }−\sqrt{\mathrm{1}−{a}^{\mathrm{2}} }\:\right)^{\mathrm{2}} }\:=? \\ $$
Answered by liberty last updated on 10/Jan/21
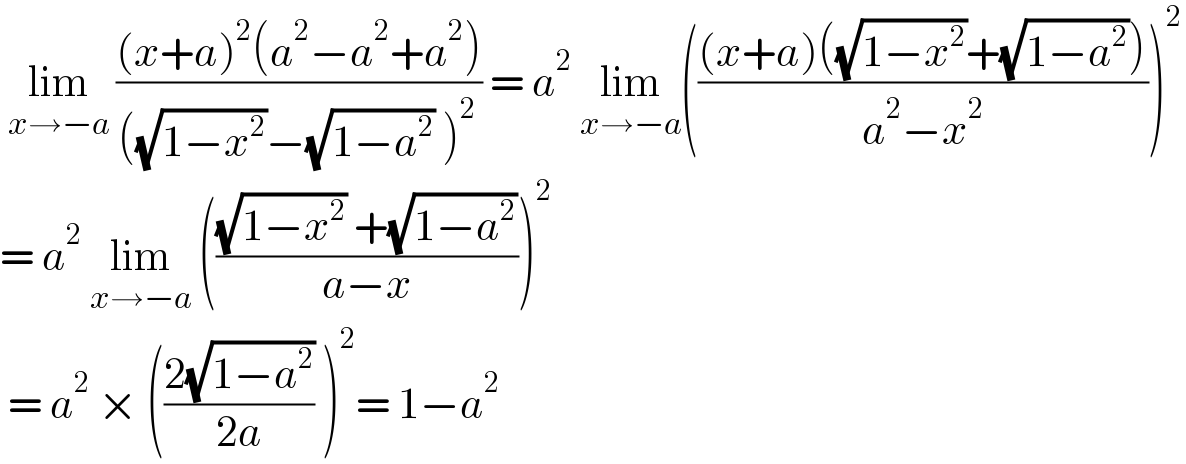
$$\:\underset{{x}\rightarrow−{a}} {\mathrm{lim}}\:\frac{\left({x}+{a}\right)^{\mathrm{2}} \left({a}^{\mathrm{2}} −{a}^{\mathrm{2}} +{a}^{\mathrm{2}} \right)}{\left(\sqrt{\mathrm{1}−{x}^{\mathrm{2}} }−\sqrt{\mathrm{1}−{a}^{\mathrm{2}} }\:\right)^{\mathrm{2}} }\:=\:{a}^{\mathrm{2}} \:\underset{{x}\rightarrow−{a}} {\mathrm{lim}}\left(\frac{\left({x}+{a}\right)\left(\sqrt{\mathrm{1}−{x}^{\mathrm{2}} }+\sqrt{\mathrm{1}−{a}^{\mathrm{2}} }\right)}{{a}^{\mathrm{2}} −{x}^{\mathrm{2}} }\right)^{\mathrm{2}} \\ $$ $$=\:{a}^{\mathrm{2}} \:\underset{{x}\rightarrow−{a}} {\mathrm{lim}}\:\left(\frac{\sqrt{\mathrm{1}−{x}^{\mathrm{2}} }\:+\sqrt{\mathrm{1}−{a}^{\mathrm{2}} }}{{a}−{x}}\right)^{\mathrm{2}} \\ $$ $$\:=\:{a}^{\mathrm{2}} \:×\:\left(\frac{\mathrm{2}\sqrt{\mathrm{1}−{a}^{\mathrm{2}} }}{\mathrm{2}{a}}\:\right)^{\mathrm{2}} =\:\mathrm{1}−{a}^{\mathrm{2}} \\ $$
