
Question Number 128509 by benjo_mathlover last updated on 08/Jan/21
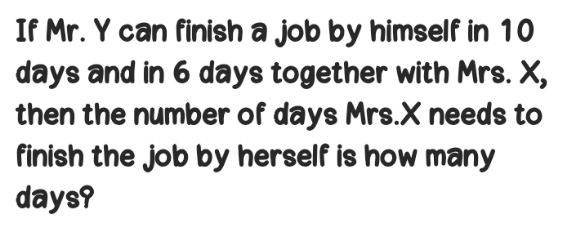
Answered by liberty last updated on 08/Jan/21

$$\left(\Leftrightarrow\right)\:\frac{\mathrm{W}}{\mathrm{y}+\mathrm{x}}\:=\:\mathrm{6}\:\mathrm{days}\:\mathrm{where}\:\frac{\mathrm{W}}{\mathrm{y}}=\mathrm{10}\:\mathrm{days}\: \\ $$$$\mathrm{so}\:\mathrm{we}\:\mathrm{find}\:\frac{\mathrm{W}}{\frac{\mathrm{W}}{\mathrm{10}}+\mathrm{x}}=\mathrm{6}\:\Rightarrow\mathrm{W}=\frac{\mathrm{6W}}{\mathrm{10}}+\mathrm{6x} \\ $$$$\Rightarrow\mathrm{x}\:=\:\frac{\mathrm{4W}}{\mathrm{60}}\:=\:\frac{\mathrm{W}}{\mathrm{15}}\:,\:\mathrm{it}\:\mathrm{follows}\:\mathrm{that}\:\mathrm{Mrs}\:\mathrm{x}\:\mathrm{can} \\ $$$$\mathrm{finish}\:\mathrm{the}\:\mathrm{job}\:\mathrm{by}\:\mathrm{herself}\:\mathrm{in}\:\mathrm{15}\:\mathrm{days} \\ $$
Commented by mr W last updated on 08/Jan/21

$${in}\:{reality}\:{Mr}.\:{Y}\:{can}\:{finish}\:{the}\:{job} \\ $$$${by}\:{himself}\:{in}\:\mathrm{10}\:{days}\:{and}\:{Mrs}.\:{X}\:{can} \\ $$$${finish}\:{the}\:{job}\:{in}\:\mathrm{15}\:{days}\:{by}\:{herself}. \\ $$$${but}\:{Mr}.\:{Y}\:{and}\:{Mrs}.\:{X}\:{together}\:{can} \\ $$$${finish}\:{in},\:{i}\:{think}\:{at}\:{least}\:\mathrm{100}\:{days}, \\ $$$${never}\:{in}\:\mathrm{6}\:{days}. \\ $$$${the}\:{teacher}\:{who}\:{puts}\:{this}\:{question}\:{is} \\ $$$${a}\:{good}\:{mathematician},\:{but}\:{not} \\ $$$${a}\:{keen}\:{observer}\:{of}\:{human}\:{nature}. \\ $$
Commented by mr W last updated on 08/Jan/21

$${or}\:{the}\:{lady}\:{is}\:{not}\:{attractive}\:{and}\:{they} \\ $$$${finish}\:{the}\:{job}\:{together}\:{only}\:{in}\:\mathrm{4}\:{days}. \\ $$
Commented by MJS_new last updated on 08/Jan/21

$$\mathrm{yes}\:\mathrm{indeed}...\:\mathrm{similar}\:\mathrm{to}\:\mathrm{my}\:\mathrm{old}\:\mathrm{example}\:\mathrm{with} \\ $$$$\mathrm{the}\:\mathrm{3}\:\mathrm{construction}\:\mathrm{workers}\:{A},\:{B}\:\mathrm{and}\:{C} \\ $$$$\mathrm{a}\:\mathrm{job}\:{could}\:\mathrm{be}\:\mathrm{finished}\:\mathrm{by} \\ $$$${A}\:\mathrm{alone}\:\mathrm{in}\:\mathrm{10}\:\mathrm{hours} \\ $$$${B}\:\mathrm{alone}\:\mathrm{in}\:\mathrm{12}\:\mathrm{hours} \\ $$$${C}\:\mathrm{alone}\:\mathrm{in}\:\mathrm{15}\:\mathrm{hours} \\ $$$$\mathrm{how}\:\mathrm{long}\:\mathrm{will}\:\mathrm{it}\:\mathrm{take}\:\mathrm{if}\:\mathrm{they}\:\mathrm{work}\:\mathrm{together}? \\ $$$$\mathrm{answer}:\:\mathrm{at}\:\mathrm{least}\:\mathrm{1}\:\mathrm{week}.\:{A}\:\mathrm{starts}\:\mathrm{to}\:\mathrm{work}. \\ $$$${C}\:\mathrm{says}\:\mathrm{he}'\mathrm{d}\:\mathrm{be}\:\mathrm{back}\:\mathrm{in}\:\mathrm{a}\:\mathrm{minute}\:\mathrm{and}\:\mathrm{leaves}. \\ $$$${B}\:\mathrm{starts}\:\mathrm{to}\:\mathrm{complain}\:\mathrm{about}\:{A}'\mathrm{s}\:\mathrm{work}\:\mathrm{and} \\ $$$$\mathrm{makes}\:\mathrm{lots}\:\mathrm{of}\:\mathrm{good}\:\mathrm{appointments}.\:\mathrm{At}\:\mathrm{this} \\ $$$$\mathrm{moment}\:{C}\:\mathrm{comes}\:\mathrm{back}\:\mathrm{with}\:\mathrm{6}\:\mathrm{beer}\:\mathrm{cans}... \\ $$
Commented by liberty last updated on 08/Jan/21
If we look at human nature, yes we have to go back to God, because it could be Mr. Y and Mrs. X finish it together within 2 days? Why not? There are miracles of God that work. I believe it.
Commented by mr W last updated on 08/Jan/21
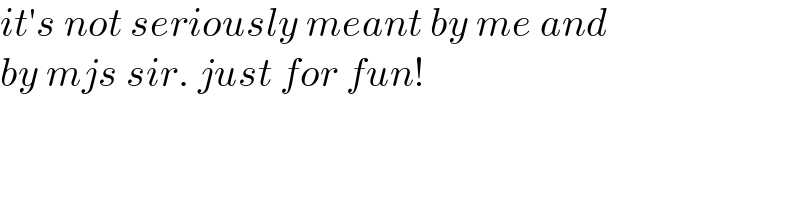
$${it}'{s}\:{not}\:{seriously}\:{meant}\:{by}\:{me}\:{and} \\ $$$${by}\:{mjs}\:{sir}.\:{just}\:{for}\:{fun}! \\ $$
Commented by liberty last updated on 08/Jan/21
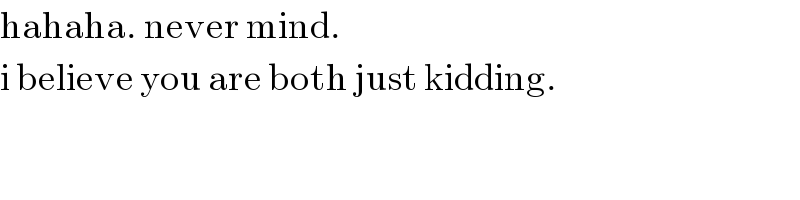
$$\mathrm{hahaha}.\:\mathrm{never}\:\mathrm{mind}. \\ $$$$\mathrm{i}\:\mathrm{believe}\:\mathrm{you}\:\mathrm{are}\:\mathrm{both}\:\mathrm{just}\:\mathrm{kidding}.\: \\ $$
