
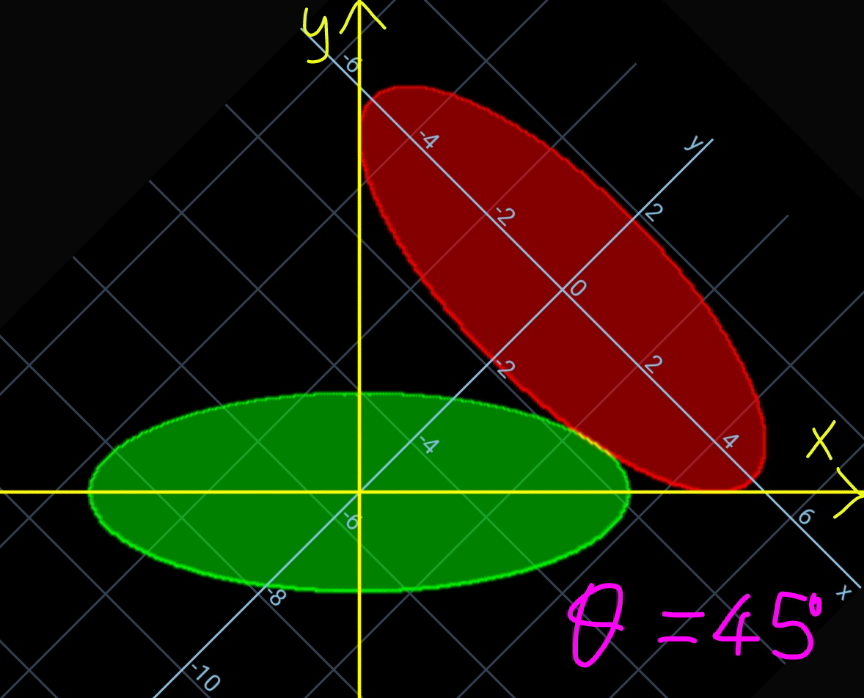
Question Number 127509 by mr W last updated on 30/Dec/20
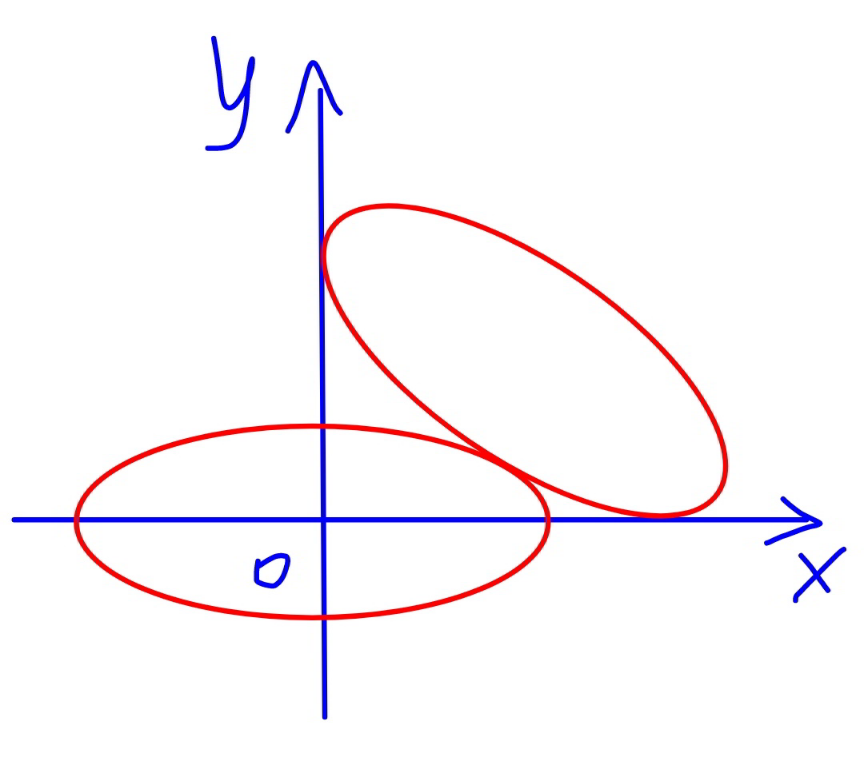
Commented by mr W last updated on 31/Dec/20

$${if}\:{both}\:{ellipses}\:{have}\:{the}\:{same}\:{shape} \\ $$$${as}\:\frac{{x}^{\mathrm{2}} }{{a}^{\mathrm{2}} }+\frac{{y}^{\mathrm{2}} }{{b}^{\mathrm{2}} }=\mathrm{1},\:{determine}\:\frac{{b}}{{a}}=? \\ $$
Commented by mr W last updated on 28/Jan/21
Answered by mr W last updated on 31/Dec/20
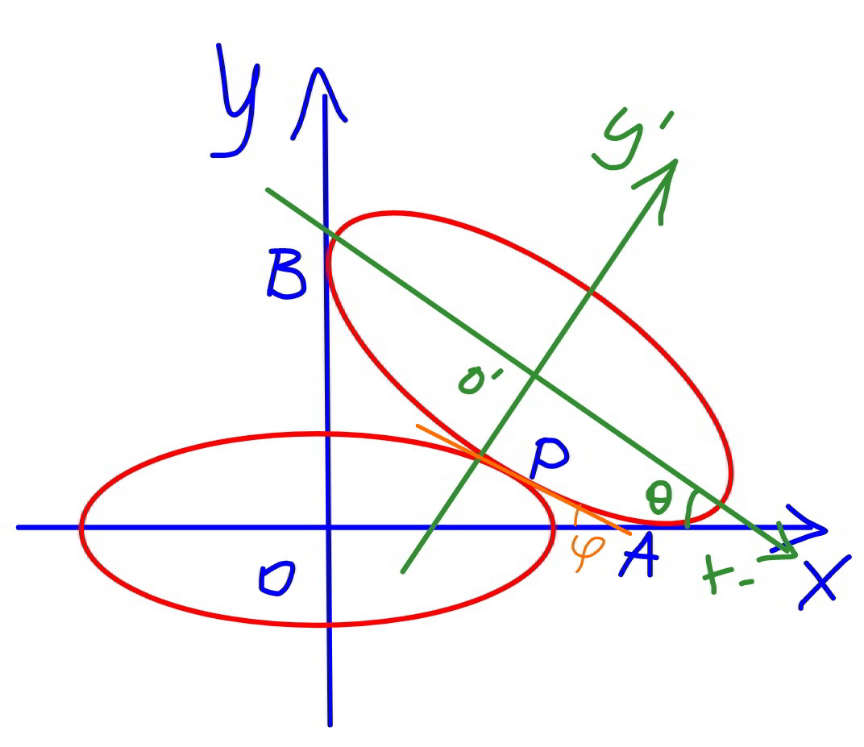
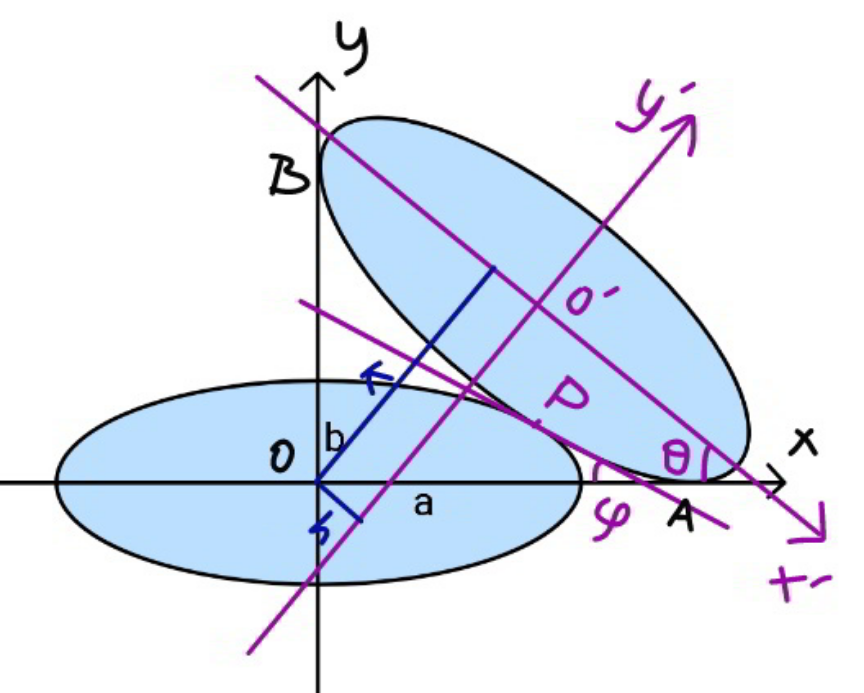
Commented by mr W last updated on 25/Jan/21
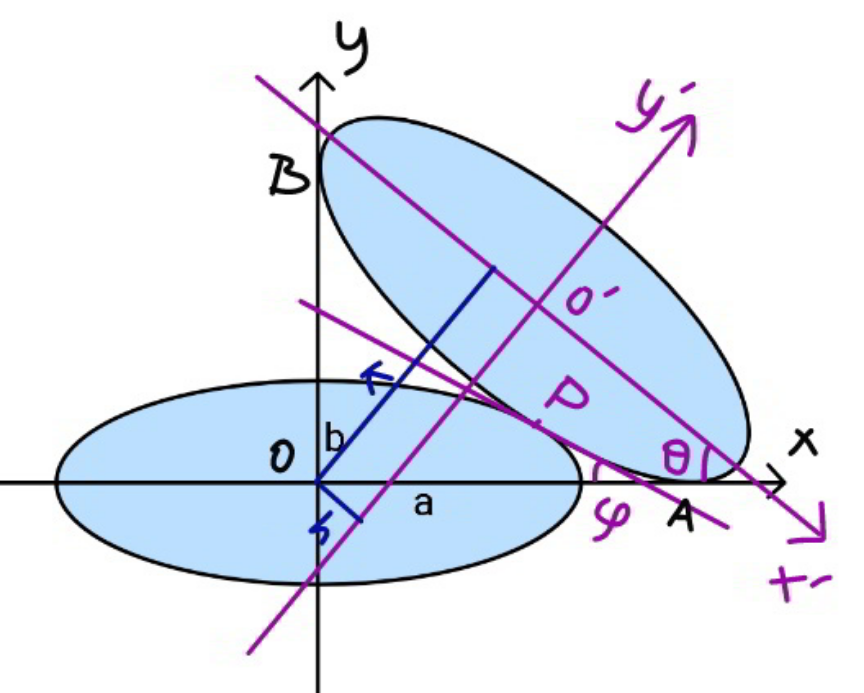
![let m=tan θ, μ=(b/a) ⇒sin θ=(m/( (√(1+m^2 )))), cos θ=(1/( (√(1+m^2 )))) in x′y′−system: O(h,k) eqn. of OA: y=k+m(x−h) ⇒mx−y+k−mh=0 OA is tangent to ellipse: a^2 m^2 +b^2 =(k−mh)^2 ⇒k−mh=−(√(a^2 m^2 +b^2 )) ...(i) eqn. of OB: y=k−(1/m)(x−h) ⇒(x/m)+y−k−(h/m)=0 OB is tangent to ellipse: (a^2 /m^2 )+b^2 =(k+(h/m))^2 ⇒k+(h/m)=−(√((a^2 /m^2 )+b^2 )) ...(ii) from (i) and (ii): ⇒(h/a)=−(1/(1+m^2 ))((√(1+m^2 μ^2 ))−m(√(m^2 +μ^2 ))) ⇒(k/a)=−(1/(1+m^2 ))(m(√(1+m^2 μ^2 ))+(√(m^2 +μ^2 ))) say P(a cos φ,−b sin φ) tan (θ−ϕ)=(dy/dx)=((−b cos φ)/(−a sin φ))=(μ/(tan φ)) ((m−tan ϕ)/(1+m tan ϕ))=(μ/(tan φ)) let η=tan φ sin φ=(η/( (√(1+η^2 )))), cos φ=(1/( (√(1+η^2 )))) ⇒tan ϕ=((η−(μ/m))/((η/m)+μ)) in xy−system: say P(x_p ,y_P ) x_P =(−k−b sin φ)sin θ+(−h+a cos φ)cos θ (x_P /a)=[(1/(1+m^2 ))(m(√(1+m^2 μ^2 ))+(√(m^2 +μ^2 )))−μ sin φ]sin θ+[(1/(1+m^2 ))((√(1+m^2 μ^2 ))−m(√(m^2 +μ^2 )))+cos φ]cos θ (x_P /a)=(m/( (√(1+m^2 ))))[(1/(1+m^2 ))(m(√(1+m^2 μ^2 ))+(√(m^2 +μ^2 )))−((μη)/( (√(1+η^2 ))))]+(1/( (√(1+m^2 ))))[(1/(1+m^2 ))((√(1+m^2 μ^2 ))−m(√(m^2 +μ^2 )))+(1/( (√(1+η^2 ))))] ⇒(x_P /a)=(1/( (√(1+m^2 ))))((√(1+m^2 μ^2 ))+((1−mμη)/( (√(1+η^2 ))))) y_P =(−k−b sin φ)cos θ−(−h+a cos φ)sin θ (y_P /a)=[(1/(1+m^2 ))(m(√(1+m^2 μ^2 ))+(√(m^2 +μ^2 )))−μ sin φ]cos θ−[(1/(1+m^2 ))((√(1+m^2 μ^2 ))−m(√(m^2 +μ^2 )))+cos φ]sin θ (y_P /a)=(1/( (√(1+m^2 ))))[(1/(1+m^2 ))(m(√(1+m^2 μ^2 ))+(√(m^2 +μ^2 )))−((μη)/( (√(1+η^2 ))))]−(m/( (√(1+m^2 ))))[(1/(1+m^2 ))((√(1+m^2 μ^2 ))−m(√(m^2 +μ^2 )))+(1/( (√(1+η^2 ))))] ⇒(y_P /a)=(1/( (√(1+m^2 ))))((√(m^2 +μ^2 ))−((m+μη)/( (√(1+η^2 ))))) (x_P ^2 /a^2 )+(y_P ^2 /b^2 )=1 ⇒((√(1+m^2 μ^2 ))+((1−mμη)/( (√(1+η^2 )))))^2 +(1/μ^2 )((√(m^2 +μ^2 ))−((m+μη)/( (√(1+η^2 )))))^2 =1+m^2 ...(I) tan ϕ=−(dy/dx)=((b/a))^2 (x_P /y_P ) ((η−(μ/m))/((η/m)+μ))=μ^2 ×(((√(1+m^2 μ^2 ))+((1−mμη)/( (√(1+η^2 )))))/( (√(m^2 +μ^2 ))−((m+μη)/( (√(1+η^2 )))))) ⇒μ^2 ((η/m)+μ)((√(1+m^2 μ^2 ))+((1−mμη)/( (√(1+η^2 )))))=(η−(μ/m))((√(m^2 +μ^2 ))−((m+μη)/( (√(1+η^2 ))))) ...(II) for any value in range 0<θ<60° we can find the coresponding μ from (I) and (II). we get μ_(max) ≈0.38436919447 at θ≈37.9382°](Q127600.png)
$${let}\:{m}=\mathrm{tan}\:\theta,\:\mu=\frac{{b}}{{a}} \\ $$$$\Rightarrow\mathrm{sin}\:\theta=\frac{{m}}{\:\sqrt{\mathrm{1}+{m}^{\mathrm{2}} }},\:\mathrm{cos}\:\theta=\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}+{m}^{\mathrm{2}} }} \\ $$$$ \\ $$$$\boldsymbol{{in}}\:\boldsymbol{{x}}'\boldsymbol{{y}}'−\boldsymbol{{system}}: \\ $$$${O}\left({h},{k}\right) \\ $$$${eqn}.\:{of}\:{OA}: \\ $$$${y}={k}+{m}\left({x}−{h}\right) \\ $$$$\Rightarrow{mx}−{y}+{k}−{mh}=\mathrm{0} \\ $$$${OA}\:{is}\:{tangent}\:{to}\:{ellipse}: \\ $$$${a}^{\mathrm{2}} {m}^{\mathrm{2}} +{b}^{\mathrm{2}} =\left({k}−{mh}\right)^{\mathrm{2}} \:\:\: \\ $$$$\Rightarrow{k}−{mh}=−\sqrt{{a}^{\mathrm{2}} {m}^{\mathrm{2}} +{b}^{\mathrm{2}} }\:\:\:...\left({i}\right) \\ $$$${eqn}.\:{of}\:{OB}: \\ $$$${y}={k}−\frac{\mathrm{1}}{{m}}\left({x}−{h}\right) \\ $$$$\Rightarrow\frac{{x}}{{m}}+{y}−{k}−\frac{{h}}{{m}}=\mathrm{0} \\ $$$${OB}\:{is}\:{tangent}\:{to}\:{ellipse}: \\ $$$$\frac{{a}^{\mathrm{2}} }{{m}^{\mathrm{2}} }+{b}^{\mathrm{2}} =\left({k}+\frac{{h}}{{m}}\right)^{\mathrm{2}} \\ $$$$\Rightarrow{k}+\frac{{h}}{{m}}=−\sqrt{\frac{{a}^{\mathrm{2}} }{{m}^{\mathrm{2}} }+{b}^{\mathrm{2}} }\:\:\:...\left({ii}\right) \\ $$$${from}\:\left({i}\right)\:{and}\:\left({ii}\right): \\ $$$$\Rightarrow\frac{{h}}{{a}}=−\frac{\mathrm{1}}{\mathrm{1}+{m}^{\mathrm{2}} }\left(\sqrt{\mathrm{1}+{m}^{\mathrm{2}} \mu^{\mathrm{2}} }−{m}\sqrt{{m}^{\mathrm{2}} +\mu^{\mathrm{2}} }\right) \\ $$$$\Rightarrow\frac{{k}}{{a}}=−\frac{\mathrm{1}}{\mathrm{1}+{m}^{\mathrm{2}} }\left({m}\sqrt{\mathrm{1}+{m}^{\mathrm{2}} \mu^{\mathrm{2}} }+\sqrt{{m}^{\mathrm{2}} +\mu^{\mathrm{2}} }\right) \\ $$$${say}\:{P}\left({a}\:\mathrm{cos}\:\phi,−{b}\:\mathrm{sin}\:\phi\right) \\ $$$$\mathrm{tan}\:\left(\theta−\varphi\right)=\frac{{dy}}{{dx}}=\frac{−{b}\:\mathrm{cos}\:\phi}{−{a}\:\mathrm{sin}\:\phi}=\frac{\mu}{\mathrm{tan}\:\:\phi} \\ $$$$\frac{{m}−\mathrm{tan}\:\varphi}{\mathrm{1}+{m}\:\mathrm{tan}\:\varphi}=\frac{\mu}{\mathrm{tan}\:\:\phi} \\ $$$${let}\:\eta=\mathrm{tan}\:\phi \\ $$$$\mathrm{sin}\:\phi=\frac{\eta}{\:\sqrt{\mathrm{1}+\eta^{\mathrm{2}} }},\:\mathrm{cos}\:\phi=\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}+\eta^{\mathrm{2}} }} \\ $$$$\Rightarrow\mathrm{tan}\:\varphi=\frac{\eta−\frac{\mu}{{m}}}{\frac{\eta}{{m}}+\mu} \\ $$$$ \\ $$$$\boldsymbol{{in}}\:\boldsymbol{{xy}}−\boldsymbol{{system}}: \\ $$$${say}\:{P}\left({x}_{{p}} ,{y}_{{P}} \right) \\ $$$${x}_{{P}} =\left(−{k}−{b}\:\mathrm{sin}\:\phi\right)\mathrm{sin}\:\theta+\left(−{h}+{a}\:\mathrm{cos}\:\phi\right)\mathrm{cos}\:\theta \\ $$$$\frac{{x}_{{P}} }{{a}}=\left[\frac{\mathrm{1}}{\mathrm{1}+{m}^{\mathrm{2}} }\left({m}\sqrt{\mathrm{1}+{m}^{\mathrm{2}} \mu^{\mathrm{2}} }+\sqrt{{m}^{\mathrm{2}} +\mu^{\mathrm{2}} }\right)−\mu\:\mathrm{sin}\:\phi\right]\mathrm{sin}\:\theta+\left[\frac{\mathrm{1}}{\mathrm{1}+{m}^{\mathrm{2}} }\left(\sqrt{\mathrm{1}+{m}^{\mathrm{2}} \mu^{\mathrm{2}} }−{m}\sqrt{{m}^{\mathrm{2}} +\mu^{\mathrm{2}} }\right)+\mathrm{cos}\:\phi\right]\mathrm{cos}\:\theta \\ $$$$\frac{{x}_{{P}} }{{a}}=\frac{{m}}{\:\sqrt{\mathrm{1}+{m}^{\mathrm{2}} }}\left[\frac{\mathrm{1}}{\mathrm{1}+{m}^{\mathrm{2}} }\left({m}\sqrt{\mathrm{1}+{m}^{\mathrm{2}} \mu^{\mathrm{2}} }+\sqrt{{m}^{\mathrm{2}} +\mu^{\mathrm{2}} }\right)−\frac{\mu\eta}{\:\sqrt{\mathrm{1}+\eta^{\mathrm{2}} }}\right]+\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}+{m}^{\mathrm{2}} }}\left[\frac{\mathrm{1}}{\mathrm{1}+{m}^{\mathrm{2}} }\left(\sqrt{\mathrm{1}+{m}^{\mathrm{2}} \mu^{\mathrm{2}} }−{m}\sqrt{{m}^{\mathrm{2}} +\mu^{\mathrm{2}} }\right)+\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}+\eta^{\mathrm{2}} }}\right] \\ $$$$\Rightarrow\frac{{x}_{{P}} }{{a}}=\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}+{m}^{\mathrm{2}} }}\left(\sqrt{\mathrm{1}+{m}^{\mathrm{2}} \mu^{\mathrm{2}} }+\frac{\mathrm{1}−{m}\mu\eta}{\:\sqrt{\mathrm{1}+\eta^{\mathrm{2}} }}\right) \\ $$$$ \\ $$$${y}_{{P}} =\left(−{k}−{b}\:\mathrm{sin}\:\phi\right)\mathrm{cos}\:\theta−\left(−{h}+{a}\:\mathrm{cos}\:\phi\right)\mathrm{sin}\:\theta \\ $$$$\frac{{y}_{{P}} }{{a}}=\left[\frac{\mathrm{1}}{\mathrm{1}+{m}^{\mathrm{2}} }\left({m}\sqrt{\mathrm{1}+{m}^{\mathrm{2}} \mu^{\mathrm{2}} }+\sqrt{{m}^{\mathrm{2}} +\mu^{\mathrm{2}} }\right)−\mu\:\mathrm{sin}\:\phi\right]\mathrm{cos}\:\theta−\left[\frac{\mathrm{1}}{\mathrm{1}+{m}^{\mathrm{2}} }\left(\sqrt{\mathrm{1}+{m}^{\mathrm{2}} \mu^{\mathrm{2}} }−{m}\sqrt{{m}^{\mathrm{2}} +\mu^{\mathrm{2}} }\right)+\mathrm{cos}\:\phi\right]\mathrm{sin}\:\theta \\ $$$$\frac{{y}_{{P}} }{{a}}=\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}+{m}^{\mathrm{2}} }}\left[\frac{\mathrm{1}}{\mathrm{1}+{m}^{\mathrm{2}} }\left({m}\sqrt{\mathrm{1}+{m}^{\mathrm{2}} \mu^{\mathrm{2}} }+\sqrt{{m}^{\mathrm{2}} +\mu^{\mathrm{2}} }\right)−\frac{\mu\eta}{\:\sqrt{\mathrm{1}+\eta^{\mathrm{2}} }}\right]−\frac{{m}}{\:\sqrt{\mathrm{1}+{m}^{\mathrm{2}} }}\left[\frac{\mathrm{1}}{\mathrm{1}+{m}^{\mathrm{2}} }\left(\sqrt{\mathrm{1}+{m}^{\mathrm{2}} \mu^{\mathrm{2}} }−{m}\sqrt{{m}^{\mathrm{2}} +\mu^{\mathrm{2}} }\right)+\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}+\eta^{\mathrm{2}} }}\right] \\ $$$$\Rightarrow\frac{{y}_{{P}} }{{a}}=\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}+{m}^{\mathrm{2}} }}\left(\sqrt{{m}^{\mathrm{2}} +\mu^{\mathrm{2}} }−\frac{{m}+\mu\eta}{\:\sqrt{\mathrm{1}+\eta^{\mathrm{2}} }}\right) \\ $$$$\frac{{x}_{{P}} ^{\mathrm{2}} }{{a}^{\mathrm{2}} }+\frac{{y}_{{P}} ^{\mathrm{2}} }{{b}^{\mathrm{2}} }=\mathrm{1} \\ $$$$\Rightarrow\left(\sqrt{\mathrm{1}+{m}^{\mathrm{2}} \mu^{\mathrm{2}} }+\frac{\mathrm{1}−{m}\mu\eta}{\:\sqrt{\mathrm{1}+\eta^{\mathrm{2}} }}\right)^{\mathrm{2}} +\frac{\mathrm{1}}{\mu^{\mathrm{2}} }\left(\sqrt{{m}^{\mathrm{2}} +\mu^{\mathrm{2}} }−\frac{{m}+\mu\eta}{\:\sqrt{\mathrm{1}+\eta^{\mathrm{2}} }}\right)^{\mathrm{2}} =\mathrm{1}+{m}^{\mathrm{2}} \:\:\:...\left({I}\right) \\ $$$$\mathrm{tan}\:\varphi=−\frac{{dy}}{{dx}}=\left(\frac{{b}}{{a}}\right)^{\mathrm{2}} \frac{{x}_{{P}} }{{y}_{{P}} } \\ $$$$\frac{\eta−\frac{\mu}{{m}}}{\frac{\eta}{{m}}+\mu}=\mu^{\mathrm{2}} ×\frac{\sqrt{\mathrm{1}+{m}^{\mathrm{2}} \mu^{\mathrm{2}} }+\frac{\mathrm{1}−{m}\mu\eta}{\:\sqrt{\mathrm{1}+\eta^{\mathrm{2}} }}}{\:\sqrt{{m}^{\mathrm{2}} +\mu^{\mathrm{2}} }−\frac{{m}+\mu\eta}{\:\sqrt{\mathrm{1}+\eta^{\mathrm{2}} }}} \\ $$$$\Rightarrow\mu^{\mathrm{2}} \left(\frac{\eta}{{m}}+\mu\right)\left(\sqrt{\mathrm{1}+{m}^{\mathrm{2}} \mu^{\mathrm{2}} }+\frac{\mathrm{1}−{m}\mu\eta}{\:\sqrt{\mathrm{1}+\eta^{\mathrm{2}} }}\right)=\left(\eta−\frac{\mu}{{m}}\right)\left(\sqrt{{m}^{\mathrm{2}} +\mu^{\mathrm{2}} }−\frac{{m}+\mu\eta}{\:\sqrt{\mathrm{1}+\eta^{\mathrm{2}} }}\right)\:\:\:...\left({II}\right) \\ $$$$ \\ $$$${for}\:{any}\:{value}\:{in}\:{range}\:\mathrm{0}<\theta<\mathrm{60}°\:{we}\: \\ $$$${can}\:{find}\:{the}\:{coresponding}\:\mu\:{from} \\ $$$$\left({I}\right)\:{and}\:\left({II}\right). \\ $$$$ \\ $$$${we}\:{get}\:\mu_{{max}} \approx\mathrm{0}.\mathrm{38436919447}\:{at}\:\theta\approx\mathrm{37}.\mathrm{9382}° \\ $$
Commented by mr W last updated on 31/Dec/20
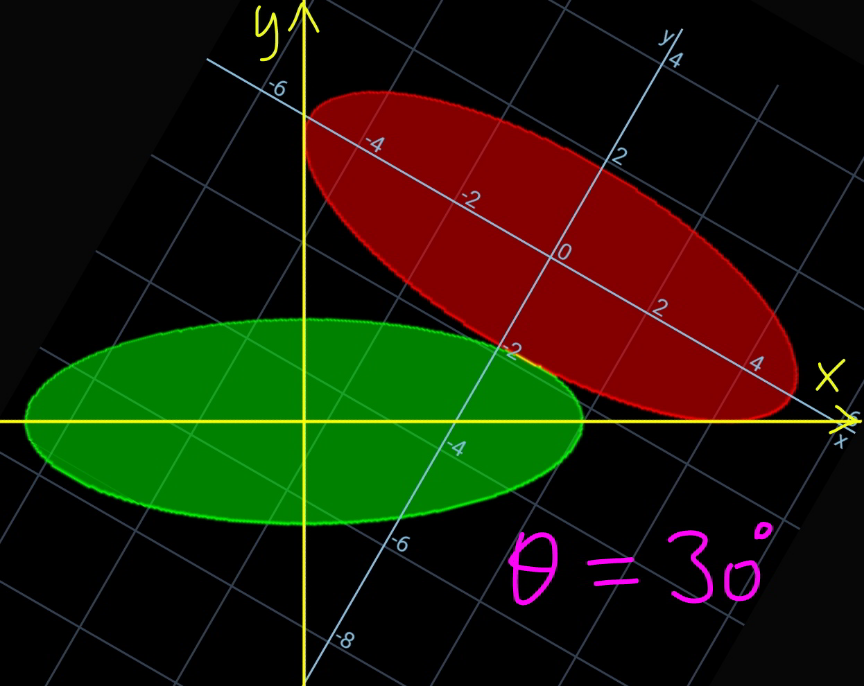
Commented by mr W last updated on 31/Dec/20
Commented by mr W last updated on 31/Dec/20

Commented by mr W last updated on 02/Jan/21

Answered by mr W last updated on 30/Jan/21
Commented by mr W last updated on 30/Jan/21
![let m=tan θ, μ=(b/a) ⇒sin θ=(m/( (√(1+m^2 )))), cos θ=(1/( (√(1+m^2 )))) in x′y′−system: O(h,k) eqn. of OA: y=k+m(x−h) ⇒mx−y+k−mh=0 OA is tangent to ellipse: a^2 m^2 +b^2 =(k−mh)^2 ⇒k−mh=−(√(a^2 m^2 +b^2 )) ...(i) eqn. of OB: y=k−(1/m)(x−h) ⇒(x/m)+y−k−(h/m)=0 OB is tangent to ellipse: (a^2 /m^2 )+b^2 =(k+(h/m))^2 ⇒k+(h/m)=−(√((a^2 /m^2 )+b^2 )) ...(ii) from (i) and (ii): ⇒(h/a)=−(1/(1+m^2 ))((√(1+m^2 μ^2 ))−m(√(m^2 +μ^2 ))) ⇒(k/a)=−(1/(1+m^2 ))(m(√(1+m^2 μ^2 ))+(√(m^2 +μ^2 ))) in xy−system: say O′(x_C ,y_C ) x_C =−k sin θ−h cos θ (x_C /a)=(1/(1+m^2 ))((√(1+m^2 μ^2 ))−m(√(m^2 +μ^2 ))) cos θ+(1/(1+m^2 ))(m(√(1+m^2 μ^2 ))+(√(m^2 +μ^2 ))) sin θ ⇒(x_C /a)=((√(1+m^2 μ^2 ))/( (√(1+m^2 )))) y_C =−k cos θ+h sin θ (y_C /a)=−(1/(1+m^2 ))((√(1+m^2 μ^2 ))−m(√(m^2 +μ^2 ))) sin θ+(1/(1+m^2 ))(m(√(1+m^2 μ^2 ))+(√(m^2 +μ^2 ))) cos θ ⇒(y_C /a)=((√(m^2 +μ^2 ))/( (√(1+m^2 )))) (x^2 /a^2 )+(y^2 /b^2 )=1 ⇒μ^2 ξ^2 +η^2 =μ^2 ...(i) ⇒η=μ(√(1−ξ^2 )) eqn. of ellipse O′: (([(x−x_C )(1/( (√(1+m^2 ))))−(y−y_C )(m/( (√(1+m^2 ))))+x_C ]^2 )/a^2 )+(([(x−x_C )sin θ−(y−y_C )cos θ+y_C ]^2 )/b^2 )=1 [((x/a)−((√(1+m^2 μ^2 ))/( (√(1+m^2 )))))−m((y/a)−((√(m^2 +μ^2 ))/( (√(1+m^2 )))))+(√(1+m^2 μ^2 ))]^2 +(([m((x/a)−((√(1+m^2 μ^2 ))/( (√(1+m^2 )))))+((y/a)−((√(m^2 +μ^2 ))/( (√(1+m^2 )))))+(√(m^2 +μ^2 ))]^2 )/μ^2 )=1+m^2 ⇒μ^2 [ξ−mη+((m(√(m^2 +μ^2 ))−(√(1+m^2 μ^2 )))/( (√(1+m^2 ))))+(√(1+m^2 μ^2 ))]^2 +[mξ+η−(((√(m^2 +μ^2 ))+m(√(1+m^2 μ^2 )))/( (√(1+m^2 ))))+(√(m^2 +μ^2 ))]^2 =μ^2 (1+m^2 ) ...(ii) ⇒μ^2 (ξ−mη+p)^2 +(mξ+η+q)^2 =μ^2 (1+m^2 ) ...(ii) ......](Q130923.png)
$${let}\:{m}=\mathrm{tan}\:\theta,\:\mu=\frac{{b}}{{a}} \\ $$$$\Rightarrow\mathrm{sin}\:\theta=\frac{{m}}{\:\sqrt{\mathrm{1}+{m}^{\mathrm{2}} }},\:\mathrm{cos}\:\theta=\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}+{m}^{\mathrm{2}} }} \\ $$$$ \\ $$$$\boldsymbol{{in}}\:\boldsymbol{{x}}'\boldsymbol{{y}}'−\boldsymbol{{system}}: \\ $$$${O}\left({h},{k}\right) \\ $$$${eqn}.\:{of}\:{OA}: \\ $$$${y}={k}+{m}\left({x}−{h}\right) \\ $$$$\Rightarrow{mx}−{y}+{k}−{mh}=\mathrm{0} \\ $$$${OA}\:{is}\:{tangent}\:{to}\:{ellipse}: \\ $$$${a}^{\mathrm{2}} {m}^{\mathrm{2}} +{b}^{\mathrm{2}} =\left({k}−{mh}\right)^{\mathrm{2}} \:\:\: \\ $$$$\Rightarrow{k}−{mh}=−\sqrt{{a}^{\mathrm{2}} {m}^{\mathrm{2}} +{b}^{\mathrm{2}} }\:\:\:...\left({i}\right) \\ $$$${eqn}.\:{of}\:{OB}: \\ $$$${y}={k}−\frac{\mathrm{1}}{{m}}\left({x}−{h}\right) \\ $$$$\Rightarrow\frac{{x}}{{m}}+{y}−{k}−\frac{{h}}{{m}}=\mathrm{0} \\ $$$${OB}\:{is}\:{tangent}\:{to}\:{ellipse}: \\ $$$$\frac{{a}^{\mathrm{2}} }{{m}^{\mathrm{2}} }+{b}^{\mathrm{2}} =\left({k}+\frac{{h}}{{m}}\right)^{\mathrm{2}} \\ $$$$\Rightarrow{k}+\frac{{h}}{{m}}=−\sqrt{\frac{{a}^{\mathrm{2}} }{{m}^{\mathrm{2}} }+{b}^{\mathrm{2}} }\:\:\:...\left({ii}\right) \\ $$$${from}\:\left({i}\right)\:{and}\:\left({ii}\right): \\ $$$$\Rightarrow\frac{{h}}{{a}}=−\frac{\mathrm{1}}{\mathrm{1}+{m}^{\mathrm{2}} }\left(\sqrt{\mathrm{1}+{m}^{\mathrm{2}} \mu^{\mathrm{2}} }−{m}\sqrt{{m}^{\mathrm{2}} +\mu^{\mathrm{2}} }\right) \\ $$$$\Rightarrow\frac{{k}}{{a}}=−\frac{\mathrm{1}}{\mathrm{1}+{m}^{\mathrm{2}} }\left({m}\sqrt{\mathrm{1}+{m}^{\mathrm{2}} \mu^{\mathrm{2}} }+\sqrt{{m}^{\mathrm{2}} +\mu^{\mathrm{2}} }\right) \\ $$$$ \\ $$$$\boldsymbol{{in}}\:\boldsymbol{{xy}}−\boldsymbol{{system}}: \\ $$$${say}\:{O}'\left({x}_{{C}} ,{y}_{{C}} \right) \\ $$$${x}_{{C}} =−{k}\:\mathrm{sin}\:\theta−{h}\:\mathrm{cos}\:\theta \\ $$$$\frac{{x}_{{C}} }{{a}}=\frac{\mathrm{1}}{\mathrm{1}+{m}^{\mathrm{2}} }\left(\sqrt{\mathrm{1}+{m}^{\mathrm{2}} \mu^{\mathrm{2}} }−{m}\sqrt{{m}^{\mathrm{2}} +\mu^{\mathrm{2}} }\right)\:\mathrm{cos}\:\theta+\frac{\mathrm{1}}{\mathrm{1}+{m}^{\mathrm{2}} }\left({m}\sqrt{\mathrm{1}+{m}^{\mathrm{2}} \mu^{\mathrm{2}} }+\sqrt{{m}^{\mathrm{2}} +\mu^{\mathrm{2}} }\right)\:\mathrm{sin}\:\theta \\ $$$$\Rightarrow\frac{{x}_{{C}} }{{a}}=\frac{\sqrt{\mathrm{1}+{m}^{\mathrm{2}} \mu^{\mathrm{2}} }}{\:\sqrt{\mathrm{1}+{m}^{\mathrm{2}} }} \\ $$$${y}_{{C}} =−{k}\:\mathrm{cos}\:\theta+{h}\:\mathrm{sin}\:\theta \\ $$$$\frac{{y}_{{C}} }{{a}}=−\frac{\mathrm{1}}{\mathrm{1}+{m}^{\mathrm{2}} }\left(\sqrt{\mathrm{1}+{m}^{\mathrm{2}} \mu^{\mathrm{2}} }−{m}\sqrt{{m}^{\mathrm{2}} +\mu^{\mathrm{2}} }\right)\:\mathrm{sin}\:\theta+\frac{\mathrm{1}}{\mathrm{1}+{m}^{\mathrm{2}} }\left({m}\sqrt{\mathrm{1}+{m}^{\mathrm{2}} \mu^{\mathrm{2}} }+\sqrt{{m}^{\mathrm{2}} +\mu^{\mathrm{2}} }\right)\:\mathrm{cos}\:\theta \\ $$$$\Rightarrow\frac{{y}_{{C}} }{{a}}=\frac{\sqrt{{m}^{\mathrm{2}} +\mu^{\mathrm{2}} }}{\:\sqrt{\mathrm{1}+{m}^{\mathrm{2}} }} \\ $$$$\frac{{x}^{\mathrm{2}} }{{a}^{\mathrm{2}} }+\frac{{y}^{\mathrm{2}} }{{b}^{\mathrm{2}} }=\mathrm{1} \\ $$$$\Rightarrow\mu^{\mathrm{2}} \xi^{\mathrm{2}} +\eta^{\mathrm{2}} =\mu^{\mathrm{2}} \:\:\:...\left({i}\right)\:\:\:\: \\ $$$$\Rightarrow\eta=\mu\sqrt{\mathrm{1}−\xi^{\mathrm{2}} } \\ $$$${eqn}.\:{of}\:{ellipse}\:{O}': \\ $$$$\frac{\left[\left({x}−{x}_{{C}} \right)\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}+{m}^{\mathrm{2}} }}−\left({y}−{y}_{{C}} \right)\frac{{m}}{\:\sqrt{\mathrm{1}+{m}^{\mathrm{2}} }}+{x}_{{C}} \right]^{\mathrm{2}} }{{a}^{\mathrm{2}} }+\frac{\left[\left({x}−{x}_{{C}} \right)\mathrm{sin}\:\theta−\left({y}−{y}_{{C}} \right)\mathrm{cos}\:\theta+{y}_{{C}} \right]^{\mathrm{2}} }{{b}^{\mathrm{2}} }=\mathrm{1} \\ $$$$\left[\left(\frac{{x}}{{a}}−\frac{\sqrt{\mathrm{1}+{m}^{\mathrm{2}} \mu^{\mathrm{2}} }}{\:\sqrt{\mathrm{1}+{m}^{\mathrm{2}} }}\right)−{m}\left(\frac{{y}}{{a}}−\frac{\sqrt{{m}^{\mathrm{2}} +\mu^{\mathrm{2}} }}{\:\sqrt{\mathrm{1}+{m}^{\mathrm{2}} }}\right)+\sqrt{\mathrm{1}+{m}^{\mathrm{2}} \mu^{\mathrm{2}} }\right]^{\mathrm{2}} +\frac{\left[{m}\left(\frac{{x}}{{a}}−\frac{\sqrt{\mathrm{1}+{m}^{\mathrm{2}} \mu^{\mathrm{2}} }}{\:\sqrt{\mathrm{1}+{m}^{\mathrm{2}} }}\right)+\left(\frac{{y}}{{a}}−\frac{\sqrt{{m}^{\mathrm{2}} +\mu^{\mathrm{2}} }}{\:\sqrt{\mathrm{1}+{m}^{\mathrm{2}} }}\right)+\sqrt{{m}^{\mathrm{2}} +\mu^{\mathrm{2}} }\right]^{\mathrm{2}} }{\mu^{\mathrm{2}} }=\mathrm{1}+{m}^{\mathrm{2}} \\ $$$$\Rightarrow\mu^{\mathrm{2}} \left[\xi−{m}\eta+\frac{{m}\sqrt{{m}^{\mathrm{2}} +\mu^{\mathrm{2}} }−\sqrt{\mathrm{1}+{m}^{\mathrm{2}} \mu^{\mathrm{2}} }}{\:\sqrt{\mathrm{1}+{m}^{\mathrm{2}} }}+\sqrt{\mathrm{1}+{m}^{\mathrm{2}} \mu^{\mathrm{2}} }\right]^{\mathrm{2}} +\left[{m}\xi+\eta−\frac{\sqrt{{m}^{\mathrm{2}} +\mu^{\mathrm{2}} }+{m}\sqrt{\mathrm{1}+{m}^{\mathrm{2}} \mu^{\mathrm{2}} }}{\:\sqrt{\mathrm{1}+{m}^{\mathrm{2}} }}+\sqrt{{m}^{\mathrm{2}} +\mu^{\mathrm{2}} }\right]^{\mathrm{2}} =\mu^{\mathrm{2}} \left(\mathrm{1}+{m}^{\mathrm{2}} \right)\:\:\:...\left({ii}\right) \\ $$$$\Rightarrow\mu^{\mathrm{2}} \left(\xi−{m}\eta+{p}\right)^{\mathrm{2}} +\left({m}\xi+\eta+{q}\right)^{\mathrm{2}} =\mu^{\mathrm{2}} \left(\mathrm{1}+{m}^{\mathrm{2}} \right)\:\:\:...\left({ii}\right) \\ $$$$...... \\ $$
