
Question Number 126669 by Dwaipayan Shikari last updated on 23/Dec/20

$$\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{{H}_{{n}} ^{\mathrm{2}} }{{n}^{\mathrm{4}} } \\ $$
Commented by talminator2856791 last updated on 23/Dec/20

$$\:\mathrm{what}\:\mathrm{does}\:{H}\:\mathrm{mean}? \\ $$
Commented by Dwaipayan Shikari last updated on 23/Dec/20
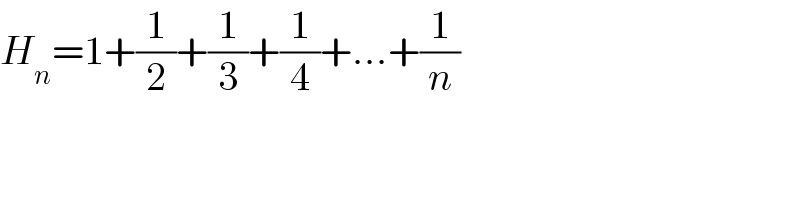
$${H}_{{n}} =\mathrm{1}+\frac{\mathrm{1}}{\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{3}}+\frac{\mathrm{1}}{\mathrm{4}}+...+\frac{\mathrm{1}}{{n}} \\ $$
Answered by Olaf last updated on 23/Dec/20

$${Hello}\:{from}\:{France}.\:{I}\:{know}\:{this}\:{is}\:{not} \\ $$$${the}\:{place}\:{here}\:{but}\:{I}\:{wish}\:{you}\:{a}\:{Merry} \\ $$$${Chistmas}.\:{Mr}\:{Shikari}\:{your}\:{questions} \\ $$$${are}\:{always}\:{difficult}\:{but}\:{very} \\ $$$${interesting}.\:{Thank}\:{you}\:{very}\:{much}\:{for} \\ $$$${helping}\:{me}\:{improve}.\:{Thanks}\:{to}\:{you}, \\ $$$${I}\:{learn}\:{a}\:{lot}\:{of}\:{things},\:{you}\:{are}\:{my} \\ $$$${best}\:{teacher}. \\ $$$${Olaf}\:{Thorendsen}. \\ $$
Commented by Dwaipayan Shikari last updated on 23/Dec/20
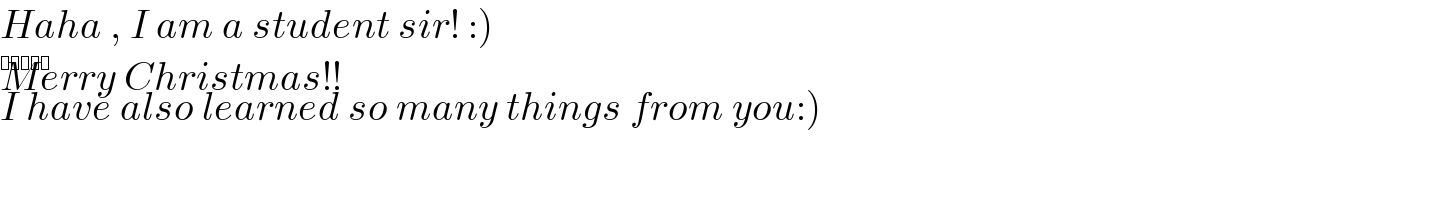
$$\left.{Haha}\:,\:{I}\:{am}\:{a}\:{student}\:{sir}!\::\right) \\ $$$${Merry}\:{Christmas}!!\: \\ $$⛄🎅🤶🦌🎄 $$\left.{I}\:{have}\:{also}\:{learned}\:{so}\:{many}\:{things}\:{from}\:{you}:\right) \\ $$$$ \\ $$
Commented by Dwaipayan Shikari last updated on 23/Dec/20
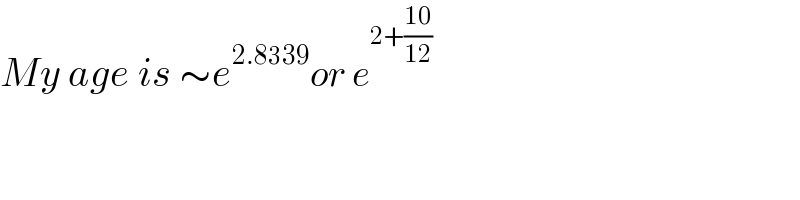
$${My}\:{age}\:{is}\:\sim{e}^{\mathrm{2}.\mathrm{8339}} {or}\:{e}^{\mathrm{2}+\frac{\mathrm{10}}{\mathrm{12}}} \\ $$
Commented by liberty last updated on 23/Dec/20
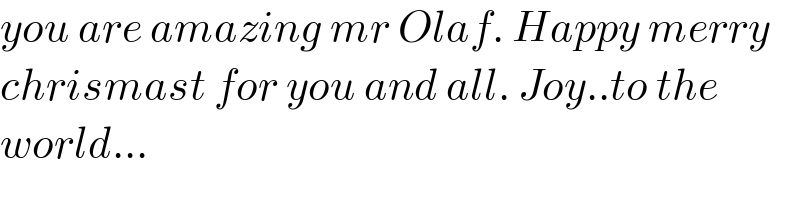
$${you}\:{are}\:{amazing}\:{mr}\:{Olaf}.\:{Happy}\:{merry} \\ $$$${chrismast}\:{for}\:{you}\:{and}\:{all}.\:{Joy}..{to}\:{the} \\ $$$${world}... \\ $$
