
Question Number 126609 by abdullahquwatan last updated on 22/Dec/20

$$\mathrm{cube}\:\mathrm{ABCD}.\mathrm{EFGH}\:{side}\:\mathrm{4}\:{cm}\:{point}\:{P}\:{is}\:{center}\:{BF}\:{and}\:{Q}\:{center}\:{AD}.\:{distance}\:{E}\:{to}\:{PQG} \\ $$
Answered by liberty last updated on 22/Dec/20
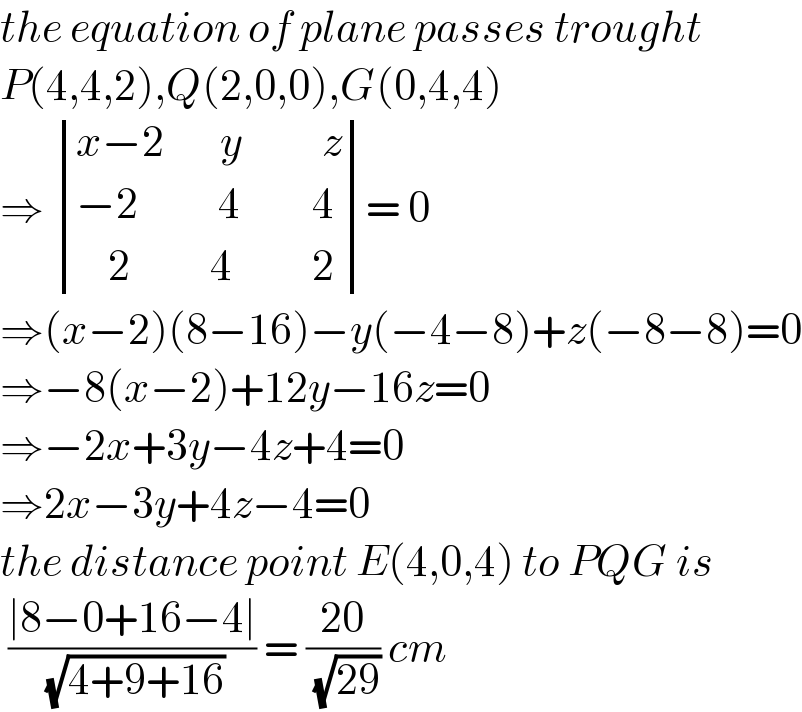
$${the}\:{equation}\:{of}\:{plane}\:{passes}\:{trought} \\ $$$${P}\left(\mathrm{4},\mathrm{4},\mathrm{2}\right),{Q}\left(\mathrm{2},\mathrm{0},\mathrm{0}\right),{G}\left(\mathrm{0},\mathrm{4},\mathrm{4}\right) \\ $$$$\Rightarrow\:\begin{vmatrix}{{x}−\mathrm{2}\:\:\:\:\:\:\:{y}\:\:\:\:\:\:\:\:\:\:{z}}\\{−\mathrm{2}\:\:\:\:\:\:\:\:\:\:\mathrm{4}\:\:\:\:\:\:\:\:\:\mathrm{4}}\\{\:\:\:\:\mathrm{2}\:\:\:\:\:\:\:\:\:\:\mathrm{4}\:\:\:\:\:\:\:\:\:\:\mathrm{2}}\end{vmatrix}=\:\mathrm{0} \\ $$$$\Rightarrow\left({x}−\mathrm{2}\right)\left(\mathrm{8}−\mathrm{16}\right)−{y}\left(−\mathrm{4}−\mathrm{8}\right)+{z}\left(−\mathrm{8}−\mathrm{8}\right)=\mathrm{0} \\ $$$$\Rightarrow−\mathrm{8}\left({x}−\mathrm{2}\right)+\mathrm{12}{y}−\mathrm{16}{z}=\mathrm{0} \\ $$$$\Rightarrow−\mathrm{2}{x}+\mathrm{3}{y}−\mathrm{4}{z}+\mathrm{4}=\mathrm{0} \\ $$$$\Rightarrow\mathrm{2}{x}−\mathrm{3}{y}+\mathrm{4}{z}−\mathrm{4}=\mathrm{0} \\ $$$${the}\:{distance}\:{point}\:{E}\left(\mathrm{4},\mathrm{0},\mathrm{4}\right)\:{to}\:{PQG}\:{is} \\ $$$$\:\frac{\mid\mathrm{8}−\mathrm{0}+\mathrm{16}−\mathrm{4}\mid}{\:\sqrt{\mathrm{4}+\mathrm{9}+\mathrm{16}}}\:=\:\frac{\mathrm{20}}{\:\sqrt{\mathrm{29}}}\:{cm} \\ $$
Commented by abdullahquwatan last updated on 22/Dec/20

$${thank}\:{you}\:{sir} \\ $$
