
Question Number 126604 by bramlexs22 last updated on 22/Dec/20
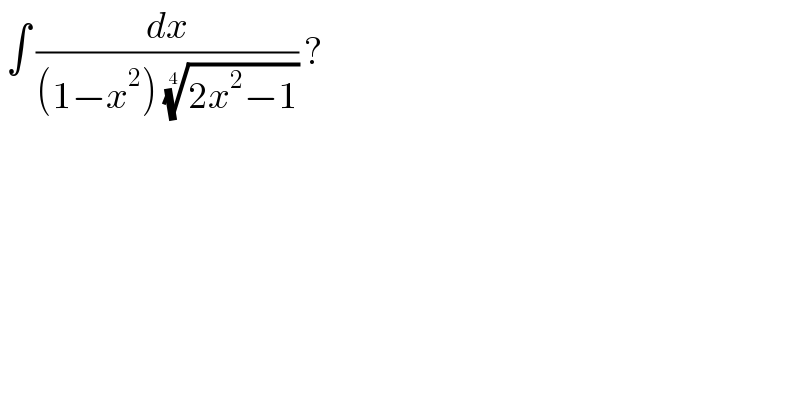
$$\:\int\:\frac{{dx}}{\left(\mathrm{1}−{x}^{\mathrm{2}} \right)\:\sqrt[{\mathrm{4}}]{\mathrm{2}{x}^{\mathrm{2}} −\mathrm{1}}}\:? \\ $$
Answered by liberty last updated on 22/Dec/20
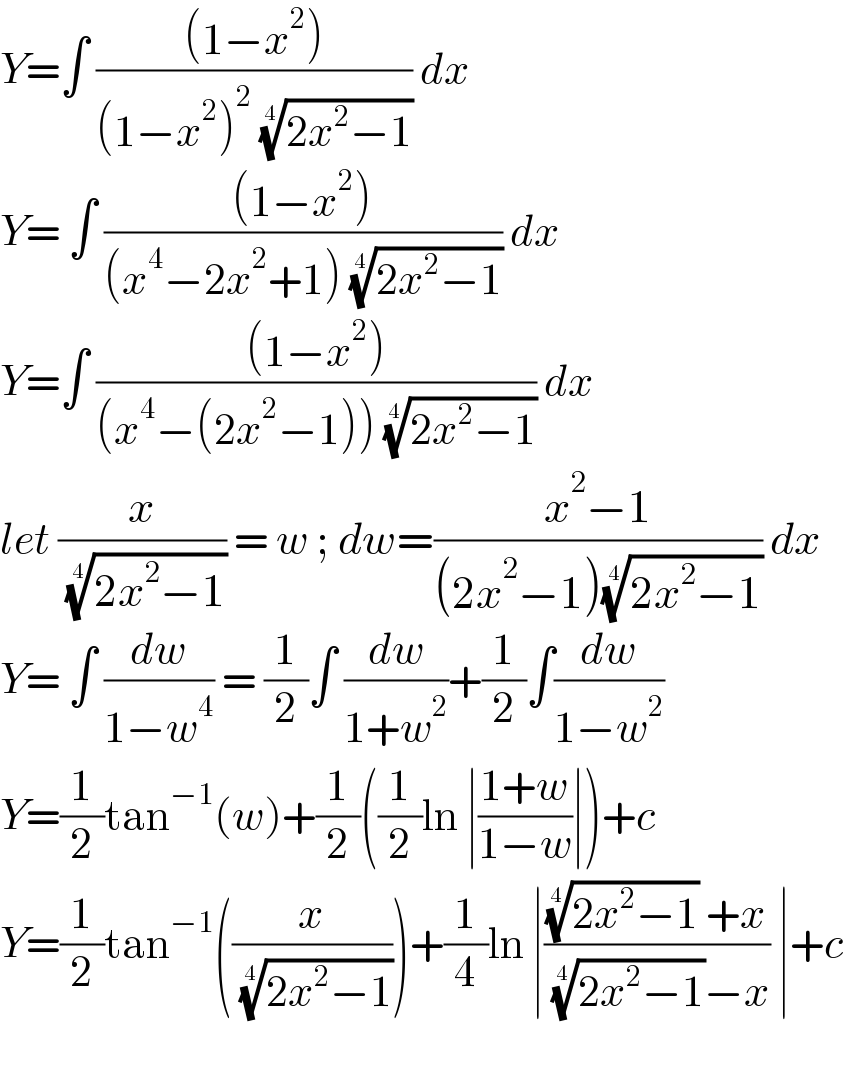
$${Y}=\int\:\frac{\left(\mathrm{1}−{x}^{\mathrm{2}} \right)}{\left(\mathrm{1}−{x}^{\mathrm{2}} \right)^{\mathrm{2}} \:\sqrt[{\mathrm{4}}]{\mathrm{2}{x}^{\mathrm{2}} −\mathrm{1}}}\:{dx} \\ $$$${Y}=\:\int\:\frac{\left(\mathrm{1}−{x}^{\mathrm{2}} \right)}{\left({x}^{\mathrm{4}} −\mathrm{2}{x}^{\mathrm{2}} +\mathrm{1}\right)\:\sqrt[{\mathrm{4}}]{\mathrm{2}{x}^{\mathrm{2}} −\mathrm{1}}}\:{dx} \\ $$$${Y}=\int\:\frac{\left(\mathrm{1}−{x}^{\mathrm{2}} \right)}{\left({x}^{\mathrm{4}} −\left(\mathrm{2}{x}^{\mathrm{2}} −\mathrm{1}\right)\right)\:\sqrt[{\mathrm{4}}]{\mathrm{2}{x}^{\mathrm{2}} −\mathrm{1}}}\:{dx}\: \\ $$$${let}\:\frac{{x}}{\:\sqrt[{\mathrm{4}}]{\mathrm{2}{x}^{\mathrm{2}} −\mathrm{1}}}\:=\:{w}\:;\:{dw}=\frac{{x}^{\mathrm{2}} −\mathrm{1}}{\left(\mathrm{2}{x}^{\mathrm{2}} −\mathrm{1}\right)\sqrt[{\mathrm{4}}]{\mathrm{2}{x}^{\mathrm{2}} −\mathrm{1}}}\:{dx} \\ $$$${Y}=\:\int\:\frac{{dw}}{\mathrm{1}−{w}^{\mathrm{4}} }\:=\:\frac{\mathrm{1}}{\mathrm{2}}\int\:\frac{{dw}}{\mathrm{1}+{w}^{\mathrm{2}} }+\frac{\mathrm{1}}{\mathrm{2}}\int\frac{{dw}}{\mathrm{1}−{w}^{\mathrm{2}} } \\ $$$${Y}=\frac{\mathrm{1}}{\mathrm{2}}\mathrm{tan}^{−\mathrm{1}} \left({w}\right)+\frac{\mathrm{1}}{\mathrm{2}}\left(\frac{\mathrm{1}}{\mathrm{2}}\mathrm{ln}\:\mid\frac{\mathrm{1}+{w}}{\mathrm{1}−{w}}\mid\right)+{c} \\ $$$${Y}=\frac{\mathrm{1}}{\mathrm{2}}\mathrm{tan}^{−\mathrm{1}} \left(\frac{{x}}{\:\sqrt[{\mathrm{4}}]{\mathrm{2}{x}^{\mathrm{2}} −\mathrm{1}}}\right)+\frac{\mathrm{1}}{\mathrm{4}}\mathrm{ln}\:\mid\frac{\sqrt[{\mathrm{4}}]{\mathrm{2}{x}^{\mathrm{2}} −\mathrm{1}}\:+{x}}{\:\sqrt[{\mathrm{4}}]{\mathrm{2}{x}^{\mathrm{2}} −\mathrm{1}}−{x}}\:\mid+{c}\: \\ $$$$ \\ $$
