
Question Number 126349 by mnjuly1970 last updated on 19/Dec/20
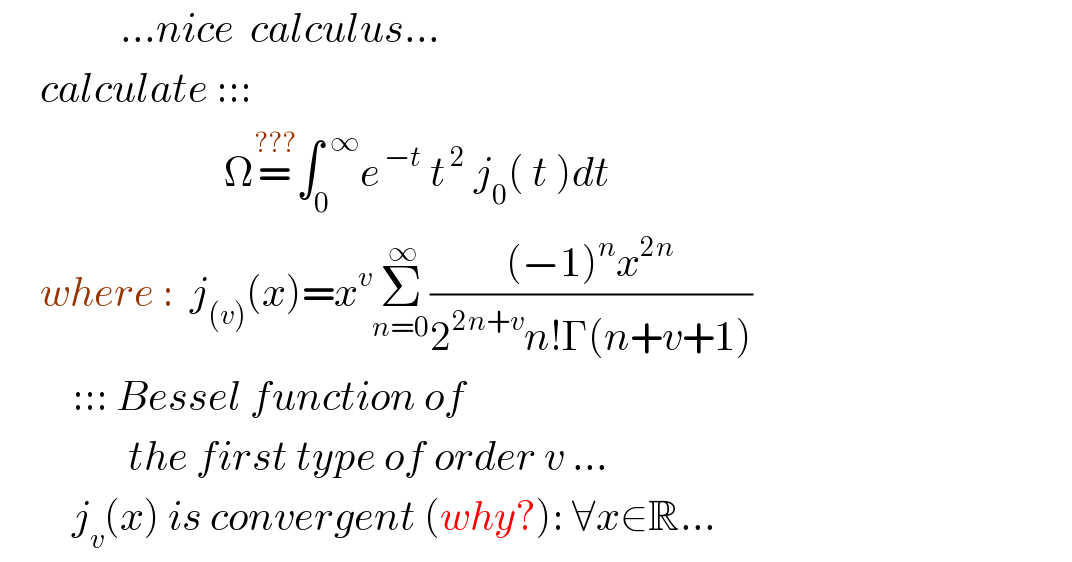
$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:...{nice}\:\:{calculus}... \\ $$$$\:\:\:\:\:{calculate}\:::: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\Omega\overset{???} {=}\int_{\mathrm{0}} ^{\:\:\infty} {e}^{\:−{t}} \:{t}^{\:\mathrm{2}} \:{j}_{\mathrm{0}} \left(\:{t}\:\right){dt} \\ $$$$\:\:\:\:\:{where}\::\:\:{j}_{\left({v}\right)} \left({x}\right)={x}^{{v}} \underset{{n}=\mathrm{0}} {\overset{\:\infty} {\sum}}\frac{\left(−\mathrm{1}\right)^{{n}} {x}^{\mathrm{2}{n}} }{\mathrm{2}^{\mathrm{2}{n}+{v}} {n}!\Gamma\left({n}+{v}+\mathrm{1}\right)}\: \\ $$$$\:\:\:\:\:\:\:\:\::::\:{Bessel}\:{function}\:{of}\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{the}\:{first}\:{type}\:{of}\:{order}\:{v}\:...\: \\ $$$$\:\:\:\:\:\:\:\:\:{j}_{{v}} \left({x}\right)\:{is}\:{convergent}\:\left({why}?\right):\:\forall{x}\in\mathbb{R}... \\ $$
Commented by Dwaipayan Shikari last updated on 20/Dec/20

$$\frac{\mathrm{1}}{\mathrm{2}} \\ $$
