
Question Number 125577 by ajfour last updated on 12/Dec/20
Commented by ajfour last updated on 12/Dec/20
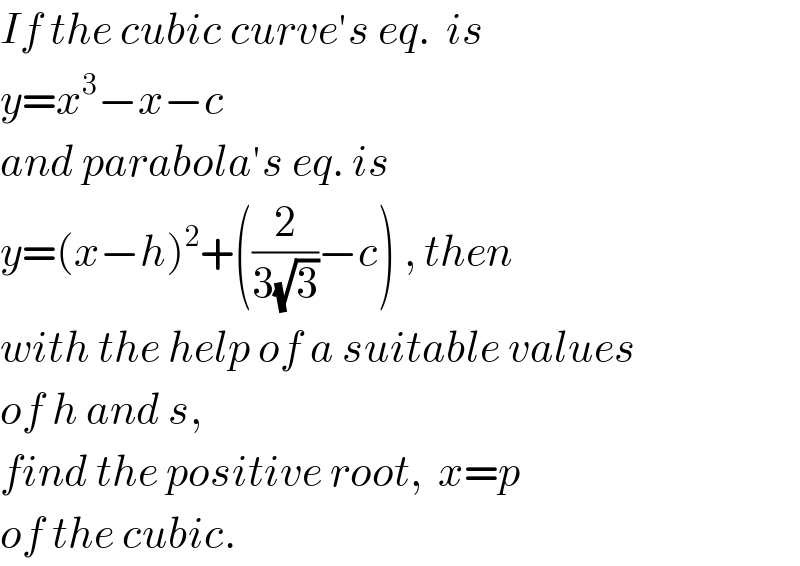
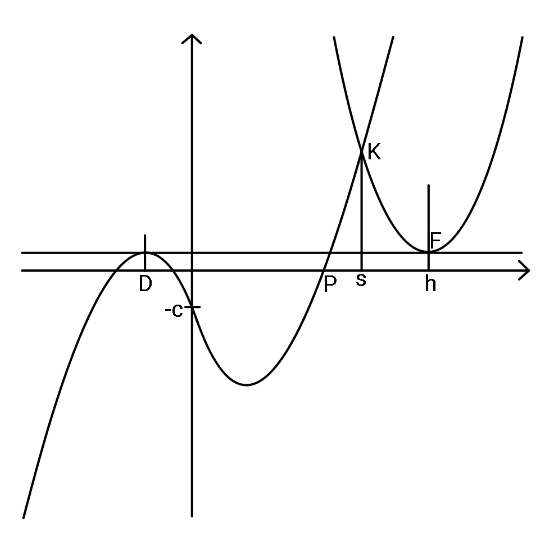
$${If}\:{the}\:{cubic}\:{curve}'{s}\:{eq}.\:\:{is} \\ $$$${y}={x}^{\mathrm{3}} −{x}−{c} \\ $$$${and}\:{parabola}'{s}\:{eq}.\:{is}\: \\ $$$${y}=\left({x}−{h}\right)^{\mathrm{2}} +\left(\frac{\mathrm{2}}{\mathrm{3}\sqrt{\mathrm{3}}}−{c}\right)\:,\:{then} \\ $$$${with}\:{the}\:{help}\:{of}\:{a}\:{suitable}\:{values} \\ $$$${of}\:{h}\:{and}\:{s}, \\ $$$${find}\:{the}\:{positive}\:{root},\:\:{x}={p} \\ $$$${of}\:{the}\:{cubic}. \\ $$
Answered by ajfour last updated on 12/Dec/20

$${y}={x}^{\mathrm{3}} −{x}−{c} \\ $$$${y}=\left({x}−{h}\right)^{\mathrm{2}} +{k}\:\: \\ $$$${p}^{\mathrm{3}} ={p}+{c}\:\:..\left({i}\right) \\ $$$$\left({s}−{h}\right)^{\mathrm{2}} +{k}={s}^{\mathrm{3}} −{s}−{c} \\ $$$${let}\:\:{s}={p}+{m}\:\:\Rightarrow \\ $$$${p}^{\mathrm{3}} +\mathrm{3}{mp}^{\mathrm{2}} +\mathrm{3}{m}^{\mathrm{2}} {p}+{m}^{\mathrm{3}} −{p}−{m}−{c}−{k} \\ $$$$\:−{p}^{\mathrm{2}} −\mathrm{2}{mp}−{m}^{\mathrm{2}} +\mathrm{2}{hp}+\mathrm{2}{mh}−{h}^{\mathrm{2}} =\mathrm{0} \\ $$$$\:\underset{−} {{let}\:\:\mathrm{3}{m}=\mathrm{1}}\:\:\Rightarrow \\ $$$${p}\left(\mathrm{2}{h}−\frac{\mathrm{1}}{\mathrm{3}}\right)−\frac{\mathrm{8}}{\mathrm{27}}−{k}−\left({h}−\frac{\mathrm{1}}{\mathrm{3}}\right)^{\mathrm{2}} =\mathrm{0} \\ $$$$\Rightarrow\:\:{p}=\frac{\left({h}−\frac{\mathrm{1}}{\mathrm{3}}\right)^{\mathrm{2}} +{k}+\frac{\mathrm{8}}{\mathrm{27}}}{\mathrm{2}{h}−\frac{\mathrm{1}}{\mathrm{3}}} \\ $$$${but}\:\:\:{p}^{\mathrm{3}} ={p}+{c}\:\:\:\Rightarrow \\ $$$$\left\{\left({h}−\frac{\mathrm{1}}{\mathrm{3}}\right)^{\mathrm{2}} +{k}+\frac{\mathrm{8}}{\mathrm{27}}\right\}^{\mathrm{3}} \\ $$$$\:\:=\left\{\left({h}−\frac{\mathrm{1}}{\mathrm{3}}\right)^{\mathrm{2}} +{k}+\frac{\mathrm{8}}{\mathrm{27}}\right\}\left(\mathrm{2}{h}−\frac{\mathrm{1}}{\mathrm{3}}\right)^{\mathrm{2}} \\ $$$$\:\:\:\:\:\:\:\:+{c}\left(\mathrm{2}{h}−\frac{\mathrm{1}}{\mathrm{3}}\right)^{\mathrm{3}} \\ $$$${let}\:\:{k}=\frac{\mathrm{2}{h}}{\mathrm{3}} \\ $$$$\:\:\left({h}^{\mathrm{2}} +\frac{\mathrm{11}}{\mathrm{27}}\right)^{\mathrm{3}} =\left({h}^{\mathrm{2}} +\frac{\mathrm{11}}{\mathrm{27}}\right)\left(\mathrm{2}{h}−\frac{\mathrm{1}}{\mathrm{3}}\right)^{\mathrm{2}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:+{c}\left(\mathrm{2}{h}−\frac{\mathrm{1}}{\mathrm{3}}\right)^{\mathrm{3}} \\ $$$$\:\left({h}^{\mathrm{2}} +\frac{\mathrm{11}}{\mathrm{27}}\right)\left({h}^{\mathrm{4}} +\frac{\mathrm{22}{h}^{\mathrm{2}} }{\mathrm{27}}+\frac{\mathrm{121}}{\mathrm{729}}−\mathrm{4}{h}^{\mathrm{2}} +\frac{\mathrm{4}{h}}{\mathrm{3}}−\frac{\mathrm{1}}{\mathrm{9}}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:={c}\left(\mathrm{2}{h}−\frac{\mathrm{1}}{\mathrm{3}}\right)^{\mathrm{3}} \\ $$$$..... \\ $$
