
Question Number 124557 by bemath last updated on 04/Dec/20
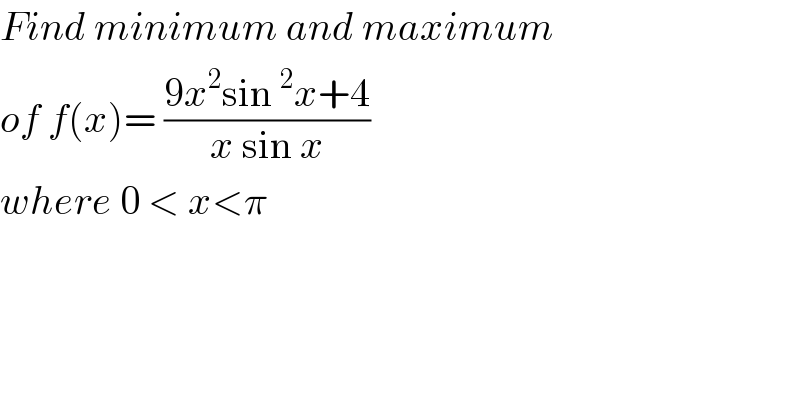
$${Find}\:{minimum}\:{and}\:{maximum} \\ $$ $${of}\:{f}\left({x}\right)=\:\frac{\mathrm{9}{x}^{\mathrm{2}} \mathrm{sin}\:^{\mathrm{2}} {x}+\mathrm{4}}{{x}\:\mathrm{sin}\:{x}} \\ $$ $${where}\:\mathrm{0}\:<\:{x}<\pi \\ $$
Answered by mr W last updated on 04/Dec/20
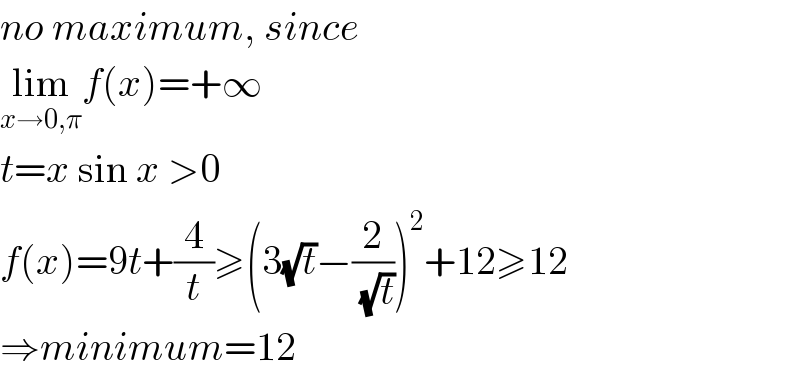
$${no}\:{maximum},\:{since} \\ $$ $$\underset{{x}\rightarrow\mathrm{0},\pi} {\mathrm{lim}}{f}\left({x}\right)=+\infty \\ $$ $${t}={x}\:\mathrm{sin}\:{x}\:>\mathrm{0} \\ $$ $${f}\left({x}\right)=\mathrm{9}{t}+\frac{\mathrm{4}}{{t}}\geqslant\left(\mathrm{3}\sqrt{{t}}−\frac{\mathrm{2}}{\:\sqrt{{t}}}\right)^{\mathrm{2}} +\mathrm{12}\geqslant\mathrm{12} \\ $$ $$\Rightarrow{minimum}=\mathrm{12} \\ $$
Commented bybemath last updated on 04/Dec/20

$${sir}\:{i}\:{got}\:{max}\:=\:\mathrm{18} \\ $$
Commented bybemath last updated on 04/Dec/20
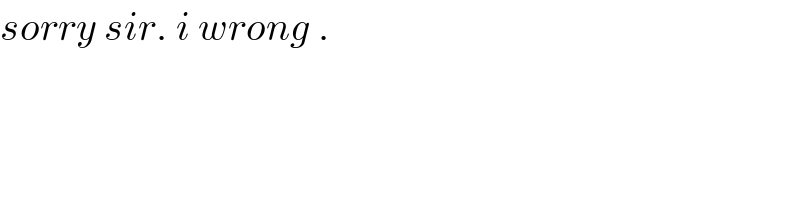
$${sorry}\:{sir}.\:{i}\:{wrong}\:. \\ $$
Commented bymr W last updated on 04/Dec/20
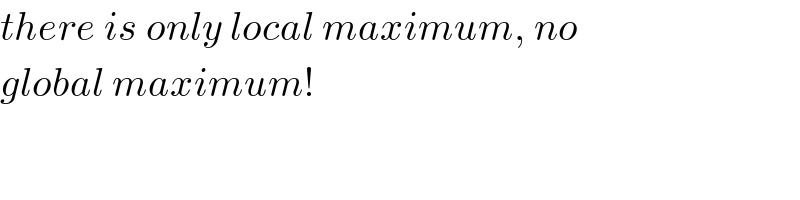
$${there}\:{is}\:{only}\:{local}\:{maximum},\:{no} \\ $$ $${global}\:{maximum}! \\ $$
