
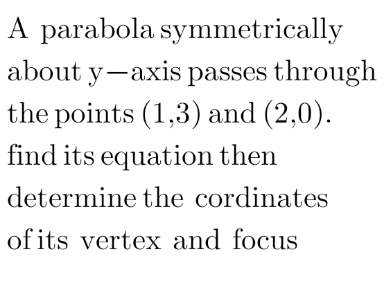
Question Number 123302 by peter frank last updated on 24/Nov/20
Answered by MJS_new last updated on 24/Nov/20
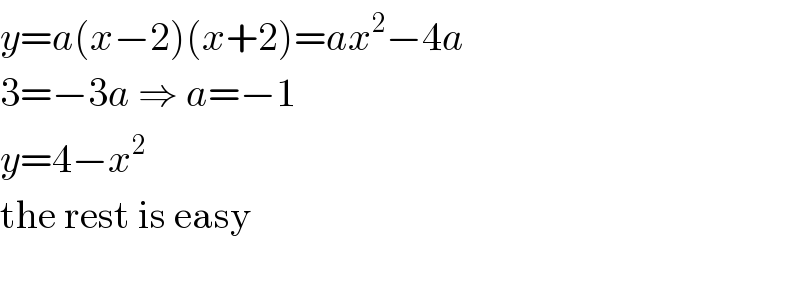
$${y}={a}\left({x}−\mathrm{2}\right)\left({x}+\mathrm{2}\right)={ax}^{\mathrm{2}} −\mathrm{4}{a} \\ $$$$\mathrm{3}=−\mathrm{3}{a}\:\Rightarrow\:{a}=−\mathrm{1} \\ $$$${y}=\mathrm{4}−{x}^{\mathrm{2}} \\ $$$$\mathrm{the}\:\mathrm{rest}\:\mathrm{is}\:\mathrm{easy} \\ $$$$ \\ $$
Commented by peter frank last updated on 24/Nov/20
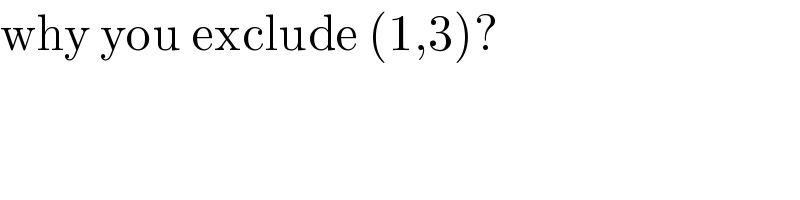
$$\mathrm{why}\:\mathrm{you}\:\mathrm{exclude}\:\left(\mathrm{1},\mathrm{3}\right)? \\ $$
Commented by MJS_new last updated on 24/Nov/20
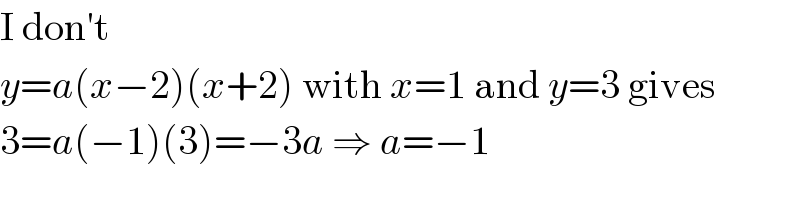
$$\mathrm{I}\:\mathrm{don}'\mathrm{t} \\ $$$${y}={a}\left({x}−\mathrm{2}\right)\left({x}+\mathrm{2}\right)\:\mathrm{with}\:{x}=\mathrm{1}\:\mathrm{and}\:{y}=\mathrm{3}\:\mathrm{gives} \\ $$$$\mathrm{3}={a}\left(−\mathrm{1}\right)\left(\mathrm{3}\right)=−\mathrm{3}{a}\:\Rightarrow\:{a}=−\mathrm{1} \\ $$
Commented by peter frank last updated on 24/Nov/20

$$\mathrm{sorry}\:\mathrm{sir}\:\mathrm{explain}\:\mathrm{first}\:\mathrm{line} \\ $$
Commented by MJS_new last updated on 24/Nov/20
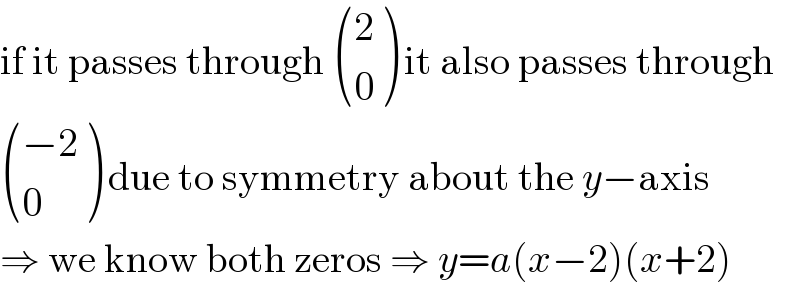
$$\mathrm{if}\:\mathrm{it}\:\mathrm{passes}\:\mathrm{through}\:\begin{pmatrix}{\mathrm{2}}\\{\mathrm{0}}\end{pmatrix}\:\mathrm{it}\:\mathrm{also}\:\mathrm{passes}\:\mathrm{through} \\ $$$$\begin{pmatrix}{−\mathrm{2}}\\{\mathrm{0}}\end{pmatrix}\:\mathrm{due}\:\mathrm{to}\:\mathrm{symmetry}\:\mathrm{about}\:\mathrm{the}\:{y}−\mathrm{axis} \\ $$$$\Rightarrow\:\mathrm{we}\:\mathrm{know}\:\mathrm{both}\:\mathrm{zeros}\:\Rightarrow\:{y}={a}\left({x}−\mathrm{2}\right)\left({x}+\mathrm{2}\right) \\ $$
Answered by MJS_new last updated on 24/Nov/20
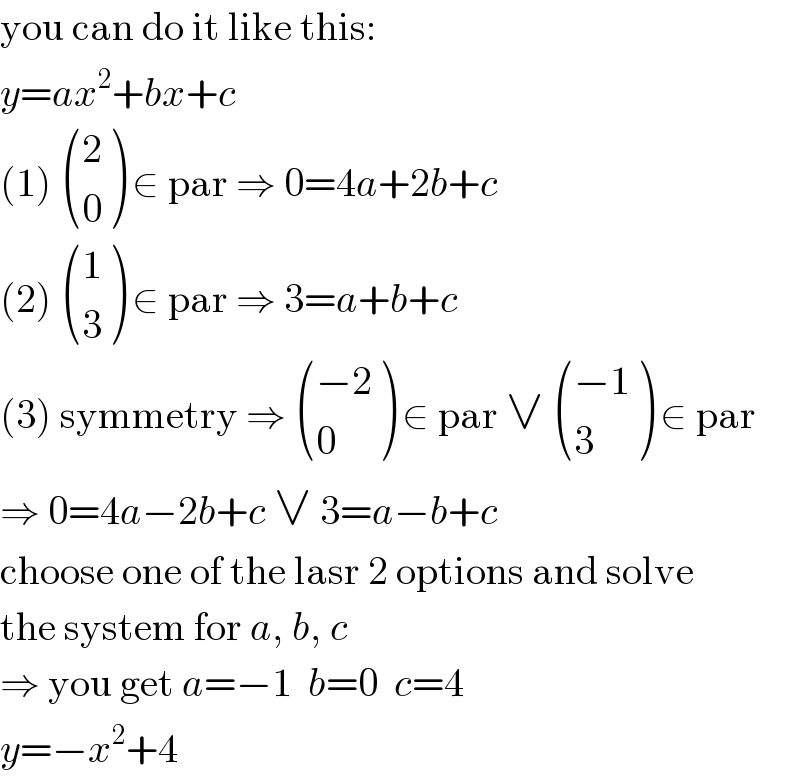
$$\mathrm{you}\:\mathrm{can}\:\mathrm{do}\:\mathrm{it}\:\mathrm{like}\:\mathrm{this}: \\ $$$${y}={ax}^{\mathrm{2}} +{bx}+{c} \\ $$$$\left(\mathrm{1}\right)\:\begin{pmatrix}{\mathrm{2}}\\{\mathrm{0}}\end{pmatrix}\:\in\:\mathrm{par}\:\Rightarrow\:\mathrm{0}=\mathrm{4}{a}+\mathrm{2}{b}+{c} \\ $$$$\left(\mathrm{2}\right)\:\begin{pmatrix}{\mathrm{1}}\\{\mathrm{3}}\end{pmatrix}\:\in\:\mathrm{par}\:\Rightarrow\:\mathrm{3}={a}+{b}+{c} \\ $$$$\left(\mathrm{3}\right)\:\mathrm{symmetry}\:\Rightarrow\:\begin{pmatrix}{−\mathrm{2}}\\{\mathrm{0}}\end{pmatrix}\:\in\:\mathrm{par}\:\vee\:\begin{pmatrix}{−\mathrm{1}}\\{\mathrm{3}}\end{pmatrix}\:\in\:\mathrm{par} \\ $$$$\Rightarrow\:\mathrm{0}=\mathrm{4}{a}−\mathrm{2}{b}+{c}\:\vee\:\mathrm{3}={a}−{b}+{c} \\ $$$$\mathrm{choose}\:\mathrm{one}\:\mathrm{of}\:\mathrm{the}\:\mathrm{lasr}\:\mathrm{2}\:\mathrm{options}\:\mathrm{and}\:\mathrm{solve} \\ $$$$\mathrm{the}\:\mathrm{system}\:\mathrm{for}\:{a},\:{b},\:{c} \\ $$$$\Rightarrow\:\mathrm{you}\:\mathrm{get}\:{a}=−\mathrm{1}\:\:{b}=\mathrm{0}\:\:{c}=\mathrm{4} \\ $$$${y}=−{x}^{\mathrm{2}} +\mathrm{4} \\ $$
