
Question Number 121337 by ajfour last updated on 06/Nov/20
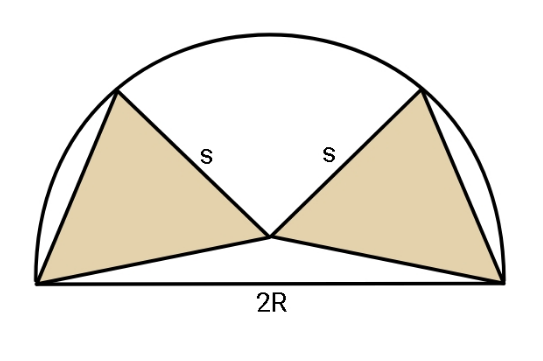
Commented by ajfour last updated on 06/Nov/20

$${Find}\:{ratio}\:\:\frac{{s}}{{R}}\:,\:{where}\:{s}\:{is}\:{side} \\ $$$${lengths}\:{of}\:{the}\:{two}\:{equilateral} \\ $$$${triangles}\:{and}\:{R}\:{the}\:{radius}\:{of}\: \\ $$$${the}\:{semicircle}. \\ $$
Commented by MJS_new last updated on 06/Nov/20

$$\mathrm{the}\:\mathrm{common}\:\mathrm{vertex}\:\mathrm{of}\:\mathrm{the}\:\mathrm{triangles}\:\mathrm{is}\:\mathrm{the} \\ $$$$\mathrm{center}\:\mathrm{of}\:\mathrm{the}\:\mathrm{circle}\:\Rightarrow\:{s}\:\mathrm{is}\:\mathrm{the}\:\mathrm{radius}\:\mathrm{of}\:\mathrm{this} \\ $$$$\mathrm{circle}\:\Rightarrow\:\mathrm{2}{R}\leqslant\mathrm{2}{s}\:...\:\mathrm{please}\:\mathrm{check}\:\mathrm{it}! \\ $$
Commented by ajfour last updated on 07/Nov/20
$${yes}\:{sir},\:\:{very}\:{silly}\:{question},\:{pardon}\:{me}. \\ $$
