
Question Number 119138 by 675480065 last updated on 22/Oct/20
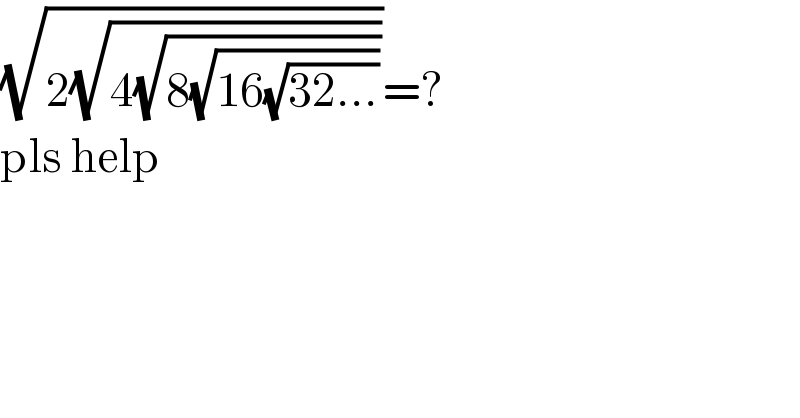
$$\sqrt{\mathrm{2}\sqrt{\mathrm{4}\sqrt{\mathrm{8}\sqrt{\mathrm{16}\sqrt{\mathrm{32}...}}}}}=? \\ $$$$\mathrm{pls}\:\mathrm{help} \\ $$
Commented by Dwaipayan Shikari last updated on 22/Oct/20
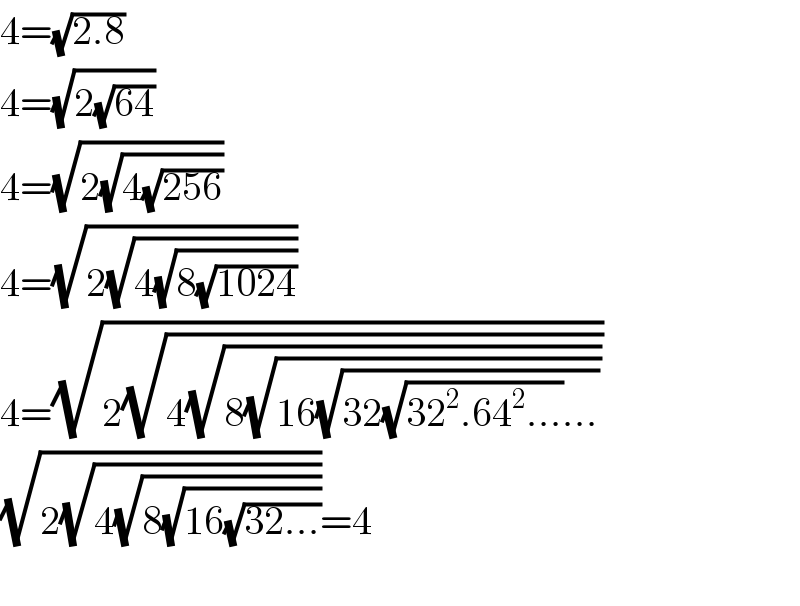
$$\mathrm{4}=\sqrt{\mathrm{2}.\mathrm{8}} \\ $$$$\mathrm{4}=\sqrt{\mathrm{2}\sqrt{\mathrm{64}}} \\ $$$$\mathrm{4}=\sqrt{\mathrm{2}\sqrt{\mathrm{4}\sqrt{\mathrm{256}}}} \\ $$$$\mathrm{4}=\sqrt{\mathrm{2}\sqrt{\mathrm{4}\sqrt{\mathrm{8}\sqrt{\mathrm{1024}}}}} \\ $$$$\mathrm{4}=\sqrt{\mathrm{2}\sqrt{\mathrm{4}\sqrt{\mathrm{8}\sqrt{\mathrm{16}\sqrt{\mathrm{32}\sqrt{\mathrm{32}^{\mathrm{2}} .\mathrm{64}^{\mathrm{2}} ...}...}}}}} \\ $$$$\sqrt{\mathrm{2}\sqrt{\mathrm{4}\sqrt{\mathrm{8}\sqrt{\mathrm{16}\sqrt{\mathrm{32}...}}}}}=\mathrm{4} \\ $$$$ \\ $$
Answered by PRITHWISH SEN 2 last updated on 22/Oct/20
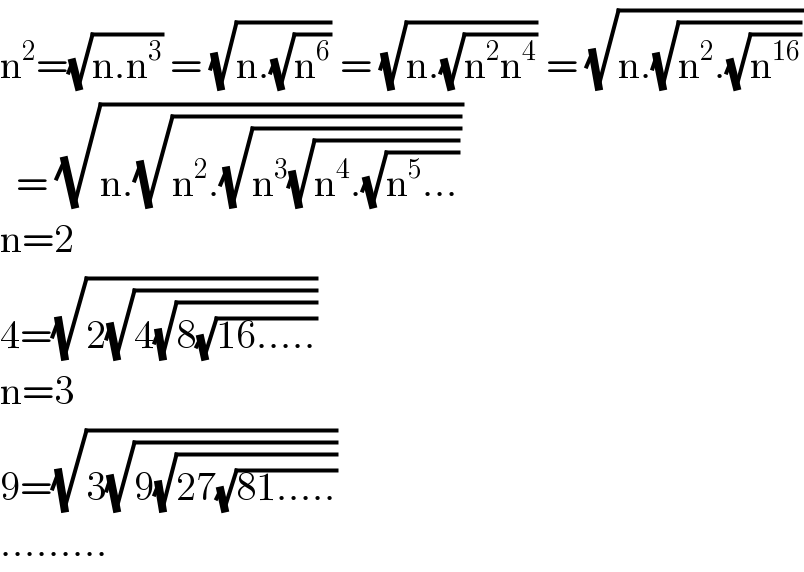
$$\mathrm{n}^{\mathrm{2}} =\sqrt{\mathrm{n}.\mathrm{n}^{\mathrm{3}} }\:=\:\sqrt{\mathrm{n}.\sqrt{\mathrm{n}^{\mathrm{6}} }}\:=\:\sqrt{\mathrm{n}.\sqrt{\mathrm{n}^{\mathrm{2}} \mathrm{n}^{\mathrm{4}} }}\:=\:\sqrt{\mathrm{n}.\sqrt{\mathrm{n}^{\mathrm{2}} .\sqrt{\mathrm{n}^{\mathrm{16}} }}} \\ $$$$\:\:=\:\sqrt{\mathrm{n}.\sqrt{\mathrm{n}^{\mathrm{2}} .\sqrt{\mathrm{n}^{\mathrm{3}} \sqrt{\mathrm{n}^{\mathrm{4}} .\sqrt{\mathrm{n}^{\mathrm{5}} ...}}}}}\: \\ $$$$\mathrm{n}=\mathrm{2} \\ $$$$\mathrm{4}=\sqrt{\mathrm{2}\sqrt{\mathrm{4}\sqrt{\mathrm{8}\sqrt{\mathrm{16}.....}}}} \\ $$$$\mathrm{n}=\mathrm{3} \\ $$$$\mathrm{9}=\sqrt{\mathrm{3}\sqrt{\mathrm{9}\sqrt{\mathrm{27}\sqrt{\mathrm{81}.....}}}} \\ $$$$......... \\ $$
