
Previous in Permutation and Combination Next in Permutation and Combination
Question Number 118694 by benjo_mathlover last updated on 19/Oct/20

$${How}\:{many}\:{sets}\:{of}\:\mathrm{3}\:{numbers}\:{each}\:{can} \\ $$$${be}\:{formed}\:{from}\:{the}\:{numbers}\:\left\{\:\mathrm{1},\mathrm{2},\mathrm{3},...,\mathrm{20}\:\right\}\:{if}\:{no} \\ $$$${two}\:{consecutive}\:{numbers}\:{are}\:{to}\:{be}\:{in}\:{a}\:{set}\:? \\ $$
Answered by bemath last updated on 19/Oct/20
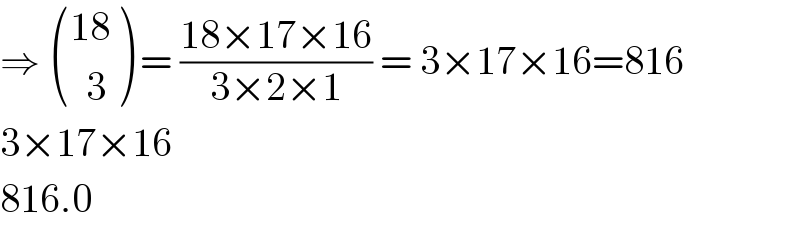
$$\Rightarrow\:\begin{pmatrix}{\mathrm{18}}\\{\:\:\mathrm{3}}\end{pmatrix}\:=\:\frac{\mathrm{18}×\mathrm{17}×\mathrm{16}}{\mathrm{3}×\mathrm{2}×\mathrm{1}}\:=\:\mathrm{3}×\mathrm{17}×\mathrm{16}=\mathrm{816} \\ $$$$\mathrm{3}×\mathrm{17}×\mathrm{16} \\ $$$$\mathrm{816}.\mathrm{0} \\ $$
Commented by benjo_mathlover last updated on 19/Oct/20

$${yes}\:{correct} \\ $$
Commented by PRITHWISH SEN 2 last updated on 19/Oct/20
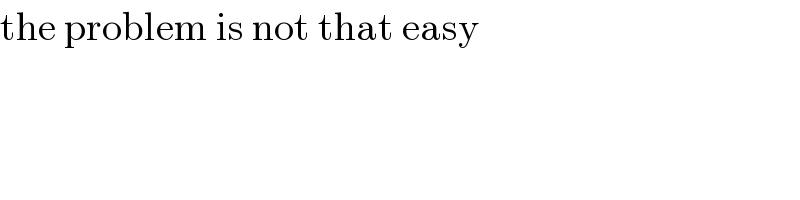
$$\mathrm{the}\:\mathrm{problem}\:\mathrm{is}\:\mathrm{not}\:\mathrm{that}\:\mathrm{easy} \\ $$
Commented by PRITHWISH SEN 2 last updated on 19/Oct/20
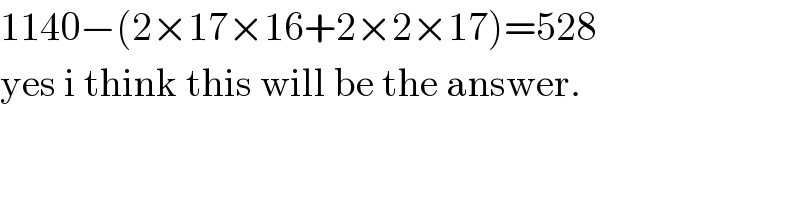
$$\mathrm{1140}−\left(\mathrm{2}×\mathrm{17}×\mathrm{16}+\mathrm{2}×\mathrm{2}×\mathrm{17}\right)=\mathrm{528}\: \\ $$$$\mathrm{yes}\:\mathrm{i}\:\mathrm{think}\:\mathrm{this}\:\mathrm{will}\:\mathrm{be}\:\mathrm{the}\:\mathrm{answer}. \\ $$
Answered by mr W last updated on 19/Oct/20

$${total}:\:{C}_{\mathrm{3}} ^{\mathrm{20}} \\ $$$$ \\ $$$${three}\:{consecutive}\:{numbers}:\: \\ $$$$\mathrm{1}+\mathrm{2}+\mathrm{3},\:\mathrm{2}+\mathrm{3}+\mathrm{4},...,\mathrm{18}+\mathrm{19}+\mathrm{20} \\ $$$$\Rightarrow\mathrm{18} \\ $$$$ \\ $$$${two}\:{consecutive}\:{numbers}:\: \\ $$$$\mathrm{1}+\mathrm{2}:\:\mathrm{17} \\ $$$$\mathrm{2}+\mathrm{3}:\:\mathrm{16} \\ $$$$\mathrm{3}+\mathrm{4}:\:\mathrm{16} \\ $$$$... \\ $$$$\mathrm{18}+\mathrm{19}:\:\mathrm{16} \\ $$$$\mathrm{19}+\mathrm{20}:\:\mathrm{17} \\ $$$$\Rightarrow\mathrm{2}×\mathrm{17}+\mathrm{17}×\mathrm{16}=\mathrm{17}×\mathrm{18} \\ $$$$ \\ $$$${totally}: \\ $$$${C}_{\mathrm{3}} ^{\mathrm{20}} −\mathrm{18}−\mathrm{17}×\mathrm{18}=\mathrm{816} \\ $$
Commented by benjo_mathlover last updated on 20/Oct/20

$${yes}... \\ $$
Commented by PRITHWISH SEN 2 last updated on 20/Oct/20
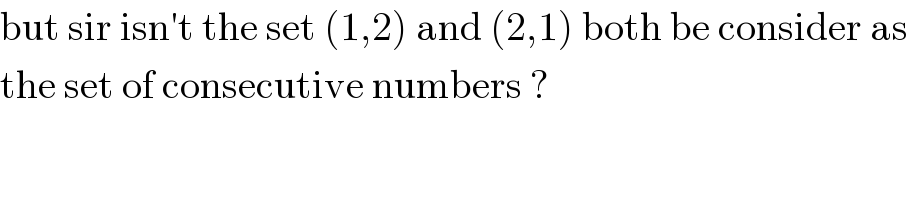
$$\mathrm{but}\:\mathrm{sir}\:\mathrm{isn}'\mathrm{t}\:\mathrm{the}\:\mathrm{set}\:\left(\mathrm{1},\mathrm{2}\right)\:\mathrm{and}\:\left(\mathrm{2},\mathrm{1}\right)\:\mathrm{both}\:\mathrm{be}\:\mathrm{consider}\:\mathrm{as} \\ $$$$\mathrm{the}\:\mathrm{set}\:\mathrm{of}\:\mathrm{consecutive}\:\mathrm{numbers}\:? \\ $$
Commented by mr W last updated on 20/Oct/20
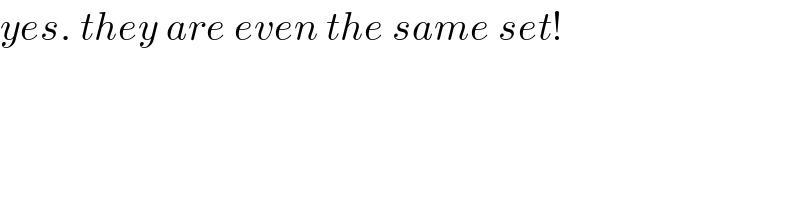
$${yes}.\:{they}\:{are}\:{even}\:{the}\:{same}\:{set}! \\ $$
