
Question Number 117781 by hmh2k20 last updated on 13/Oct/20
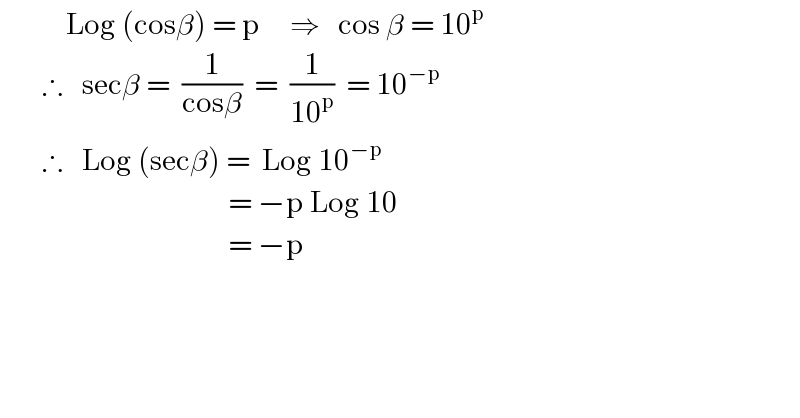
$$\:\:\:\:\:\:\:\:\:\:\:\mathrm{Log}\:\left(\mathrm{cos}\beta\right)\:=\:\mathrm{p}\:\:\:\:\:\Rightarrow\:\:\:\mathrm{cos}\:\beta\:=\:\mathrm{10}^{\mathrm{p}} \:\: \\ $$$$\:\:\:\:\:\:\:\therefore\:\:\:\mathrm{sec}\beta\:=\:\:\frac{\mathrm{1}}{\mathrm{cos}\beta}\:\:=\:\:\frac{\mathrm{1}}{\mathrm{10}^{\mathrm{p}} }\:\:=\:\mathrm{10}^{−\mathrm{p}} \\ $$$$\:\:\:\:\:\:\:\therefore\:\:\:\mathrm{Log}\:\left(\mathrm{sec}\beta\right)\:=\:\:\mathrm{Log}\:\mathrm{10}^{−\mathrm{p}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\:−\mathrm{p}\:\mathrm{Log}\:\mathrm{10} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\:−\mathrm{p} \\ $$
Answered by $@y@m last updated on 13/Oct/20

$${Kindly}\:{use}\:{editor}\:{option}\:{of}\:{the}\:{app} \\ $$$${for}\:{rough}\:{work}. \\ $$$${Just}\:{tap}\:{on}\:\equiv\:{sign}\:{on}\:{top}\:{left}\:{side} \\ $$$${and}\:{then}\:{tap}\:{on}\:\mathrm{editor}. \\ $$$${This}\:{section}\:{is}\:{meant}\:{for}\:{mutual} \\ $$$${discussion}. \\ $$
Commented by Tinku Tara last updated on 13/Oct/20

$$\mathrm{Latest}\:\mathrm{updated}\:\mathrm{app}\:\mathrm{opens}\:\mathrm{forum} \\ $$$$\mathrm{be}\:\mathrm{default}\:\mathrm{and}\:\mathrm{editor}\:\mathrm{is}\:\mathrm{opened} \\ $$$$\mathrm{from}\:\mathrm{navigation}\:\mathrm{window}.\: \\ $$$$\mathrm{Most}\:\mathrm{of}\:\mathrm{users}\:\mathrm{are}\:\mathrm{using}\:\mathrm{the}\:\mathrm{app} \\ $$$$\mathrm{only}\:\mathrm{to}\:\mathrm{navigate}\:\mathrm{forum}\:\mathrm{and}\:\mathrm{a} \\ $$$$\mathrm{few}\:\mathrm{actually}\:\mathrm{create}\:\mathrm{user}\:\mathrm{id}.\:\mathrm{This} \\ $$$$\mathrm{interface}\:\mathrm{simplfies}\:\mathrm{browsing}\:\mathrm{for} \\ $$$$\mathrm{majority}\:\mathrm{of}\:\mathrm{users}. \\ $$$$\mathrm{Only}\:\mathrm{a}\:\mathrm{small}\:\mathrm{set}\:\mathrm{of}\:\mathrm{users}\:\mathrm{are}\:\mathrm{actually} \\ $$$$\mathrm{using}\:\mathrm{offline}\:\mathrm{editor}. \\ $$
Commented by $@y@m last updated on 13/Oct/20

$${You}\:{are}\:{right}. \\ $$$${Offline}\:{editor}\:{is}\:{used}\:{rarely}. \\ $$$${So}\:{I}\:{appreciate}\:{new}\:{update}. \\ $$
