
Previous in Differential Equation Next in Differential Equation
Question Number 116425 by bemath last updated on 04/Oct/20
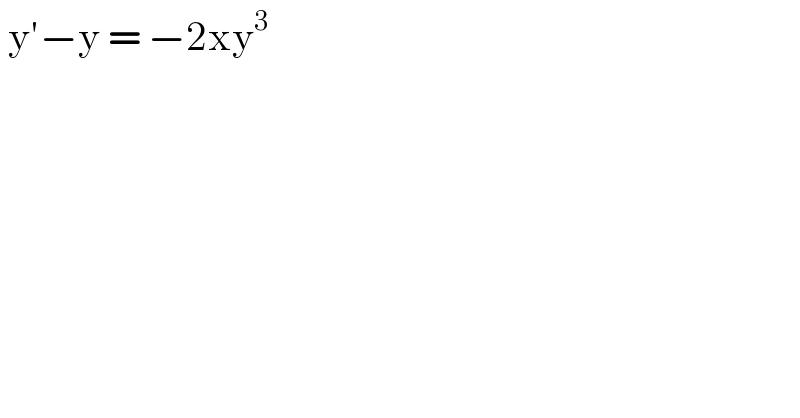
$$\:\mathrm{y}'−\mathrm{y}\:=\:−\mathrm{2xy}^{\mathrm{3}} \\ $$
Commented by bemath last updated on 04/Oct/20
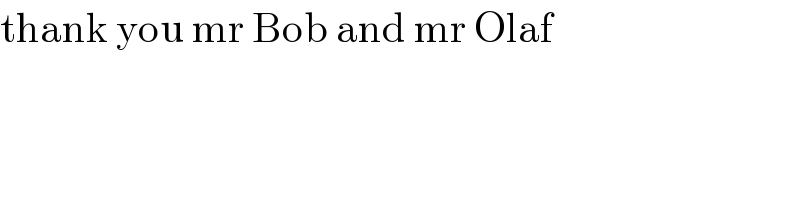
$$\mathrm{thank}\:\mathrm{you}\:\mathrm{mr}\:\mathrm{Bob}\:\mathrm{and}\:\mathrm{mr}\:\mathrm{Olaf} \\ $$
Answered by bobhans last updated on 04/Oct/20
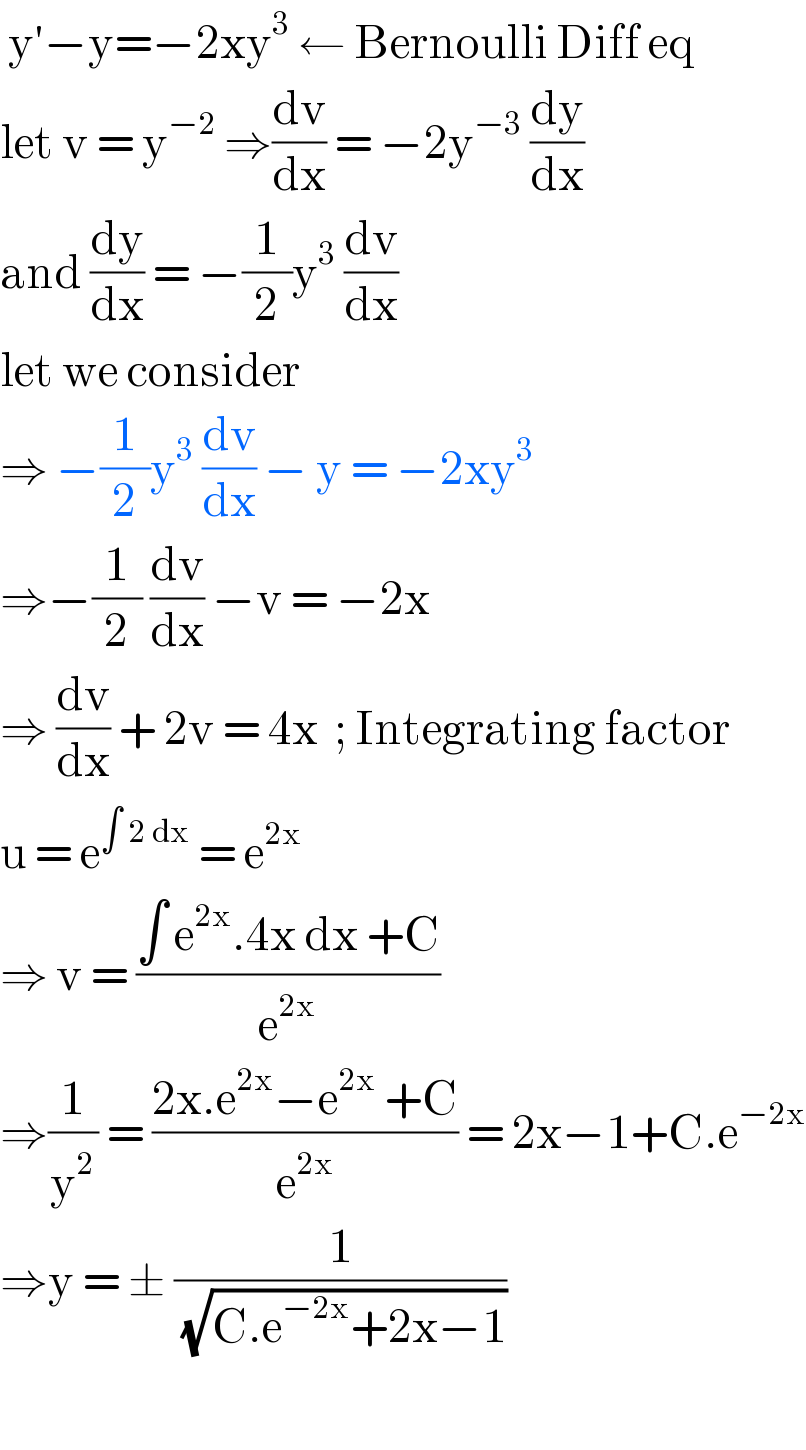
$$\:\mathrm{y}'−\mathrm{y}=−\mathrm{2xy}^{\mathrm{3}} \:\leftarrow\:\mathrm{Bernoulli}\:\mathrm{Diff}\:\mathrm{eq} \\ $$$$\mathrm{let}\:\mathrm{v}\:=\:\mathrm{y}^{−\mathrm{2}} \:\Rightarrow\frac{\mathrm{dv}}{\mathrm{dx}}\:=\:−\mathrm{2y}^{−\mathrm{3}} \:\frac{\mathrm{dy}}{\mathrm{dx}} \\ $$$$\mathrm{and}\:\frac{\mathrm{dy}}{\mathrm{dx}}\:=\:−\frac{\mathrm{1}}{\mathrm{2}}\mathrm{y}^{\mathrm{3}} \:\frac{\mathrm{dv}}{\mathrm{dx}} \\ $$$$\mathrm{let}\:\mathrm{we}\:\mathrm{consider}\: \\ $$$$\Rightarrow\:−\frac{\mathrm{1}}{\mathrm{2}}\mathrm{y}^{\mathrm{3}} \:\frac{\mathrm{dv}}{\mathrm{dx}}\:−\:\mathrm{y}\:=\:−\mathrm{2xy}^{\mathrm{3}} \: \\ $$$$\Rightarrow−\frac{\mathrm{1}}{\mathrm{2}}\:\frac{\mathrm{dv}}{\mathrm{dx}}\:−\mathrm{v}\:=\:−\mathrm{2x} \\ $$$$\Rightarrow\:\frac{\mathrm{dv}}{\mathrm{dx}}\:+\:\mathrm{2v}\:=\:\mathrm{4x}\:\:;\:\mathrm{Integrating}\:\mathrm{factor} \\ $$$$\mathrm{u}\:=\:\mathrm{e}^{\int\:\mathrm{2}\:\mathrm{dx}} \:=\:\mathrm{e}^{\mathrm{2x}} \: \\ $$$$\Rightarrow\:\mathrm{v}\:=\:\frac{\int\:\mathrm{e}^{\mathrm{2x}} .\mathrm{4x}\:\mathrm{dx}\:+\mathrm{C}}{\mathrm{e}^{\mathrm{2x}} }\: \\ $$$$\Rightarrow\frac{\mathrm{1}}{\mathrm{y}^{\mathrm{2}} }\:=\:\frac{\mathrm{2x}.\mathrm{e}^{\mathrm{2x}} −\mathrm{e}^{\mathrm{2x}} \:+\mathrm{C}}{\mathrm{e}^{\mathrm{2x}} }\:=\:\mathrm{2x}−\mathrm{1}+\mathrm{C}.\mathrm{e}^{−\mathrm{2x}} \\ $$$$\Rightarrow\mathrm{y}\:=\:\pm\:\frac{\mathrm{1}}{\:\sqrt{\mathrm{C}.\mathrm{e}^{−\mathrm{2x}} +\mathrm{2x}−\mathrm{1}}} \\ $$$$ \\ $$
Answered by Olaf last updated on 04/Oct/20

$$\mathrm{I}\:\mathrm{suppose}\:\mathrm{that}\:{y}\:\mathrm{is}\:\mathrm{a}\:\mathrm{positive}\:\mathrm{function} \\ $$$$\mathrm{and}\:{y}\:=\:\frac{\mathrm{1}}{\:\sqrt{{u}}} \\ $$$$−\frac{\mathrm{1}}{\mathrm{2}}.\frac{{u}'}{{u}^{\mathrm{3}/\mathrm{2}} }−\frac{\mathrm{1}}{{u}^{\mathrm{1}/\mathrm{2}} }\:=\:−\mathrm{2}{x}\frac{\mathrm{1}}{{u}^{\mathrm{3}/\mathrm{2}} } \\ $$$${u}'+\mathrm{2}{u}\:=\:\mathrm{4}{x} \\ $$$${u}_{\mathrm{0}} \:=\:\mathrm{2}{x}−\mathrm{1}\:\mathrm{is}\:\mathrm{a}\:\mathrm{particular}\:\mathrm{solution}. \\ $$$$\mathrm{Homogen}\:\mathrm{equation}\:: \\ $$$${u}_{\mathrm{H}} '+\mathrm{2}{u}_{\mathrm{H}} \:=\:\mathrm{0} \\ $$$$\frac{{u}_{\mathrm{H}} '}{{u}_{\mathrm{H}} }\:=\:−\mathrm{2} \\ $$$$\mathrm{ln}{u}_{\mathrm{H}} \:=\:−\mathrm{2}{x}+\mathrm{C} \\ $$$${u}_{\mathrm{H}} \:=\:\mathrm{K}{e}^{−\mathrm{2}{x}} \\ $$$${u}\:=\:{u}_{\mathrm{H}} +{u}_{\mathrm{0}} \:=\:\mathrm{K}{e}^{−\mathrm{2}{x}} +\mathrm{2}{x}−\mathrm{1} \\ $$$${y}\:=\:\frac{\mathrm{1}}{\:\sqrt{{u}}}\:=\:\frac{\mathrm{1}}{\:\sqrt{\mathrm{K}{e}^{−\mathrm{2}{x}} +\mathrm{2}{x}−\mathrm{1}}} \\ $$$$\mathrm{Symetrically},\:\mathrm{if}\:{y}\:\mathrm{is}\:\mathrm{a}\:\mathrm{negative}\:\mathrm{function} \\ $$$${y}\:=\:\:−\frac{\mathrm{1}}{\:\sqrt{\mathrm{K}{e}^{−\mathrm{2}{x}} +\mathrm{2}{x}−\mathrm{1}}}\:\mathrm{is}\:\mathrm{a}\:\mathrm{solution}\:\mathrm{too}. \\ $$$$ \\ $$
