
Question Number 115174 by bemath last updated on 24/Sep/20
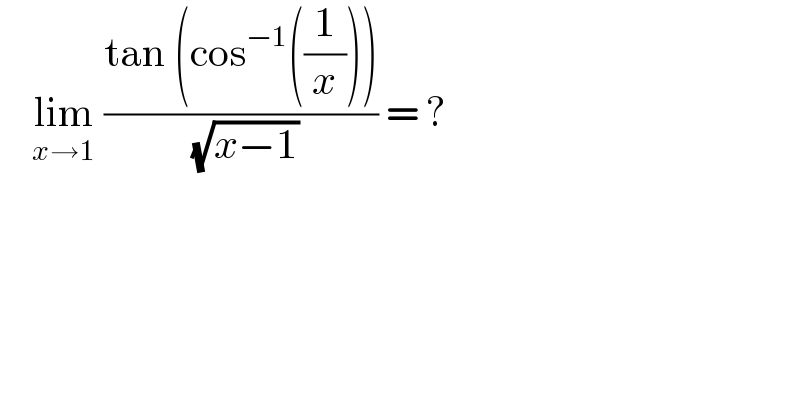
$$\:\:\:\:\underset{{x}\rightarrow\mathrm{1}} {\mathrm{lim}}\:\frac{\mathrm{tan}\:\left(\mathrm{cos}^{−\mathrm{1}} \left(\frac{\mathrm{1}}{{x}}\right)\right)}{\:\sqrt{{x}−\mathrm{1}}}\:=\:? \\ $$
Answered by bobhans last updated on 24/Sep/20
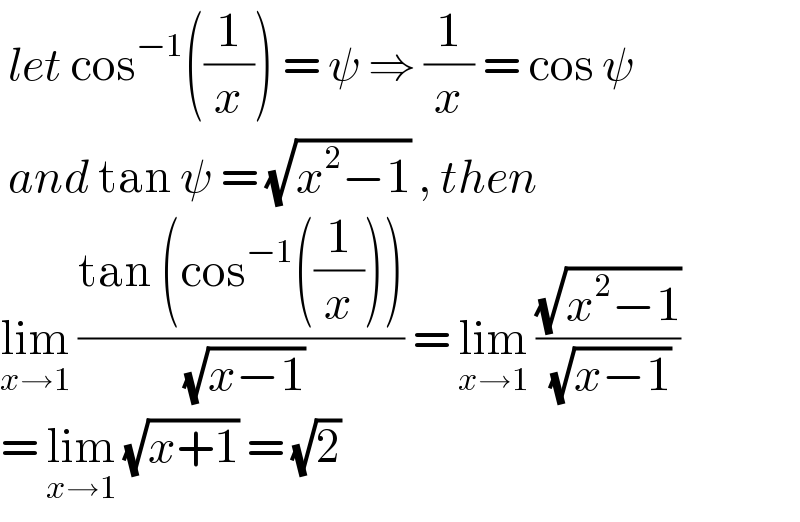
$$\:{let}\:\mathrm{cos}^{−\mathrm{1}} \left(\frac{\mathrm{1}}{{x}}\right)\:=\:\psi\:\Rightarrow\:\frac{\mathrm{1}}{{x}}\:=\:\mathrm{cos}\:\psi\: \\ $$$$\:{and}\:\mathrm{tan}\:\psi\:=\:\sqrt{{x}^{\mathrm{2}} −\mathrm{1}}\:,\:{then}\: \\ $$$$\underset{{x}\rightarrow\mathrm{1}} {\mathrm{lim}}\:\frac{\mathrm{tan}\:\left(\mathrm{cos}^{−\mathrm{1}} \left(\frac{\mathrm{1}}{{x}}\right)\right)}{\:\sqrt{{x}−\mathrm{1}}}\:=\:\underset{{x}\rightarrow\mathrm{1}} {\mathrm{lim}}\:\frac{\sqrt{{x}^{\mathrm{2}} −\mathrm{1}}}{\:\sqrt{{x}−\mathrm{1}}}\: \\ $$$$=\:\underset{{x}\rightarrow\mathrm{1}} {\mathrm{lim}}\:\sqrt{{x}+\mathrm{1}}\:=\:\sqrt{\mathrm{2}}\: \\ $$
Answered by Olaf last updated on 24/Sep/20
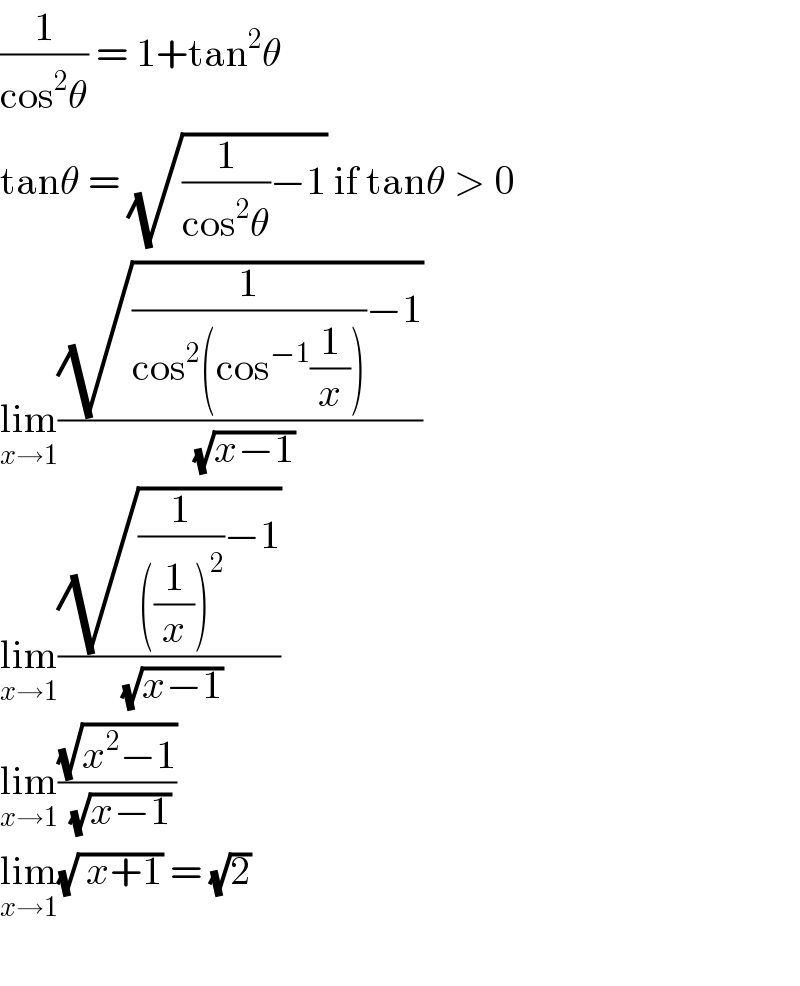
$$\frac{\mathrm{1}}{\mathrm{cos}^{\mathrm{2}} \theta}\:=\:\mathrm{1}+\mathrm{tan}^{\mathrm{2}} \theta \\ $$$$\mathrm{tan}\theta\:=\:\sqrt{\frac{\mathrm{1}}{\mathrm{cos}^{\mathrm{2}} \theta}−\mathrm{1}}\:\mathrm{if}\:\mathrm{tan}\theta\:>\:\mathrm{0} \\ $$$$\underset{{x}\rightarrow\mathrm{1}} {\mathrm{lim}}\frac{\sqrt{\frac{\mathrm{1}}{\mathrm{cos}^{\mathrm{2}} \left(\mathrm{cos}^{−\mathrm{1}} \frac{\mathrm{1}}{{x}}\right)}−\mathrm{1}}}{\:\sqrt{{x}−\mathrm{1}}} \\ $$$$\underset{{x}\rightarrow\mathrm{1}} {\mathrm{lim}}\frac{\sqrt{\frac{\mathrm{1}}{\left(\frac{\mathrm{1}}{{x}}\right)^{\mathrm{2}} }−\mathrm{1}}}{\:\sqrt{{x}−\mathrm{1}}} \\ $$$$\underset{{x}\rightarrow\mathrm{1}} {\mathrm{lim}}\frac{\sqrt{{x}^{\mathrm{2}} −\mathrm{1}}}{\:\sqrt{{x}−\mathrm{1}}} \\ $$$$\underset{{x}\rightarrow\mathrm{1}} {\mathrm{lim}}\sqrt{\:{x}+\mathrm{1}}\:=\:\sqrt{\mathrm{2}} \\ $$$$ \\ $$
