
Question Number 114933 by mathmax by abdo last updated on 22/Sep/20
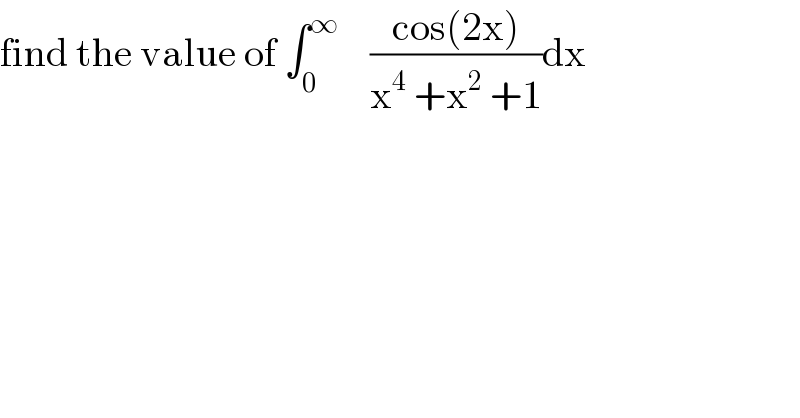
$$\mathrm{find}\:\mathrm{the}\:\mathrm{value}\:\mathrm{of}\:\int_{\mathrm{0}} ^{\infty} \:\:\:\:\frac{\mathrm{cos}\left(\mathrm{2x}\right)}{\mathrm{x}^{\mathrm{4}} \:+\mathrm{x}^{\mathrm{2}} \:+\mathrm{1}}\mathrm{dx} \\ $$
Answered by mathdave last updated on 22/Sep/20
![solution let I=∫_0 ^∞ ((cos(2x))/(1+x^2 +x^4 ))dx=(1/2)∫_(−∞) ^∞ ((cos2x)/(1+x^2 +x^4 ))dx 2I=∫_(−∞) ^∞ ((e^(2ix) +e^(−2ix) )/(1+x^2 +x^4 ))dx=∫_(−∞) ^∞ (e^(2ix) /(1+x^2 +x^4 ))dx let 1+x^2 +x^4 =(x^2 −x+1)(x^2 +x+1) let the root of x^2 −x+1=0 are z_1 =(1/2)+i((√3)/2) and z_2 =(1/2)−i((√3)/2) let the root of x^2 +x+1=0 are z_3 =−(1/2)+i((√3)/2) and z_4 =−(1/2)−i((√3)/2) by using the infinite semicycle on the upperhalf plane as the contour,we have the poles has z_1 =(1/2)+i((√3)/2) and z_3 =−(1/2)+i((√3)/2) then apply residue theorem let f(z)=(e^(2iz) /(1+x^2 +x^4 ))=(e^(2iz) /((z−z_1 )(z−z_2 )(z−z_3 )(z−z_4 ))) by residue theorem Res[f(z):z_1 ]=lim_(z→z_1 ) [(z−z_1 )f(z)]=(e^(2iz_1 ) /((z_1 −z_2 )(z_1 −z_3 )(z_1 −z_4 ))) =(e^(−(√3)+i) /(−3+i(√3)))=−(1/(12))(3+i(√3))e^(−(√3)+i) ......(1) and Res[f(z):z_3 ]=lim_(z→z_3 ) [(z−z_3 )f(z)]=(e^(2iz_3 ) /((z_3 −z_1 )(z_3 −z_2 )(z_3 −z_4 ))) =(e^(−(√3)−i) /(3+i(√3)))=(1/(12))(3−i(√3))e^(−(√3)−i) .........(2) but ∫_R f(z)dz=2πiΣResf(z) 2I=2πi[(1/(12))(3−i(√3))e^(−(√3)−i) −(1/(12))(3+i(√3))e^(−(√3)+i) ] 2I=((πe^(−(√3)) )/6)[(3i+(√3))e^(−i) −(3i−(√3))e^i ] 2I=((πe^(−(√3)) )/6)[−3i(e^i −e^(−i) )+(√3)(e^i +e^(−i) )] 2I=((πe^(−(√3)) )/6)[−((6i×i)/(2i))(e^i −e^(−i) )+((2(√3))/2)(e^i +e^(−i) )] 2I=((πe^(−(√3)) )/6)[6(((e^i −e^(−i) )/(2i)))+2(√3)(((e^i +e^(−i) )/2))] 2I=πe^(−(√3)) (sin(1)+(1/(√3))cos(1)) ∵I=(1/2)πe^(−(√3)) [sin(1)+((√3)/3)cos(1)] ∫_0 ^∞ ((cos(2x))/(1+x^2 +x^4 ))dx=((πe^(−(√3)) )/6)[3sin(1)+(√3)cos(1)] by mathdave(22/09/2020)](Q115007.png)
$${solution} \\ $$$${let} \\ $$$${I}=\int_{\mathrm{0}} ^{\infty} \frac{\mathrm{cos}\left(\mathrm{2}{x}\right)}{\mathrm{1}+{x}^{\mathrm{2}} +{x}^{\mathrm{4}} }{dx}=\frac{\mathrm{1}}{\mathrm{2}}\int_{−\infty} ^{\infty} \frac{\mathrm{cos2}{x}}{\mathrm{1}+{x}^{\mathrm{2}} +{x}^{\mathrm{4}} }{dx} \\ $$$$\mathrm{2}{I}=\int_{−\infty} ^{\infty} \frac{{e}^{\mathrm{2}{ix}} +{e}^{−\mathrm{2}{ix}} }{\mathrm{1}+{x}^{\mathrm{2}} +{x}^{\mathrm{4}} }{dx}=\int_{−\infty} ^{\infty} \frac{{e}^{\mathrm{2}{ix}} }{\mathrm{1}+{x}^{\mathrm{2}} +{x}^{\mathrm{4}} }{dx} \\ $$$${let}\:\mathrm{1}+{x}^{\mathrm{2}} +{x}^{\mathrm{4}} =\left({x}^{\mathrm{2}} −{x}+\mathrm{1}\right)\left({x}^{\mathrm{2}} +{x}+\mathrm{1}\right) \\ $$$${let}\:{the}\:{root}\:{of}\:\:{x}^{\mathrm{2}} −{x}+\mathrm{1}=\mathrm{0}\:{are} \\ $$$${z}_{\mathrm{1}} =\frac{\mathrm{1}}{\mathrm{2}}+{i}\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\:{and}\:{z}_{\mathrm{2}} =\frac{\mathrm{1}}{\mathrm{2}}−{i}\frac{\sqrt{\mathrm{3}}}{\mathrm{2}} \\ $$$${let}\:{the}\:{root}\:{of}\:{x}^{\mathrm{2}} +{x}+\mathrm{1}=\mathrm{0}\:{are} \\ $$$${z}_{\mathrm{3}} =−\frac{\mathrm{1}}{\mathrm{2}}+{i}\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\:{and}\:{z}_{\mathrm{4}} =−\frac{\mathrm{1}}{\mathrm{2}}−{i}\frac{\sqrt{\mathrm{3}}}{\mathrm{2}} \\ $$$${by}\:{using}\:{the}\:{infinite}\:{semicycle}\:{on}\:{the} \\ $$$${upperhalf}\:{plane}\:{as}\:{the}\:{contour},{we}\:{have} \\ $$$${the}\:{poles}\:{has}\:{z}_{\mathrm{1}} =\frac{\mathrm{1}}{\mathrm{2}}+{i}\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\:{and}\:{z}_{\mathrm{3}} =−\frac{\mathrm{1}}{\mathrm{2}}+{i}\frac{\sqrt{\mathrm{3}}}{\mathrm{2}} \\ $$$${then}\:{apply}\:{residue}\:{theorem} \\ $$$${let} \\ $$$${f}\left({z}\right)=\frac{{e}^{\mathrm{2}{iz}} }{\mathrm{1}+{x}^{\mathrm{2}} +{x}^{\mathrm{4}} }=\frac{{e}^{\mathrm{2}{iz}} }{\left({z}−{z}_{\mathrm{1}} \right)\left({z}−{z}_{\mathrm{2}} \right)\left({z}−{z}_{\mathrm{3}} \right)\left({z}−{z}_{\mathrm{4}} \right)} \\ $$$${by}\:{residue}\:{theorem} \\ $$$${Res}\left[{f}\left({z}\right):{z}_{\mathrm{1}} \right]=\underset{{z}\rightarrow{z}_{\mathrm{1}} } {\mathrm{lim}}\left[\left({z}−{z}_{\mathrm{1}} \right){f}\left({z}\right)\right]=\frac{{e}^{\mathrm{2}{iz}_{\mathrm{1}} } }{\left({z}_{\mathrm{1}} −{z}_{\mathrm{2}} \right)\left({z}_{\mathrm{1}} −{z}_{\mathrm{3}} \right)\left({z}_{\mathrm{1}} −{z}_{\mathrm{4}} \right)} \\ $$$$=\frac{{e}^{−\sqrt{\mathrm{3}}+{i}} }{−\mathrm{3}+{i}\sqrt{\mathrm{3}}}=−\frac{\mathrm{1}}{\mathrm{12}}\left(\mathrm{3}+{i}\sqrt{\mathrm{3}}\right){e}^{−\sqrt{\mathrm{3}}+{i}} ......\left(\mathrm{1}\right)\:{and} \\ $$$${Res}\left[{f}\left({z}\right):{z}_{\mathrm{3}} \right]=\underset{{z}\rightarrow{z}_{\mathrm{3}} } {\mathrm{lim}}\left[\left({z}−{z}_{\mathrm{3}} \right){f}\left({z}\right)\right]=\frac{{e}^{\mathrm{2}{iz}_{\mathrm{3}} } }{\left({z}_{\mathrm{3}} −{z}_{\mathrm{1}} \right)\left({z}_{\mathrm{3}} −{z}_{\mathrm{2}} \right)\left({z}_{\mathrm{3}} −{z}_{\mathrm{4}} \right)} \\ $$$$=\frac{{e}^{−\sqrt{\mathrm{3}}−{i}} }{\mathrm{3}+{i}\sqrt{\mathrm{3}}}=\frac{\mathrm{1}}{\mathrm{12}}\left(\mathrm{3}−{i}\sqrt{\mathrm{3}}\right){e}^{−\sqrt{\mathrm{3}}−{i}} .........\left(\mathrm{2}\right)\:{but} \\ $$$$\int_{{R}} {f}\left({z}\right){dz}=\mathrm{2}\pi{i}\Sigma{Resf}\left({z}\right) \\ $$$$\mathrm{2}{I}=\mathrm{2}\pi{i}\left[\frac{\mathrm{1}}{\mathrm{12}}\left(\mathrm{3}−{i}\sqrt{\mathrm{3}}\right){e}^{−\sqrt{\mathrm{3}}−{i}} −\frac{\mathrm{1}}{\mathrm{12}}\left(\mathrm{3}+{i}\sqrt{\mathrm{3}}\right){e}^{−\sqrt{\mathrm{3}}+{i}} \right] \\ $$$$\mathrm{2}{I}=\frac{\pi{e}^{−\sqrt{\mathrm{3}}} }{\mathrm{6}}\left[\left(\mathrm{3}{i}+\sqrt{\mathrm{3}}\right){e}^{−{i}} −\left(\mathrm{3}{i}−\sqrt{\mathrm{3}}\right){e}^{{i}} \right] \\ $$$$\mathrm{2}{I}=\frac{\pi{e}^{−\sqrt{\mathrm{3}}} }{\mathrm{6}}\left[−\mathrm{3}{i}\left({e}^{{i}} −{e}^{−{i}} \right)+\sqrt{\mathrm{3}}\left({e}^{{i}} +{e}^{−{i}} \right)\right] \\ $$$$\mathrm{2}{I}=\frac{\pi{e}^{−\sqrt{\mathrm{3}}} }{\mathrm{6}}\left[−\frac{\mathrm{6}{i}×{i}}{\mathrm{2}{i}}\left({e}^{{i}} −{e}^{−{i}} \right)+\frac{\mathrm{2}\sqrt{\mathrm{3}}}{\mathrm{2}}\left({e}^{{i}} +{e}^{−{i}} \right)\right] \\ $$$$\mathrm{2}{I}=\frac{\pi{e}^{−\sqrt{\mathrm{3}}} }{\mathrm{6}}\left[\mathrm{6}\left(\frac{{e}^{{i}} −{e}^{−{i}} }{\mathrm{2}{i}}\right)+\mathrm{2}\sqrt{\mathrm{3}}\left(\frac{{e}^{{i}} +{e}^{−{i}} }{\mathrm{2}}\right)\right] \\ $$$$\mathrm{2}{I}=\pi{e}^{−\sqrt{\mathrm{3}}} \left(\mathrm{sin}\left(\mathrm{1}\right)+\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}\mathrm{cos}\left(\mathrm{1}\right)\right) \\ $$$$\because{I}=\frac{\mathrm{1}}{\mathrm{2}}\pi{e}^{−\sqrt{\mathrm{3}}} \left[\mathrm{sin}\left(\mathrm{1}\right)+\frac{\sqrt{\mathrm{3}}}{\mathrm{3}}\mathrm{cos}\left(\mathrm{1}\right)\right] \\ $$$$\int_{\mathrm{0}} ^{\infty} \frac{\mathrm{cos}\left(\mathrm{2}{x}\right)}{\mathrm{1}+{x}^{\mathrm{2}} +{x}^{\mathrm{4}} }{dx}=\frac{\pi{e}^{−\sqrt{\mathrm{3}}} }{\mathrm{6}}\left[\mathrm{3sin}\left(\mathrm{1}\right)+\sqrt{\mathrm{3}}\mathrm{cos}\left(\mathrm{1}\right)\right] \\ $$$${by}\:{mathdave}\left(\mathrm{22}/\mathrm{09}/\mathrm{2020}\right) \\ $$$$ \\ $$
Commented by mathmax by abdo last updated on 23/Sep/20

$$\mathrm{thank}\:\mathrm{you}\:\mathrm{sir} \\ $$
Commented by mathdave last updated on 23/Sep/20

$${u}\:{re}\:{welcome} \\ $$
Commented by Tawa11 last updated on 06/Sep/21

$$\mathrm{great}\:\mathrm{sir} \\ $$
Answered by mathmax by abdo last updated on 27/Sep/20
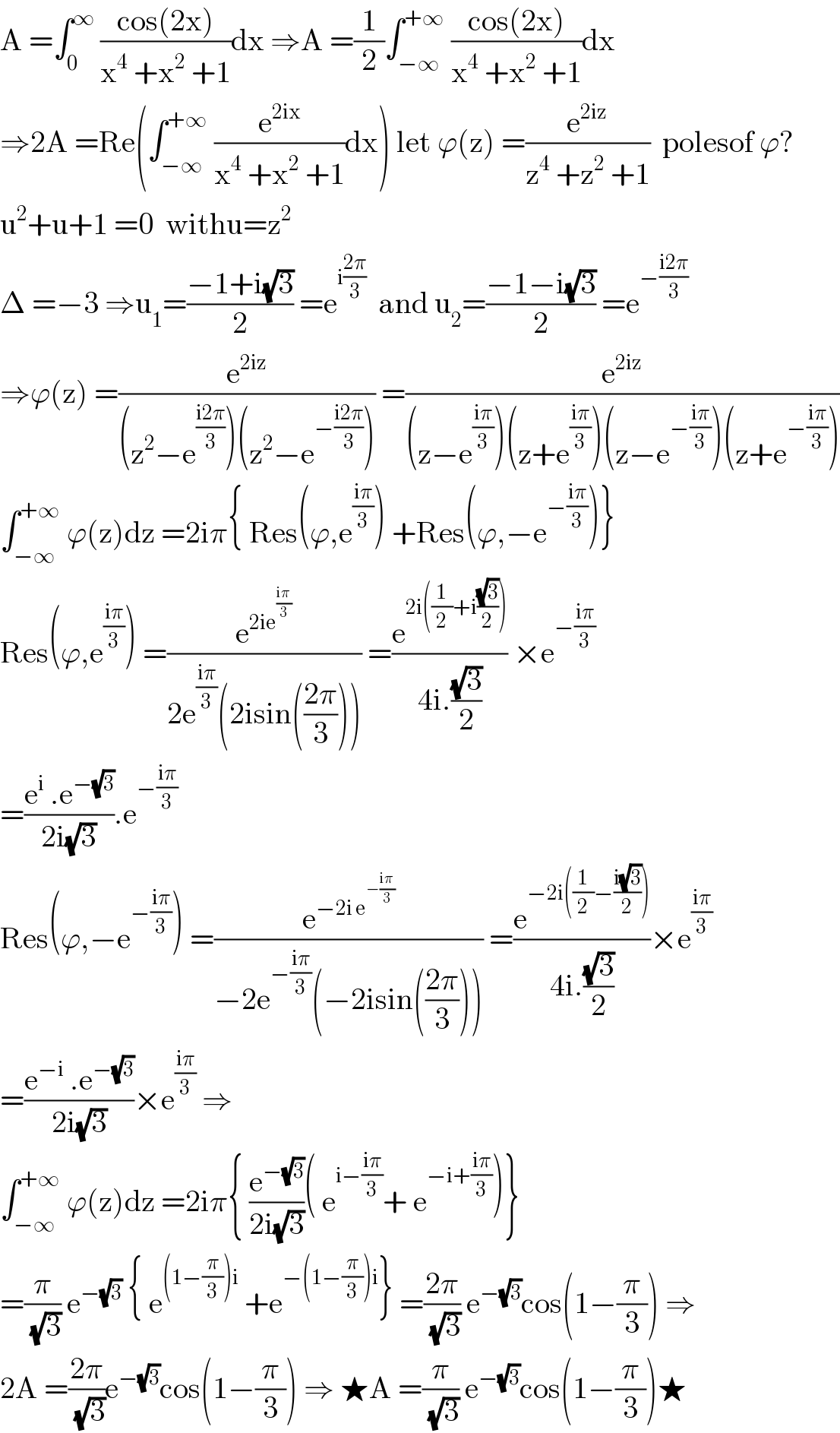
$$\mathrm{A}\:=\int_{\mathrm{0}} ^{\infty} \:\frac{\mathrm{cos}\left(\mathrm{2x}\right)}{\mathrm{x}^{\mathrm{4}} \:+\mathrm{x}^{\mathrm{2}} \:+\mathrm{1}}\mathrm{dx}\:\Rightarrow\mathrm{A}\:=\frac{\mathrm{1}}{\mathrm{2}}\int_{−\infty} ^{+\infty} \:\frac{\mathrm{cos}\left(\mathrm{2x}\right)}{\mathrm{x}^{\mathrm{4}} \:+\mathrm{x}^{\mathrm{2}} \:+\mathrm{1}}\mathrm{dx} \\ $$$$\Rightarrow\mathrm{2A}\:=\mathrm{Re}\left(\int_{−\infty} ^{+\infty} \:\frac{\mathrm{e}^{\mathrm{2ix}} }{\mathrm{x}^{\mathrm{4}} \:+\mathrm{x}^{\mathrm{2}} \:+\mathrm{1}}\mathrm{dx}\right)\:\mathrm{let}\:\varphi\left(\mathrm{z}\right)\:=\frac{\mathrm{e}^{\mathrm{2iz}} }{\mathrm{z}^{\mathrm{4}} \:+\mathrm{z}^{\mathrm{2}} \:+\mathrm{1}}\:\:\mathrm{polesof}\:\varphi? \\ $$$$\mathrm{u}^{\mathrm{2}} +\mathrm{u}+\mathrm{1}\:=\mathrm{0}\:\:\mathrm{withu}=\mathrm{z}^{\mathrm{2}} \\ $$$$\Delta\:=−\mathrm{3}\:\Rightarrow\mathrm{u}_{\mathrm{1}} =\frac{−\mathrm{1}+\mathrm{i}\sqrt{\mathrm{3}}}{\mathrm{2}}\:=\mathrm{e}^{\mathrm{i}\frac{\mathrm{2}\pi}{\mathrm{3}}} \:\:\mathrm{and}\:\mathrm{u}_{\mathrm{2}} =\frac{−\mathrm{1}−\mathrm{i}\sqrt{\mathrm{3}}}{\mathrm{2}}\:=\mathrm{e}^{−\frac{\mathrm{i2}\pi}{\mathrm{3}}} \\ $$$$\Rightarrow\varphi\left(\mathrm{z}\right)\:=\frac{\mathrm{e}^{\mathrm{2iz}} }{\left(\mathrm{z}^{\mathrm{2}} −\mathrm{e}^{\frac{\mathrm{i2}\pi}{\mathrm{3}}} \right)\left(\mathrm{z}^{\mathrm{2}} −\mathrm{e}^{−\frac{\mathrm{i2}\pi}{\mathrm{3}}} \right)}\:=\frac{\mathrm{e}^{\mathrm{2iz}} }{\left(\mathrm{z}−\mathrm{e}^{\frac{\mathrm{i}\pi}{\mathrm{3}}} \right)\left(\mathrm{z}+\mathrm{e}^{\frac{\mathrm{i}\pi}{\mathrm{3}}} \right)\left(\mathrm{z}−\mathrm{e}^{−\frac{\mathrm{i}\pi}{\mathrm{3}}} \right)\left(\mathrm{z}+\mathrm{e}^{−\frac{\mathrm{i}\pi}{\mathrm{3}}} \right)} \\ $$$$\int_{−\infty} ^{+\infty} \:\varphi\left(\mathrm{z}\right)\mathrm{dz}\:=\mathrm{2i}\pi\left\{\:\mathrm{Res}\left(\varphi,\mathrm{e}^{\frac{\mathrm{i}\pi}{\mathrm{3}}} \right)\:+\mathrm{Res}\left(\varphi,−\mathrm{e}^{−\frac{\mathrm{i}\pi}{\mathrm{3}}} \right)\right\} \\ $$$$\mathrm{Res}\left(\varphi,\mathrm{e}^{\frac{\mathrm{i}\pi}{\mathrm{3}}} \right)\:=\frac{\mathrm{e}^{\mathrm{2ie}^{\frac{\mathrm{i}\pi}{\mathrm{3}}} } }{\mathrm{2e}^{\frac{\mathrm{i}\pi}{\mathrm{3}}} \left(\mathrm{2isin}\left(\frac{\mathrm{2}\pi}{\mathrm{3}}\right)\right)}\:=\frac{\mathrm{e}^{\mathrm{2i}\left(\frac{\mathrm{1}}{\mathrm{2}}+\mathrm{i}\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\right)} }{\mathrm{4i}.\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}}\:×\mathrm{e}^{−\frac{\mathrm{i}\pi}{\mathrm{3}}} \\ $$$$=\frac{\mathrm{e}^{\mathrm{i}} \:.\mathrm{e}^{−\sqrt{\mathrm{3}}} }{\mathrm{2i}\sqrt{\mathrm{3}}}.\mathrm{e}^{−\frac{\mathrm{i}\pi}{\mathrm{3}}} \\ $$$$\mathrm{Res}\left(\varphi,−\mathrm{e}^{−\frac{\mathrm{i}\pi}{\mathrm{3}}} \right)\:=\frac{\mathrm{e}^{−\mathrm{2i}\:\mathrm{e}^{−\frac{\mathrm{i}\pi}{\mathrm{3}}} } }{−\mathrm{2e}^{−\frac{\mathrm{i}\pi}{\mathrm{3}}} \left(−\mathrm{2isin}\left(\frac{\mathrm{2}\pi}{\mathrm{3}}\right)\right)}\:=\frac{\mathrm{e}^{−\mathrm{2i}\left(\frac{\mathrm{1}}{\mathrm{2}}−\frac{\mathrm{i}\sqrt{\mathrm{3}}}{\mathrm{2}}\right)} }{\mathrm{4i}.\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}}×\mathrm{e}^{\frac{\mathrm{i}\pi}{\mathrm{3}}} \\ $$$$=\frac{\mathrm{e}^{−\mathrm{i}} \:.\mathrm{e}^{−\sqrt{\mathrm{3}}} }{\mathrm{2i}\sqrt{\mathrm{3}}}×\mathrm{e}^{\frac{\mathrm{i}\pi}{\mathrm{3}}} \:\Rightarrow \\ $$$$\int_{−\infty} ^{+\infty} \:\varphi\left(\mathrm{z}\right)\mathrm{dz}\:=\mathrm{2i}\pi\left\{\:\frac{\mathrm{e}^{−\sqrt{\mathrm{3}}} }{\mathrm{2i}\sqrt{\mathrm{3}}}\left(\:\mathrm{e}^{\mathrm{i}−\frac{\mathrm{i}\pi}{\mathrm{3}}} +\:\mathrm{e}^{−\mathrm{i}+\frac{\mathrm{i}\pi}{\mathrm{3}}} \right)\right\} \\ $$$$=\frac{\pi}{\sqrt{\mathrm{3}}}\:\mathrm{e}^{−\sqrt{\mathrm{3}}} \:\left\{\:\mathrm{e}^{\left(\mathrm{1}−\frac{\pi}{\mathrm{3}}\right)\mathrm{i}} \:+\mathrm{e}^{−\left(\mathrm{1}−\frac{\pi}{\mathrm{3}}\right)\mathrm{i}} \right\}\:=\frac{\mathrm{2}\pi}{\sqrt{\mathrm{3}}}\:\mathrm{e}^{−\sqrt{\mathrm{3}}} \mathrm{cos}\left(\mathrm{1}−\frac{\pi}{\mathrm{3}}\right)\:\Rightarrow \\ $$$$\mathrm{2A}\:=\frac{\mathrm{2}\pi}{\sqrt{\mathrm{3}}}\mathrm{e}^{−\sqrt{\mathrm{3}}} \mathrm{cos}\left(\mathrm{1}−\frac{\pi}{\mathrm{3}}\right)\:\Rightarrow\:\bigstar\mathrm{A}\:=\frac{\pi}{\sqrt{\mathrm{3}}}\:\mathrm{e}^{−\sqrt{\mathrm{3}}} \mathrm{cos}\left(\mathrm{1}−\frac{\pi}{\mathrm{3}}\right)\bigstar \\ $$
