
Question Number 112266 by Aina Samuel Temidayo last updated on 07/Sep/20

$$\mathrm{Let}\:\Omega\:\mathrm{denote}\:\mathrm{the}\:\mathrm{circumcircle}\:\mathrm{of}\:\mathrm{ABC}. \\ $$$$\mathrm{The}\:\mathrm{tangent}\:\mathrm{to}\:\Omega\:\mathrm{at}\:\mathrm{A}\:\mathrm{meets}\:\mathrm{BC}\:\mathrm{at}\:\mathrm{X}. \\ $$$$\mathrm{Let}\:\mathrm{the}\:\mathrm{angle}\:\mathrm{bisectors}\:\mathrm{of}\:\angle\mathrm{AXB}\:\mathrm{meet} \\ $$$$\mathrm{AC}\:\mathrm{and}\:\mathrm{AB}\:\mathrm{at}\:\mathrm{E}\:\mathrm{and}\:\mathrm{F} \\ $$$$\mathrm{respectively}.\:\mathrm{D}\:\mathrm{is}\:\mathrm{the}\:\mathrm{foot}\:\mathrm{of}\:\mathrm{the}\:\mathrm{angle} \\ $$$$\mathrm{bisector}\:\mathrm{from}\:\angle\mathrm{BAC}\:\mathrm{on}\:\mathrm{BC}.\:\mathrm{Let}\:\mathrm{AD} \\ $$$$\mathrm{intersect}\:\mathrm{EF}\:\mathrm{at}\:\mathrm{K}\:\mathrm{and}\:\Omega\:\mathrm{again}\:\mathrm{at} \\ $$$$\mathrm{L}\left(\mathrm{other}\:\mathrm{than}\:\mathrm{A}\right).\:\mathrm{Prove}\:\mathrm{that}\:\mathrm{AEDF}\:\mathrm{is} \\ $$$$\mathrm{a}\:\mathrm{rhombus}\:\mathrm{and}\:\mathrm{further}\:\mathrm{prove}\:\mathrm{that}\:\mathrm{the} \\ $$$$\mathrm{circle}\:\mathrm{defined}\:\mathrm{by}\:\mathrm{triangle}\:\mathrm{KLX}\:\mathrm{passes} \\ $$$$\mathrm{through}\:\mathrm{the}\:\mathrm{midpoint}\:\mathrm{of}\:\mathrm{line}\:\mathrm{segment} \\ $$$$\mathrm{BC}. \\ $$
Answered by 1549442205PVT last updated on 07/Sep/20

Commented by 1549442205PVT last updated on 07/Sep/20

$$\left.\mathrm{i}\right)\mathrm{Denote}\:\mathrm{by}\:\mathrm{H}\:\mathrm{the}\:\mathrm{midpoint}\:\mathrm{of}\:\mathrm{BC}.\mathrm{Since} \\ $$$$\mathrm{AL}\:\mathrm{is}\:\mathrm{the}\:\mathrm{bisector}\:\mathrm{ray}\:\mathrm{of}\:\mathrm{the}\:\mathrm{angle}\:\widehat {\mathrm{A}}, \\ $$$$\mathrm{L}\:\mathrm{is}\:\mathrm{middle}\:\mathrm{point}\:\mathrm{of}\:\mathrm{the}\:\mathrm{smaller}\:\mathrm{arc}\:\mathrm{BC} \\ $$$$\mathrm{Hence},\mathrm{three}\:\mathrm{points}\:\mathrm{O},\mathrm{H},\mathrm{L}\:\mathrm{colinear}. \\ $$$$\mathrm{and}\:\widehat {\mathrm{OHX}}=\mathrm{90}°\left(\mathrm{property}\:\mathrm{of}\:\mathrm{the}\:\mathrm{diameter}\right) \\ $$$$\mathrm{On}\:\mathrm{the}\:\mathrm{other}\:\mathrm{hands},\widehat {\mathrm{OAX}}=\mathrm{90}°\left(\mathrm{since}\right. \\ $$$$\left.\mathrm{XA}\:\mathrm{is}\:\mathrm{the}\:\mathrm{tangent}\:\mathrm{at}\:\mathrm{A}\:\mathrm{of}\:\mathrm{the}\:\mathrm{circle}\left(\mathrm{O}\right)\right) \\ $$$$\mathrm{We}\:\mathrm{infer}\:\mathrm{the}\:\mathrm{quadrilateral}\:\mathrm{AXHO}\:\mathrm{is} \\ $$$$\left.\mathrm{inscribed}\right)\Rightarrow\widehat {\mathrm{AXH}}+\widehat {\mathrm{AOH}}=\mathrm{180}°\left(\mathrm{1}\right).\mathrm{On}\:\mathrm{the} \\ $$$$\mathrm{other}\:\mathrm{hands},\mathrm{the}\:\mathrm{triangle}\:\mathrm{OAL}\:\mathrm{is}\:\mathrm{isosceles} \\ $$$$\mathrm{at}\:\mathrm{O},\mathrm{so}\:\widehat {\mathrm{OAL}}=\widehat {\mathrm{OLA}}=\alpha.\mathrm{Moreover}, \\ $$$$\mathrm{in}\:\mathrm{the}\:\mathrm{triangle}\:\mathrm{OAL}\:\mathrm{we}\:\mathrm{have} \\ $$$$\widehat {\:\mathrm{OAL}}+\widehat {\mathrm{OLA}}+\widehat {\mathrm{AOH}}=\mathrm{180}°\:\mathrm{infer} \\ $$$$\mathrm{2}\alpha+\widehat {\mathrm{AOH}}=\mathrm{180}°\:\left(\mathrm{2}\right).\mathrm{From}\:\left(\mathrm{1}\right)\left(\mathrm{2}\right)\mathrm{we}\:\mathrm{get} \\ $$$$\widehat {\mathrm{AXH}}=\mathrm{2}\alpha.\mathrm{From}\:\mathrm{that}\:\mathrm{since}\:\widehat {\mathrm{AXE}}= \\ $$$$\frac{\mathrm{1}}{\mathrm{2}}\widehat {\mathrm{AXH}}\:\mathrm{we}\:\mathrm{infer}\:\widehat {\mathrm{OAL}}=\widehat {\mathrm{AXE}}=\alpha. \\ $$$$\mathrm{This}\:\mathrm{gives}\:\mathrm{us}\:\mathrm{that}\:\widehat {\mathrm{KAX}}+\widehat {\mathrm{AXE}}= \\ $$$$\widehat {\mathrm{KAX}}+\widehat {\mathrm{OAL}}=\widehat {\mathrm{OAX}}=\mathrm{90}° \\ $$$$\mathrm{Thus},\mathrm{KD}\bot\mathrm{EF}.\mathrm{Consider}\:\mathrm{two}\:\mathrm{teiangles} \\ $$$$\mathrm{XAC}\:\mathrm{and}\:\mathrm{XBA}\:\mathrm{have}\:\mathrm{the}\:\mathrm{common}\:\mathrm{angle} \\ $$$$\widehat {\mathrm{AXC}},\widehat {\mathrm{ACB}}=\widehat {\mathrm{BAX}}.\mathrm{It}\:\mathrm{follows}\:\mathrm{that} \\ $$$$\Delta\mathrm{AXC}\backsim\Delta\mathrm{BXA}\left(\mathrm{a}.\mathrm{a}\right)\Rightarrow\frac{\mathrm{AX}}{\mathrm{BX}}=\frac{\mathrm{AC}}{\mathrm{AB}}\left(\mathrm{3}\right) \\ $$$$.\mathrm{On}\:\mathrm{the}\:\mathrm{other}\:\mathrm{hands},\mathrm{since}\:\mathrm{AD}\:\mathrm{is}\:\mathrm{the}\: \\ $$$$\mathrm{bisector}\:\mathrm{of}\:\mathrm{the}\:\mathrm{angle}\:\widehat {\mathrm{A}}\:\mathrm{we}\:\mathrm{have} \\ $$$$\frac{\mathrm{BD}}{\mathrm{DC}}=\frac{\mathrm{AB}}{\mathrm{AC}}\left(\mathrm{4}\right).\mathrm{Since}\:\mathrm{XF}\:\mathrm{is}\:\mathrm{the}\:\mathrm{bisector} \\ $$$$\mathrm{of}\:\mathrm{the}\:\widehat {\mathrm{AXB}},\frac{\mathrm{BF}}{\mathrm{FA}}=\frac{\mathrm{BX}}{\mathrm{AX}}\:\left(\mathrm{5}\right).\mathrm{From}\:\left(\mathrm{3}\right)\left(\mathrm{4}\right) \\ $$$$\left(\mathrm{5}\right)\mathrm{we}\:\mathrm{obtain}\:\frac{\mathrm{BD}}{\mathrm{DC}}=\frac{\mathrm{BF}}{\mathrm{FA}}\Rightarrow\mathrm{DF}//\mathrm{AC}. \\ $$$$\mathrm{Similarly},\mathrm{we}\:\mathrm{have}\:\mathrm{DE}//\mathrm{AB}\:\:,\mathrm{so} \\ $$$$\mathrm{quadrilateral}\:\mathrm{AEDF}\:\mathrm{is}\:\mathrm{a}\:\mathrm{parallelogram} \\ $$$$\mathrm{that}\:\mathrm{has}\:\mathrm{two}\:\mathrm{mutual}\:\mathrm{perpendicular}\: \\ $$$$\mathrm{diagonals}.\mathrm{That}\:\mathrm{shows}\:\mathrm{AEDF}\:\mathrm{is}\:\mathrm{a}\:\mathrm{rhombus}\left(\mathrm{q}.\mathrm{e}.\mathrm{d}\right) \\ $$$$\left.\mathrm{ii}\right)\mathrm{By}\:\mathrm{above}\:\mathrm{proof}\:\mathrm{we}\:\mathrm{have}\:\mathrm{O},\mathrm{H},\mathrm{L}\:\mathrm{are} \\ $$$$\mathrm{colinear}\:\left(\mathrm{H}−\mathrm{midpoint}\:\mathrm{of}\:\mathrm{BC}\right)\:\mathrm{and} \\ $$$$\widehat {\mathrm{OLA}}=\frac{\mathrm{1}}{\mathrm{2}}\widehat {\mathrm{AXH}}=\widehat {\mathrm{KXH}}=\alpha.\mathrm{Therefore}, \\ $$$$\mathrm{quadrilateral}\:\mathrm{HKXL}\:\mathrm{is}\:\mathrm{inscribed}\:\mathrm{which} \\ $$$$\mathrm{shows}\:\mathrm{that}\:\mathrm{the}\:\mathrm{circumcircle}\:\mathrm{of}\:\mathrm{triangle} \\ $$$$\mathrm{KXL}\:\mathrm{go}\:\mathrm{through}\:\mathrm{the}\:\mathrm{midpoint}\:\mathrm{H}\:\mathrm{of}\:\mathrm{BC} \\ $$$$\left(\boldsymbol{\mathrm{q}}.\boldsymbol{\mathrm{e}}.\boldsymbol{\mathrm{d}}\right) \\ $$
Commented by Aina Samuel Temidayo last updated on 07/Sep/20

$$\mathrm{Thanks}. \\ $$
Commented by Aina Samuel Temidayo last updated on 07/Sep/20

$$\mathrm{I}\:\mathrm{really}\:\mathrm{appreciate}\:\mathrm{your}\:\mathrm{efforts}. \\ $$
Commented by Aina Samuel Temidayo last updated on 07/Sep/20

$$\mathrm{Are}\:\mathrm{you}\:\mathrm{there}? \\ $$
Commented by Aina Samuel Temidayo last updated on 07/Sep/20

Commented by Aina Samuel Temidayo last updated on 07/Sep/20
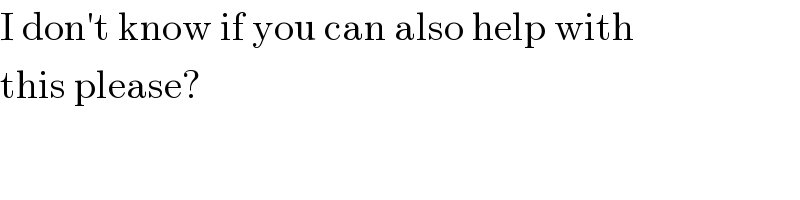
$$\mathrm{I}\:\mathrm{don}'\mathrm{t}\:\mathrm{know}\:\mathrm{if}\:\mathrm{you}\:\mathrm{can}\:\mathrm{also}\:\mathrm{help}\:\mathrm{with} \\ $$$$\mathrm{this}\:\mathrm{please}? \\ $$
Commented by Aina Samuel Temidayo last updated on 07/Sep/20
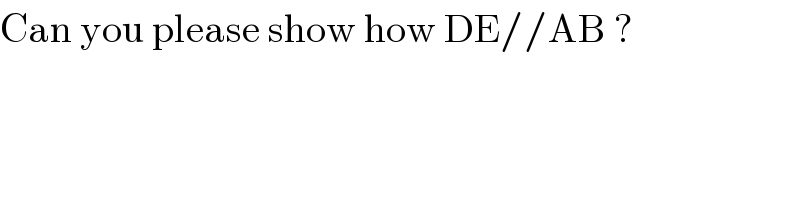
$$\mathrm{Can}\:\mathrm{you}\:\mathrm{please}\:\mathrm{show}\:\mathrm{how}\:\mathrm{DE}//\mathrm{AB}\:? \\ $$
Commented by 1549442205PVT last updated on 07/Sep/20

$$\mathrm{because}\:\mathrm{AL}\:\mathrm{is}\:\mathrm{bisector}\:\mathrm{of}\:\widehat {\mathrm{BAC}}. \\ $$
Commented by 1549442205PVT last updated on 07/Sep/20
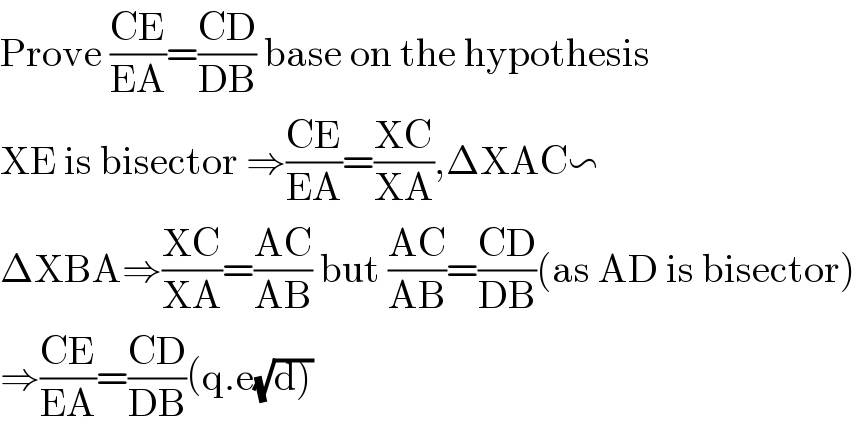
$$\mathrm{Prove}\:\frac{\mathrm{CE}}{\mathrm{EA}}=\frac{\mathrm{CD}}{\mathrm{DB}}\:\mathrm{base}\:\mathrm{on}\:\mathrm{the}\:\mathrm{hypothesis} \\ $$$$\mathrm{XE}\:\mathrm{is}\:\mathrm{bisector}\:\Rightarrow\frac{\mathrm{CE}}{\mathrm{EA}}=\frac{\mathrm{XC}}{\mathrm{XA}},\Delta\mathrm{XAC}\backsim \\ $$$$\Delta\mathrm{XBA}\Rightarrow\frac{\mathrm{XC}}{\mathrm{XA}}=\frac{\mathrm{AC}}{\mathrm{AB}}\:\mathrm{but}\:\frac{\mathrm{AC}}{\mathrm{AB}}=\frac{\mathrm{CD}}{\mathrm{DB}}\left(\mathrm{as}\:\mathrm{AD}\:\mathrm{is}\:\mathrm{bisector}\right) \\ $$$$\Rightarrow\frac{\mathrm{CE}}{\mathrm{EA}}=\frac{\mathrm{CD}}{\mathrm{DB}}\left(\mathrm{q}.\mathrm{e}\sqrt{\left.\mathrm{d}\right)}\right. \\ $$
Commented by Aina Samuel Temidayo last updated on 07/Sep/20

$$\mathrm{Thanks}. \\ $$$$ \\ $$
