
Question Number 111432 by ajfour last updated on 03/Sep/20
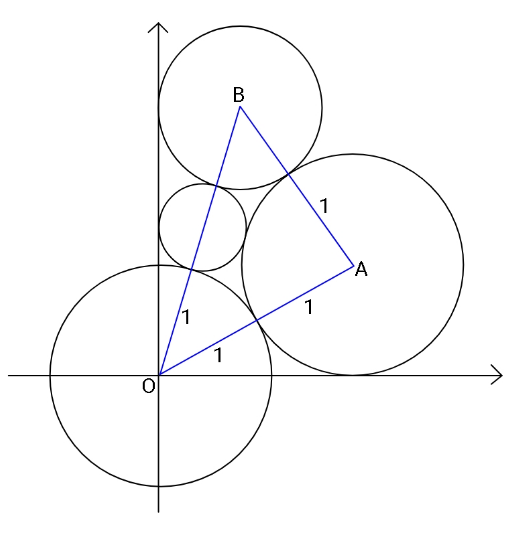
Commented by ajfour last updated on 03/Sep/20
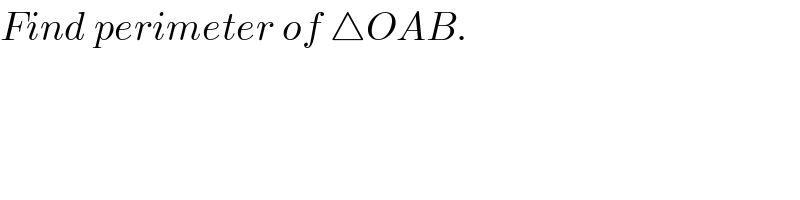
$${Find}\:{perimeter}\:{of}\:\bigtriangleup{OAB}. \\ $$
Commented by ajfour last updated on 04/Sep/20

$${mrW}\:{Sir},\:{can}\:{you}\:{please}\:{confirm} \\ $$$${answer}\:{to}\:{this}\:{question}? \\ $$
Answered by ajfour last updated on 04/Sep/20
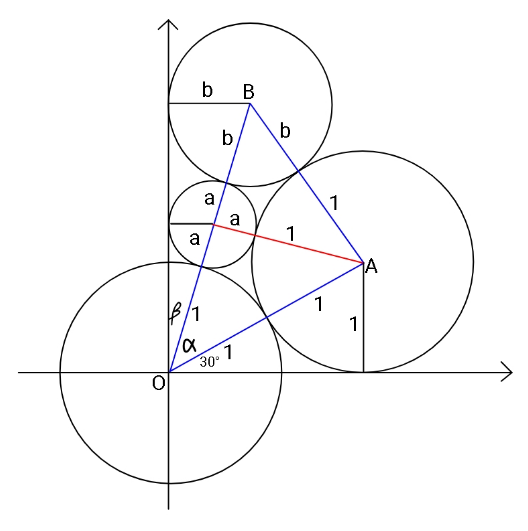
Commented by ajfour last updated on 04/Sep/20

$$\mathrm{cos}\:\alpha=\frac{\mathrm{1}}{{a}+\mathrm{1}} \\ $$$$\mathrm{sin}\:\beta=\frac{{a}}{{a}+\mathrm{1}}\:=\:\mathrm{1}−\mathrm{cos}\:\alpha\:=\frac{{b}}{\mathrm{1}+\mathrm{2}{a}+{b}} \\ $$$$\alpha+\beta=\mathrm{60}° \\ $$$$\Rightarrow\:\mathrm{sin}\:\beta\:=\frac{{a}}{{a}+\mathrm{1}}=\:\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\left(\frac{\mathrm{1}}{{a}+\mathrm{1}}\right)−\frac{\mathrm{1}}{\mathrm{2}}\sqrt{\mathrm{1}−\frac{\mathrm{1}}{\left({a}+\mathrm{1}\right)^{\mathrm{2}} }} \\ $$$$\Rightarrow\:\:\:\mathrm{2}{a}=\sqrt{\mathrm{3}}−\sqrt{\left({a}+\mathrm{1}\right)^{\mathrm{2}} −\mathrm{1}} \\ $$$$\Rightarrow\:\:\mathrm{3}+\mathrm{4}{a}^{\mathrm{2}} −\mathrm{4}\sqrt{\mathrm{3}}{a}\:=\:{a}^{\mathrm{2}} +\mathrm{2}{a} \\ $$$$\Rightarrow\:\:\:\:\mathrm{3}{a}^{\mathrm{2}} −\left(\mathrm{4}\sqrt{\mathrm{3}}+\mathrm{2}\right){a}+\mathrm{3}=\mathrm{0} \\ $$$$\Rightarrow\:\:{a}=\frac{\mathrm{2}\sqrt{\mathrm{3}}+\mathrm{1}}{\mathrm{3}}−\frac{\sqrt{\left(\mathrm{2}\sqrt{\mathrm{3}}+\mathrm{1}\right)^{\mathrm{2}} −\mathrm{9}}}{\mathrm{3}} \\ $$$$\Rightarrow\:\:{a}=\frac{\mathrm{2}\sqrt{\mathrm{3}}+\mathrm{1}−\mathrm{2}\sqrt{\mathrm{1}+\sqrt{\mathrm{3}}}}{\mathrm{3}}\:\approx\:\mathrm{0}.\mathrm{38611} \\ $$$$\:\:\:\:\:\:\:\mathrm{1}+\frac{\mathrm{1}}{{a}}=\mathrm{1}+\frac{\mathrm{2}{a}+\mathrm{1}}{{b}} \\ $$$$\Rightarrow\:\:\:\:\:{b}={a}\left(\mathrm{2}{a}+\mathrm{1}\right)\approx\:\mathrm{0}.\mathrm{68425} \\ $$$$..... \\ $$
Commented by mr W last updated on 06/Feb/25
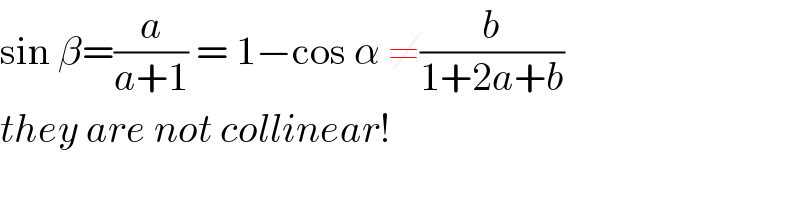
$$\mathrm{sin}\:\beta=\frac{{a}}{{a}+\mathrm{1}}\:=\:\mathrm{1}−\mathrm{cos}\:\alpha\:\neq\frac{{b}}{\mathrm{1}+\mathrm{2}{a}+{b}} \\ $$$${they}\:{are}\:{not}\:{collinear}! \\ $$
Answered by 1549442205PVT last updated on 05/Sep/20
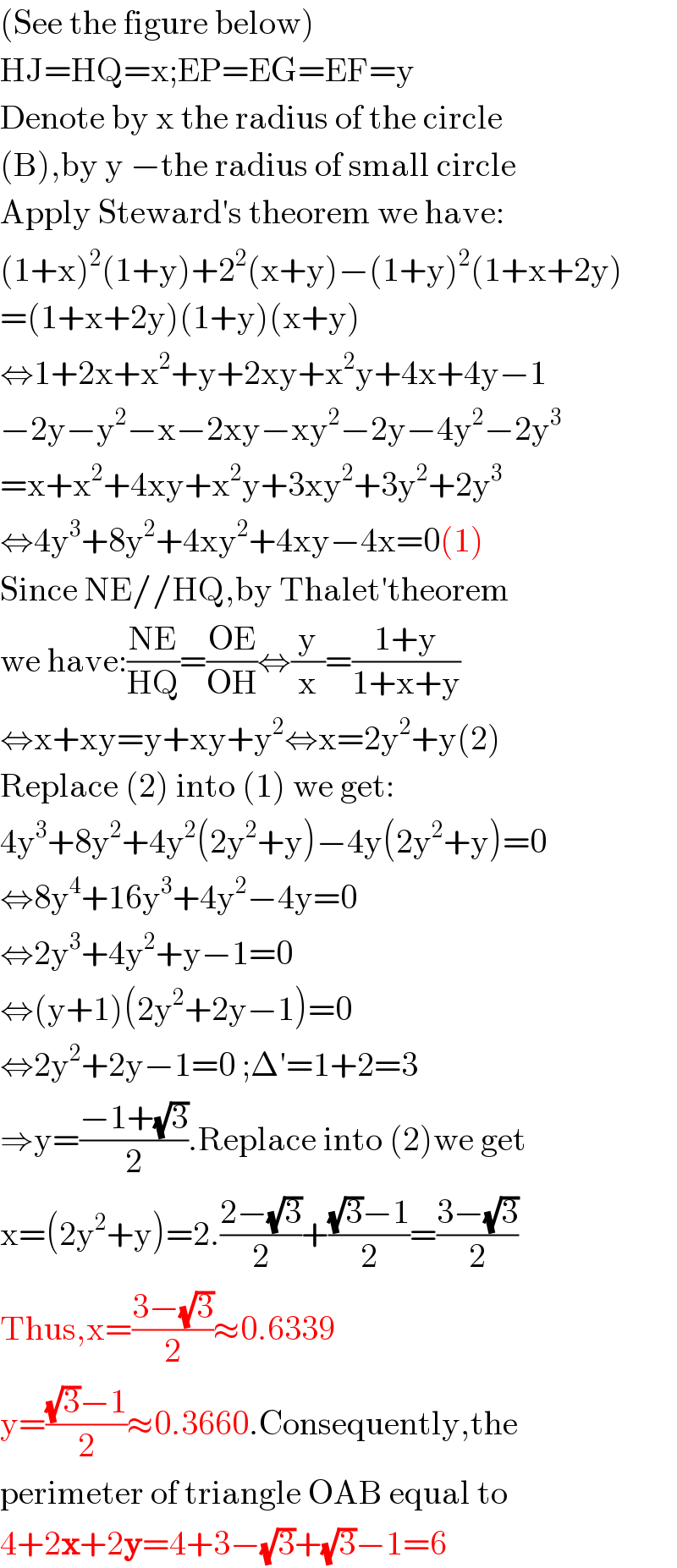
$$\left(\mathrm{See}\:\mathrm{the}\:\mathrm{figure}\:\mathrm{below}\right) \\ $$$$\mathrm{HJ}=\mathrm{HQ}=\mathrm{x};\mathrm{EP}=\mathrm{EG}=\mathrm{EF}=\mathrm{y} \\ $$$$\mathrm{Denote}\:\mathrm{by}\:\mathrm{x}\:\mathrm{the}\:\mathrm{radius}\:\mathrm{of}\:\mathrm{the}\:\mathrm{circle} \\ $$$$\left(\mathrm{B}\right),\mathrm{by}\:\mathrm{y}\:−\mathrm{the}\:\mathrm{radius}\:\mathrm{of}\:\mathrm{small}\:\mathrm{circle} \\ $$$$\mathrm{Apply}\:\mathrm{Steward}'\mathrm{s}\:\mathrm{theorem}\:\mathrm{we}\:\mathrm{have}: \\ $$$$\left(\mathrm{1}+\mathrm{x}\right)^{\mathrm{2}} \left(\mathrm{1}+\mathrm{y}\right)+\mathrm{2}^{\mathrm{2}} \left(\mathrm{x}+\mathrm{y}\right)−\left(\mathrm{1}+\mathrm{y}\right)^{\mathrm{2}} \left(\mathrm{1}+\mathrm{x}+\mathrm{2y}\right) \\ $$$$=\left(\mathrm{1}+\mathrm{x}+\mathrm{2y}\right)\left(\mathrm{1}+\mathrm{y}\right)\left(\mathrm{x}+\mathrm{y}\right) \\ $$$$\Leftrightarrow\mathrm{1}+\mathrm{2x}+\mathrm{x}^{\mathrm{2}} +\mathrm{y}+\mathrm{2xy}+\mathrm{x}^{\mathrm{2}} \mathrm{y}+\mathrm{4x}+\mathrm{4y}−\mathrm{1} \\ $$$$−\mathrm{2y}−\mathrm{y}^{\mathrm{2}} −\mathrm{x}−\mathrm{2xy}−\mathrm{xy}^{\mathrm{2}} −\mathrm{2y}−\mathrm{4y}^{\mathrm{2}} −\mathrm{2y}^{\mathrm{3}} \\ $$$$=\mathrm{x}+\mathrm{x}^{\mathrm{2}} +\mathrm{4xy}+\mathrm{x}^{\mathrm{2}} \mathrm{y}+\mathrm{3xy}^{\mathrm{2}} +\mathrm{3y}^{\mathrm{2}} +\mathrm{2y}^{\mathrm{3}} \\ $$$$\Leftrightarrow\mathrm{4y}^{\mathrm{3}} +\mathrm{8y}^{\mathrm{2}} +\mathrm{4xy}^{\mathrm{2}} +\mathrm{4xy}−\mathrm{4x}=\mathrm{0}\left(\mathrm{1}\right) \\ $$$$\mathrm{Since}\:\mathrm{NE}//\mathrm{HQ},\mathrm{by}\:\mathrm{Thalet}'\mathrm{theorem} \\ $$$$\mathrm{we}\:\mathrm{have}:\frac{\mathrm{NE}}{\mathrm{HQ}}=\frac{\mathrm{OE}}{\mathrm{OH}}\Leftrightarrow\frac{\mathrm{y}}{\mathrm{x}}=\frac{\mathrm{1}+\mathrm{y}}{\mathrm{1}+\mathrm{x}+\mathrm{y}} \\ $$$$\Leftrightarrow\mathrm{x}+\mathrm{xy}=\mathrm{y}+\mathrm{xy}+\mathrm{y}^{\mathrm{2}} \Leftrightarrow\mathrm{x}=\mathrm{2y}^{\mathrm{2}} +\mathrm{y}\left(\mathrm{2}\right) \\ $$$$\mathrm{Replace}\:\left(\mathrm{2}\right)\:\mathrm{into}\:\left(\mathrm{1}\right)\:\mathrm{we}\:\mathrm{get}: \\ $$$$\mathrm{4y}^{\mathrm{3}} +\mathrm{8y}^{\mathrm{2}} +\mathrm{4y}^{\mathrm{2}} \left(\mathrm{2y}^{\mathrm{2}} +\mathrm{y}\right)−\mathrm{4y}\left(\mathrm{2y}^{\mathrm{2}} +\mathrm{y}\right)=\mathrm{0} \\ $$$$\Leftrightarrow\mathrm{8y}^{\mathrm{4}} +\mathrm{16y}^{\mathrm{3}} +\mathrm{4y}^{\mathrm{2}} −\mathrm{4y}=\mathrm{0} \\ $$$$\Leftrightarrow\mathrm{2y}^{\mathrm{3}} +\mathrm{4y}^{\mathrm{2}} +\mathrm{y}−\mathrm{1}=\mathrm{0} \\ $$$$\Leftrightarrow\left(\mathrm{y}+\mathrm{1}\right)\left(\mathrm{2y}^{\mathrm{2}} +\mathrm{2y}−\mathrm{1}\right)=\mathrm{0} \\ $$$$\Leftrightarrow\mathrm{2y}^{\mathrm{2}} +\mathrm{2y}−\mathrm{1}=\mathrm{0}\:;\Delta'=\mathrm{1}+\mathrm{2}=\mathrm{3} \\ $$$$\Rightarrow\mathrm{y}=\frac{−\mathrm{1}+\sqrt{\mathrm{3}}}{\mathrm{2}}.\mathrm{Replace}\:\mathrm{into}\:\left(\mathrm{2}\right)\mathrm{we}\:\mathrm{get} \\ $$$$\mathrm{x}=\left(\mathrm{2y}^{\mathrm{2}} +\mathrm{y}\right)=\mathrm{2}.\frac{\mathrm{2}−\sqrt{\mathrm{3}}}{\mathrm{2}}+\frac{\sqrt{\mathrm{3}}−\mathrm{1}}{\mathrm{2}}=\frac{\mathrm{3}−\sqrt{\mathrm{3}}}{\mathrm{2}} \\ $$$$\mathrm{Thus},\mathrm{x}=\frac{\mathrm{3}−\sqrt{\mathrm{3}}}{\mathrm{2}}\approx\mathrm{0}.\mathrm{6339} \\ $$$$\mathrm{y}=\frac{\sqrt{\mathrm{3}}−\mathrm{1}}{\mathrm{2}}\approx\mathrm{0}.\mathrm{3660}.\mathrm{Consequently},\mathrm{the} \\ $$$$\mathrm{perimeter}\:\mathrm{of}\:\mathrm{triangle}\:\mathrm{OAB}\:\mathrm{equal}\:\mathrm{to} \\ $$$$\mathrm{4}+\mathrm{2}\boldsymbol{\mathrm{x}}+\mathrm{2}\boldsymbol{\mathrm{y}}=\mathrm{4}+\mathrm{3}−\sqrt{\mathrm{3}}+\sqrt{\mathrm{3}}−\mathrm{1}=\mathrm{6} \\ $$
Commented by 1549442205PVT last updated on 04/Sep/20
Commented by ajfour last updated on 04/Sep/20
$${question}\:{is}\:{alright}\:{sir}. \\ $$
Commented by 1549442205PVT last updated on 05/Sep/20

$$\mathrm{Thank}\:\mathrm{you}\:,\mathrm{Sir}.\:\mathrm{I}\:\mathrm{mistaked}\:\mathrm{and}\:\mathrm{corrected} \\ $$$$\mathrm{E}\:\mathrm{is}\:\mathrm{vertex}\:\mathrm{of}\:\mathrm{isosceles}\:\mathrm{triangle}.\mathrm{But} \\ $$$$\mathrm{my}\:\mathrm{solution}\:\mathrm{don}'\mathrm{t}\:\mathrm{use}\:\mathrm{this}\:\mathrm{hypothesis} \\ $$
