
Question Number 109483 by ajfour last updated on 24/Aug/20
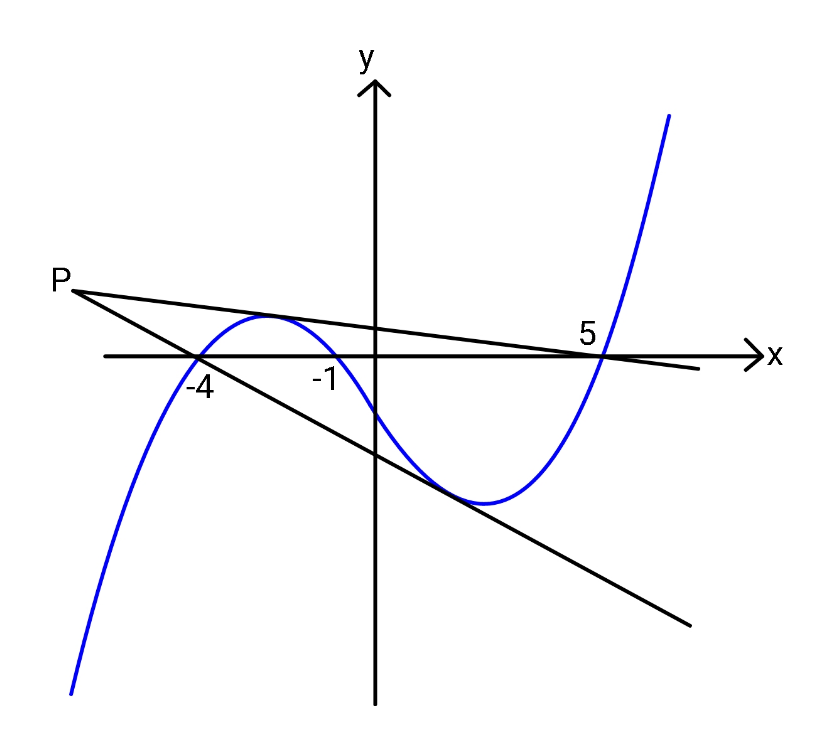
Commented by ajfour last updated on 24/Aug/20
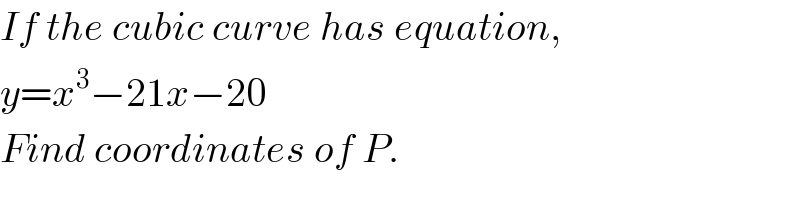
$${If}\:{the}\:{cubic}\:{curve}\:{has}\:{equation}, \\ $$$${y}={x}^{\mathrm{3}} −\mathrm{21}{x}−\mathrm{20} \\ $$$${Find}\:{coordinates}\:{of}\:{P}. \\ $$
Commented by ajfour last updated on 24/Aug/20
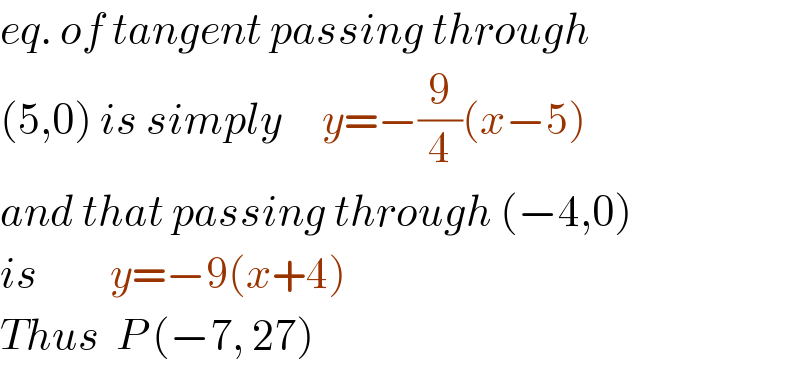
$${eq}.\:{of}\:{tangent}\:{passing}\:{through} \\ $$$$\left(\mathrm{5},\mathrm{0}\right)\:{is}\:{simply}\:\:\:\:\:{y}=−\frac{\mathrm{9}}{\mathrm{4}}\left({x}−\mathrm{5}\right) \\ $$$${and}\:{that}\:{passing}\:{through}\:\left(−\mathrm{4},\mathrm{0}\right) \\ $$$${is}\:\:\:\:\:\:\:\:\:{y}=−\mathrm{9}\left({x}+\mathrm{4}\right) \\ $$$${Thus}\:\:{P}\:\left(−\mathrm{7},\:\mathrm{27}\right) \\ $$
Answered by 1549442205PVT last updated on 25/Aug/20
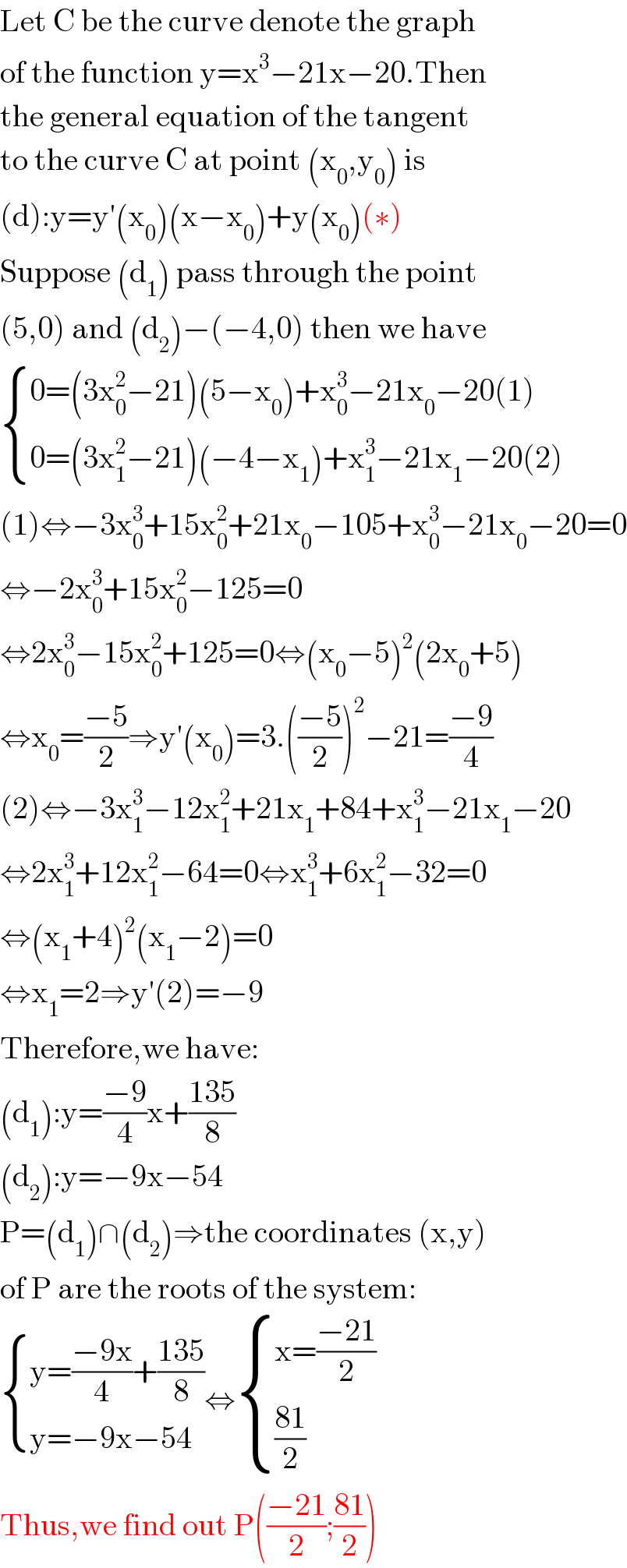
$$\mathrm{Let}\:\mathrm{C}\:\mathrm{be}\:\mathrm{the}\:\mathrm{curve}\:\mathrm{denote}\:\mathrm{the}\:\mathrm{graph} \\ $$$$\mathrm{of}\:\mathrm{the}\:\mathrm{function}\:\mathrm{y}=\mathrm{x}^{\mathrm{3}} −\mathrm{21x}−\mathrm{20}.\mathrm{Then} \\ $$$$\mathrm{the}\:\mathrm{general}\:\mathrm{equation}\:\mathrm{of}\:\mathrm{the}\:\mathrm{tangent} \\ $$$$\mathrm{to}\:\mathrm{the}\:\mathrm{curve}\:\mathrm{C}\:\mathrm{at}\:\mathrm{point}\:\left(\mathrm{x}_{\mathrm{0}} ,\mathrm{y}_{\mathrm{0}} \right)\:\mathrm{is} \\ $$$$\left(\mathrm{d}\right):\mathrm{y}=\mathrm{y}'\left(\mathrm{x}_{\mathrm{0}} \right)\left(\mathrm{x}−\mathrm{x}_{\mathrm{0}} \right)+\mathrm{y}\left(\mathrm{x}_{\mathrm{0}} \right)\left(\ast\right) \\ $$$$\mathrm{Suppose}\:\left(\mathrm{d}_{\mathrm{1}} \right)\:\mathrm{pass}\:\mathrm{through}\:\mathrm{the}\:\mathrm{point} \\ $$$$\left(\mathrm{5},\mathrm{0}\right)\:\mathrm{and}\:\left(\mathrm{d}_{\mathrm{2}} \right)−\left(−\mathrm{4},\mathrm{0}\right)\:\mathrm{then}\:\mathrm{we}\:\mathrm{have} \\ $$$$\begin{cases}{\mathrm{0}=\left(\mathrm{3x}_{\mathrm{0}} ^{\mathrm{2}} −\mathrm{21}\right)\left(\mathrm{5}−\mathrm{x}_{\mathrm{0}} \right)+\mathrm{x}_{\mathrm{0}} ^{\mathrm{3}} −\mathrm{21x}_{\mathrm{0}} −\mathrm{20}\left(\mathrm{1}\right)}\\{\mathrm{0}=\left(\mathrm{3x}_{\mathrm{1}} ^{\mathrm{2}} −\mathrm{21}\right)\left(−\mathrm{4}−\mathrm{x}_{\mathrm{1}} \right)+\mathrm{x}_{\mathrm{1}} ^{\mathrm{3}} −\mathrm{21x}_{\mathrm{1}} −\mathrm{20}\left(\mathrm{2}\right)}\end{cases} \\ $$$$\left(\mathrm{1}\right)\Leftrightarrow−\mathrm{3x}_{\mathrm{0}} ^{\mathrm{3}} +\mathrm{15x}_{\mathrm{0}} ^{\mathrm{2}} +\mathrm{21x}_{\mathrm{0}} −\mathrm{105}+\mathrm{x}_{\mathrm{0}} ^{\mathrm{3}} −\mathrm{21x}_{\mathrm{0}} −\mathrm{20}=\mathrm{0} \\ $$$$\Leftrightarrow−\mathrm{2x}_{\mathrm{0}} ^{\mathrm{3}} +\mathrm{15x}_{\mathrm{0}} ^{\mathrm{2}} −\mathrm{125}=\mathrm{0} \\ $$$$\Leftrightarrow\mathrm{2x}_{\mathrm{0}} ^{\mathrm{3}} −\mathrm{15x}_{\mathrm{0}} ^{\mathrm{2}} +\mathrm{125}=\mathrm{0}\Leftrightarrow\left(\mathrm{x}_{\mathrm{0}} −\mathrm{5}\right)^{\mathrm{2}} \left(\mathrm{2x}_{\mathrm{0}} +\mathrm{5}\right) \\ $$$$\Leftrightarrow\mathrm{x}_{\mathrm{0}} =\frac{−\mathrm{5}}{\mathrm{2}}\Rightarrow\mathrm{y}'\left(\mathrm{x}_{\mathrm{0}} \right)=\mathrm{3}.\left(\frac{−\mathrm{5}}{\mathrm{2}}\right)^{\mathrm{2}} −\mathrm{21}=\frac{−\mathrm{9}}{\mathrm{4}} \\ $$$$\left(\mathrm{2}\right)\Leftrightarrow−\mathrm{3x}_{\mathrm{1}} ^{\mathrm{3}} −\mathrm{12x}_{\mathrm{1}} ^{\mathrm{2}} +\mathrm{21x}_{\mathrm{1}} +\mathrm{84}+\mathrm{x}_{\mathrm{1}} ^{\mathrm{3}} −\mathrm{21x}_{\mathrm{1}} −\mathrm{20} \\ $$$$\Leftrightarrow\mathrm{2x}_{\mathrm{1}} ^{\mathrm{3}} +\mathrm{12x}_{\mathrm{1}} ^{\mathrm{2}} −\mathrm{64}=\mathrm{0}\Leftrightarrow\mathrm{x}_{\mathrm{1}} ^{\mathrm{3}} +\mathrm{6x}_{\mathrm{1}} ^{\mathrm{2}} −\mathrm{32}=\mathrm{0} \\ $$$$\Leftrightarrow\left(\mathrm{x}_{\mathrm{1}} +\mathrm{4}\right)^{\mathrm{2}} \left(\mathrm{x}_{\mathrm{1}} −\mathrm{2}\right)=\mathrm{0} \\ $$$$\Leftrightarrow\mathrm{x}_{\mathrm{1}} =\mathrm{2}\Rightarrow\mathrm{y}'\left(\mathrm{2}\right)=−\mathrm{9} \\ $$$$\mathrm{Therefore},\mathrm{we}\:\mathrm{have}: \\ $$$$\left(\mathrm{d}_{\mathrm{1}} \right):\mathrm{y}=\frac{−\mathrm{9}}{\mathrm{4}}\mathrm{x}+\frac{\mathrm{135}}{\mathrm{8}} \\ $$$$\left(\mathrm{d}_{\mathrm{2}} \right):\mathrm{y}=−\mathrm{9x}−\mathrm{54} \\ $$$$\mathrm{P}=\left(\mathrm{d}_{\mathrm{1}} \right)\cap\left(\mathrm{d}_{\mathrm{2}} \right)\Rightarrow\mathrm{the}\:\mathrm{coordinates}\:\left(\mathrm{x},\mathrm{y}\right) \\ $$$$\mathrm{of}\:\mathrm{P}\:\mathrm{are}\:\mathrm{the}\:\mathrm{roots}\:\mathrm{of}\:\mathrm{the}\:\mathrm{system}: \\ $$$$\begin{cases}{\mathrm{y}=\frac{−\mathrm{9x}}{\mathrm{4}}+\frac{\mathrm{135}}{\mathrm{8}}}\\{\mathrm{y}=−\mathrm{9x}−\mathrm{54}}\end{cases}\Leftrightarrow\begin{cases}{\mathrm{x}=\frac{−\mathrm{21}}{\mathrm{2}}}\\{\frac{\mathrm{81}}{\mathrm{2}}}\end{cases} \\ $$$$\mathrm{Thus},\mathrm{we}\:\mathrm{find}\:\mathrm{out}\:\mathrm{P}\left(\frac{−\mathrm{21}}{\mathrm{2}};\frac{\mathrm{81}}{\mathrm{2}}\right) \\ $$
Commented by ajfour last updated on 24/Aug/20

$${Thanks}\:{for}\:{your}\:{method}\:{Sir},\:{i} \\ $$$${think}\:{your}\:{finally}\:{obtained}\:{eqs}. \\ $$$${of}\:{lines}\:{are}\:{not}\:{correct},\:{please} \\ $$$${check}.. \\ $$
Commented by 1549442205PVT last updated on 25/Aug/20

$$\mathrm{Thank}\:\mathrm{Sir}\:.\mathrm{I}\:\mathrm{mistaked}.\mathrm{After}\:\mathrm{find}\:\mathrm{out} \\ $$$$\mathrm{the}\:\mathrm{slops}\:\mathrm{x}_{\mathrm{0}} =−\frac{\mathrm{9}}{\mathrm{4}}\:\mathrm{need}\:\mathrm{must}\:\mathrm{replace} \\ $$$$\mathrm{that}\:\mathrm{value}\:\mathrm{into}\:\:\left(\ast\right)\mathrm{to}\:\mathrm{find}\:\mathrm{free}\:\mathrm{efficient} \\ $$$$\mathrm{of}\:\mathrm{the}\:\mathrm{tangent}\:\mathrm{y}=−\frac{\mathrm{9}}{\mathrm{4}}\mathrm{x}+\mathrm{b} \\ $$$$\mathrm{b}=−\mathrm{y}'\left(\mathrm{x}_{\mathrm{0}} \right).+\mathrm{y}\left(\mathrm{x}_{\mathrm{0}} \right)=\frac{\mathrm{9}}{\mathrm{4}}.\left(−\frac{\mathrm{5}}{\mathrm{2}}\right)+\frac{\mathrm{135}}{\mathrm{8}} \\ $$$$=−\frac{\mathrm{45}}{\mathrm{8}}+\frac{\mathrm{135}}{\mathrm{8}}=\frac{\mathrm{90}}{\mathrm{8}}=\frac{\mathrm{45}}{\mathrm{4}}\Rightarrow\mathrm{y}=−\frac{\mathrm{9}}{\mathrm{4}}\mathrm{x}+\frac{\mathrm{45}}{\mathrm{4}} \\ $$$$\mathrm{For}\:\mathrm{x}_{\mathrm{1}} =\mathrm{2}.\mathrm{we}\:\mathrm{have}\:\mathrm{y}=−\mathrm{9x}+\mathrm{c} \\ $$$$\mathrm{c}=−\mathrm{y}'\left(\mathrm{x}_{\mathrm{1}} \right).\mathrm{x}_{\mathrm{1}} +\mathrm{y}\left(\mathrm{x}_{\mathrm{1}} \right)=\mathrm{9}.\mathrm{2}−\mathrm{54}=\mathrm{36} \\ $$$$\mathrm{we}\:\mathrm{get}\:\mathrm{y}=−\mathrm{9x}+\mathrm{36} \\ $$$$\mathrm{Sir}'\mathrm{s}\:\:\mathrm{results}\:\mathrm{are}\:\mathrm{correct} \\ $$
