
Previous in Permutation and Combination Next in Permutation and Combination
Question Number 107451 by mr W last updated on 11/Aug/20
![How many words can you form using the letters in UNUSUALLY such that no same letters are next to each other? [Answer: 10200]](Q107451.png)
$${How}\:{many}\:{words}\:{can}\:{you}\:{form}\:{using} \\ $$$${the}\:{letters}\:\:{in}\:\boldsymbol{{UNUSUALLY}} \\ $$$${such}\:{that}\:{no}\:{same}\:{letters}\:{are}\:\:{next} \\ $$$${to}\:{each}\:{other}? \\ $$$$ \\ $$$$\left[{Answer}:\:\mathrm{10200}\right] \\ $$
Commented by I want to learn more last updated on 11/Aug/20

$$\mathrm{Please}\:\mathrm{i}\:\mathrm{will}\:\mathrm{love}\:\mathrm{to}\:\mathrm{see}\:\mathrm{the}\:\mathrm{workings}\:\mathrm{sir}.\:\mathrm{Please} \\ $$
Answered by mr W last updated on 11/Aug/20
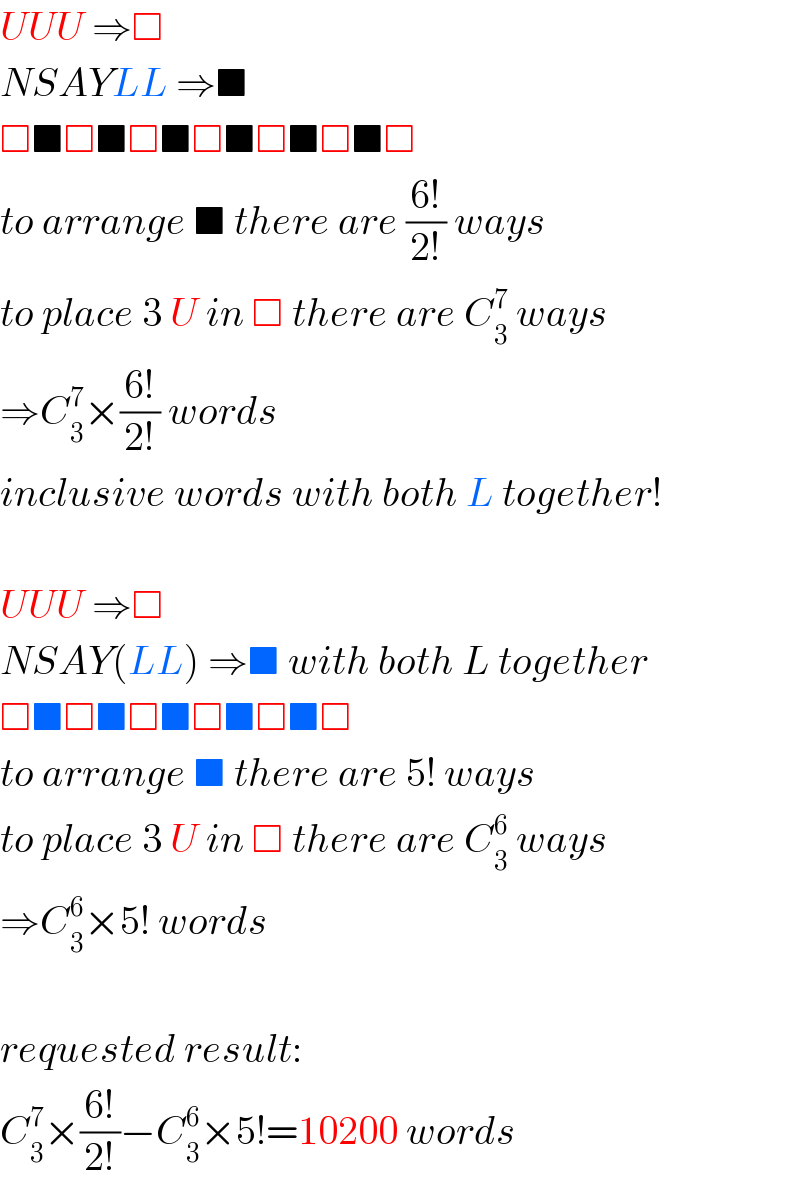
$${UUU}\:\Rightarrow\Box \\ $$$${NSAYLL}\:\Rightarrow\blacksquare \\ $$$$\Box\blacksquare\Box\blacksquare\Box\blacksquare\Box\blacksquare\Box\blacksquare\Box\blacksquare\Box \\ $$$${to}\:{arrange}\:\blacksquare\:{there}\:{are}\:\frac{\mathrm{6}!}{\mathrm{2}!}\:{ways} \\ $$$${to}\:{place}\:\mathrm{3}\:{U}\:{in}\:\Box\:{there}\:{are}\:{C}_{\mathrm{3}} ^{\mathrm{7}} \:{ways} \\ $$$$\Rightarrow{C}_{\mathrm{3}} ^{\mathrm{7}} ×\frac{\mathrm{6}!}{\mathrm{2}!}\:{words} \\ $$$${inclusive}\:{words}\:{with}\:{both}\:{L}\:{together}! \\ $$$$ \\ $$$${UUU}\:\Rightarrow\Box \\ $$$${NSAY}\left({LL}\right)\:\Rightarrow\blacksquare\:{with}\:{both}\:{L}\:{together} \\ $$$$\Box\blacksquare\Box\blacksquare\Box\blacksquare\Box\blacksquare\Box\blacksquare\Box \\ $$$${to}\:{arrange}\:\blacksquare\:{there}\:{are}\:\mathrm{5}!\:{ways} \\ $$$${to}\:{place}\:\mathrm{3}\:{U}\:{in}\:\Box\:{there}\:{are}\:{C}_{\mathrm{3}} ^{\mathrm{6}} \:{ways} \\ $$$$\Rightarrow{C}_{\mathrm{3}} ^{\mathrm{6}} ×\mathrm{5}!\:{words} \\ $$$$ \\ $$$${requested}\:{result}: \\ $$$${C}_{\mathrm{3}} ^{\mathrm{7}} ×\frac{\mathrm{6}!}{\mathrm{2}!}−{C}_{\mathrm{3}} ^{\mathrm{6}} ×\mathrm{5}!=\mathrm{10200}\:{words} \\ $$
Commented by I want to learn more last updated on 11/Aug/20
$$\mathrm{Wow},\:\:\:\mathrm{thanks}\:\mathrm{sir}. \\ $$
