
Question Number 107328 by mathdave last updated on 10/Aug/20

Commented by mnjuly1970 last updated on 10/Aug/20
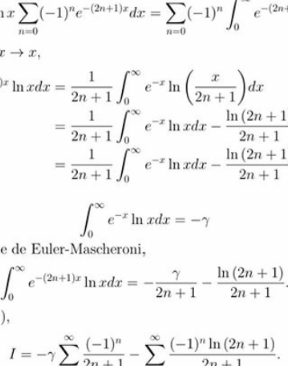
Commented by mnjuly1970 last updated on 10/Aug/20

Commented by mathdave last updated on 10/Aug/20

$${pls}\:{hw}\:{u}\:{got}\:{all}\:{ds} \\ $$
Commented by mathdave last updated on 10/Aug/20
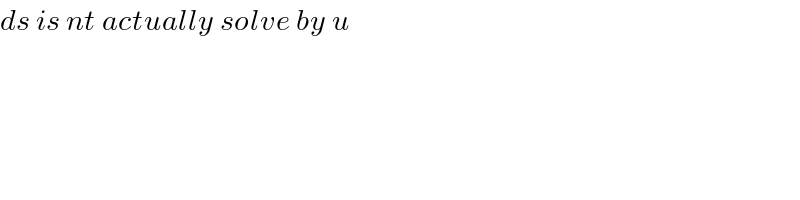
$${ds}\:{is}\:{nt}\:{actually}\:{solve}\:{by}\:{u} \\ $$
Commented by mnjuly1970 last updated on 10/Aug/20

$$\:\:\:\:\:\:{please}:\:{now}\:,{solve}\:{it} \\ $$$$\:\:\:\:\:\:{in}\:{your}\:{own}\:{way}! \\ $$$$ \\ $$$$ \\ $$
Commented by mathmax by abdo last updated on 10/Aug/20
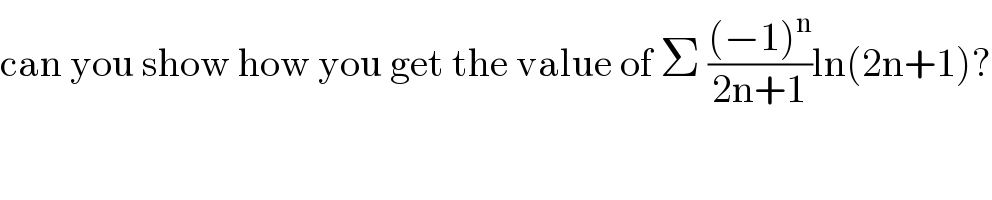
$$\mathrm{can}\:\mathrm{you}\:\mathrm{show}\:\mathrm{how}\:\mathrm{you}\:\mathrm{get}\:\mathrm{the}\:\mathrm{value}\:\mathrm{of}\:\Sigma\:\frac{\left(−\mathrm{1}\right)^{\mathrm{n}} }{\mathrm{2n}+\mathrm{1}}\mathrm{ln}\left(\mathrm{2n}+\mathrm{1}\right)? \\ $$
Answered by mathmax by abdo last updated on 10/Aug/20
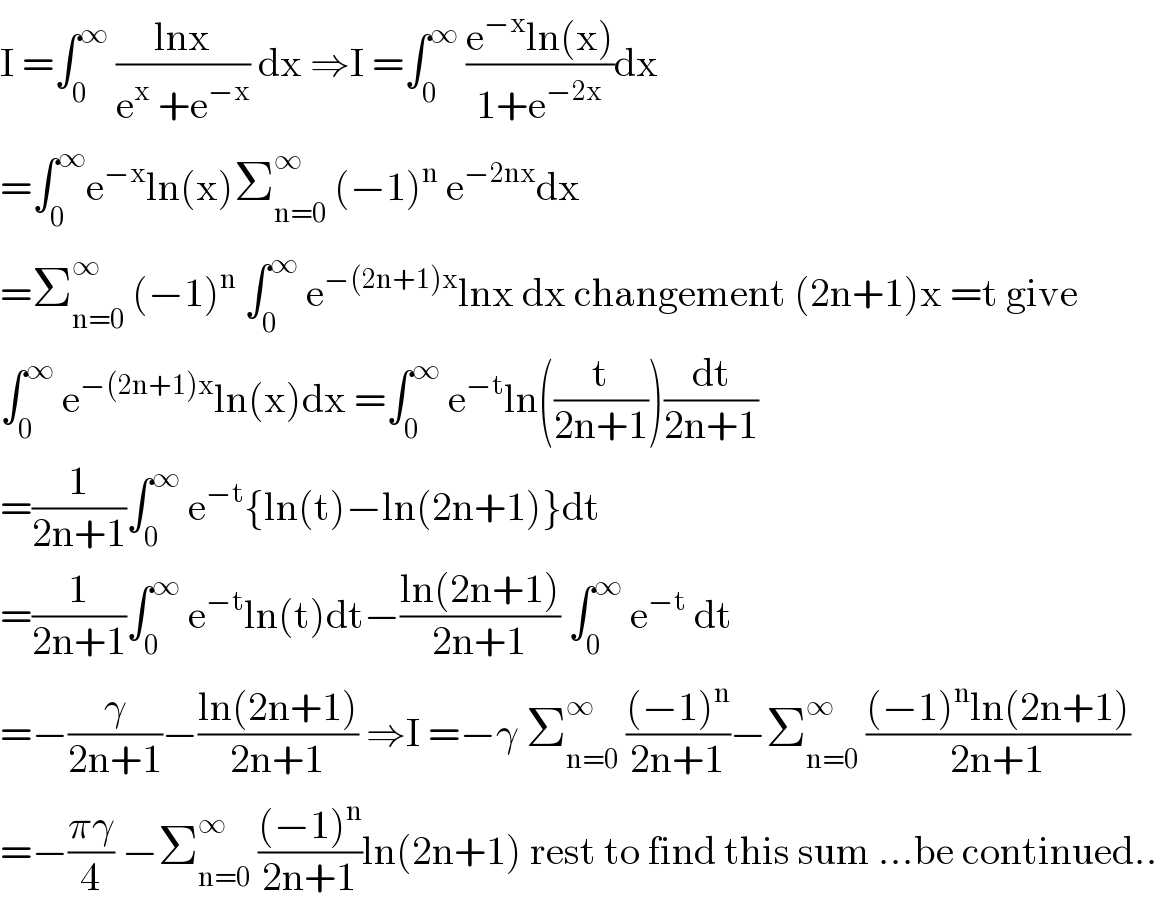
$$\mathrm{I}\:=\int_{\mathrm{0}} ^{\infty} \:\frac{\mathrm{lnx}}{\mathrm{e}^{\mathrm{x}} \:+\mathrm{e}^{−\mathrm{x}} }\:\mathrm{dx}\:\Rightarrow\mathrm{I}\:=\int_{\mathrm{0}} ^{\infty} \:\frac{\mathrm{e}^{−\mathrm{x}} \mathrm{ln}\left(\mathrm{x}\right)}{\mathrm{1}+\mathrm{e}^{−\mathrm{2x}} }\mathrm{dx} \\ $$$$=\int_{\mathrm{0}} ^{\infty} \mathrm{e}^{−\mathrm{x}} \mathrm{ln}\left(\mathrm{x}\right)\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \:\left(−\mathrm{1}\right)^{\mathrm{n}} \:\mathrm{e}^{−\mathrm{2nx}} \mathrm{dx} \\ $$$$=\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \:\left(−\mathrm{1}\right)^{\mathrm{n}} \:\int_{\mathrm{0}} ^{\infty} \:\mathrm{e}^{−\left(\mathrm{2n}+\mathrm{1}\right)\mathrm{x}} \mathrm{lnx}\:\mathrm{dx}\:\mathrm{changement}\:\left(\mathrm{2n}+\mathrm{1}\right)\mathrm{x}\:=\mathrm{t}\:\mathrm{give} \\ $$$$\int_{\mathrm{0}} ^{\infty} \:\mathrm{e}^{−\left(\mathrm{2n}+\mathrm{1}\right)\mathrm{x}} \mathrm{ln}\left(\mathrm{x}\right)\mathrm{dx}\:=\int_{\mathrm{0}} ^{\infty} \:\mathrm{e}^{−\mathrm{t}} \mathrm{ln}\left(\frac{\mathrm{t}}{\mathrm{2n}+\mathrm{1}}\right)\frac{\mathrm{dt}}{\mathrm{2n}+\mathrm{1}} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2n}+\mathrm{1}}\int_{\mathrm{0}} ^{\infty} \:\mathrm{e}^{−\mathrm{t}} \left\{\mathrm{ln}\left(\mathrm{t}\right)−\mathrm{ln}\left(\mathrm{2n}+\mathrm{1}\right)\right\}\mathrm{dt} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2n}+\mathrm{1}}\int_{\mathrm{0}} ^{\infty} \:\mathrm{e}^{−\mathrm{t}} \mathrm{ln}\left(\mathrm{t}\right)\mathrm{dt}−\frac{\mathrm{ln}\left(\mathrm{2n}+\mathrm{1}\right)}{\mathrm{2n}+\mathrm{1}}\:\int_{\mathrm{0}} ^{\infty} \:\mathrm{e}^{−\mathrm{t}} \:\mathrm{dt} \\ $$$$=−\frac{\gamma}{\mathrm{2n}+\mathrm{1}}−\frac{\mathrm{ln}\left(\mathrm{2n}+\mathrm{1}\right)}{\mathrm{2n}+\mathrm{1}}\:\Rightarrow\mathrm{I}\:=−\gamma\:\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \:\frac{\left(−\mathrm{1}\right)^{\mathrm{n}} }{\mathrm{2n}+\mathrm{1}}−\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \:\frac{\left(−\mathrm{1}\right)^{\mathrm{n}} \mathrm{ln}\left(\mathrm{2n}+\mathrm{1}\right)}{\mathrm{2n}+\mathrm{1}} \\ $$$$=−\frac{\pi\gamma}{\mathrm{4}}\:−\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \:\frac{\left(−\mathrm{1}\right)^{\mathrm{n}} }{\mathrm{2n}+\mathrm{1}}\mathrm{ln}\left(\mathrm{2n}+\mathrm{1}\right)\:\mathrm{rest}\:\mathrm{to}\:\mathrm{find}\:\mathrm{this}\:\mathrm{sum}\:...\mathrm{be}\:\mathrm{continued}.. \\ $$
Commented by mnjuly1970 last updated on 10/Aug/20
$$\:\:\:{perfect}\:.{mercey} \\ $$
Commented by mathdave last updated on 10/Aug/20
$${u}\:{had}\:{really}\:{done}\:{well}\:{ooo}\:{well}\:{done}\:{sir} \\ $$
Commented by mathmax by abdo last updated on 10/Aug/20

$$\mathrm{you}\:\mathrm{are}\:\mathrm{welcome} \\ $$
