
Previous in Permutation and Combination Next in Permutation and Combination
Question Number 106815 by pticantor last updated on 07/Aug/20

$$\:\:\:\boldsymbol{{please}}\:\boldsymbol{{help}}\:\boldsymbol{{me}}\:\boldsymbol{{to}}\:\boldsymbol{{show}} \\ $$$$\boldsymbol{{that}}\:\:\boldsymbol{{the}}\:\boldsymbol{{equation}}\: \\ $$$$\:\boldsymbol{{X}}^{\boldsymbol{{n}}} +\boldsymbol{{aX}}+\boldsymbol{{c}}=\mathrm{0}\:\boldsymbol{{can}}\:\boldsymbol{{not}}\:\boldsymbol{{have}} \\ $$$$\boldsymbol{{more}}\:\boldsymbol{{than}}\:\mathrm{3}\:\boldsymbol{{reals}}\:\boldsymbol{{solutions}} \\ $$$$ \\ $$
Answered by 1549442205PVT last updated on 07/Aug/20
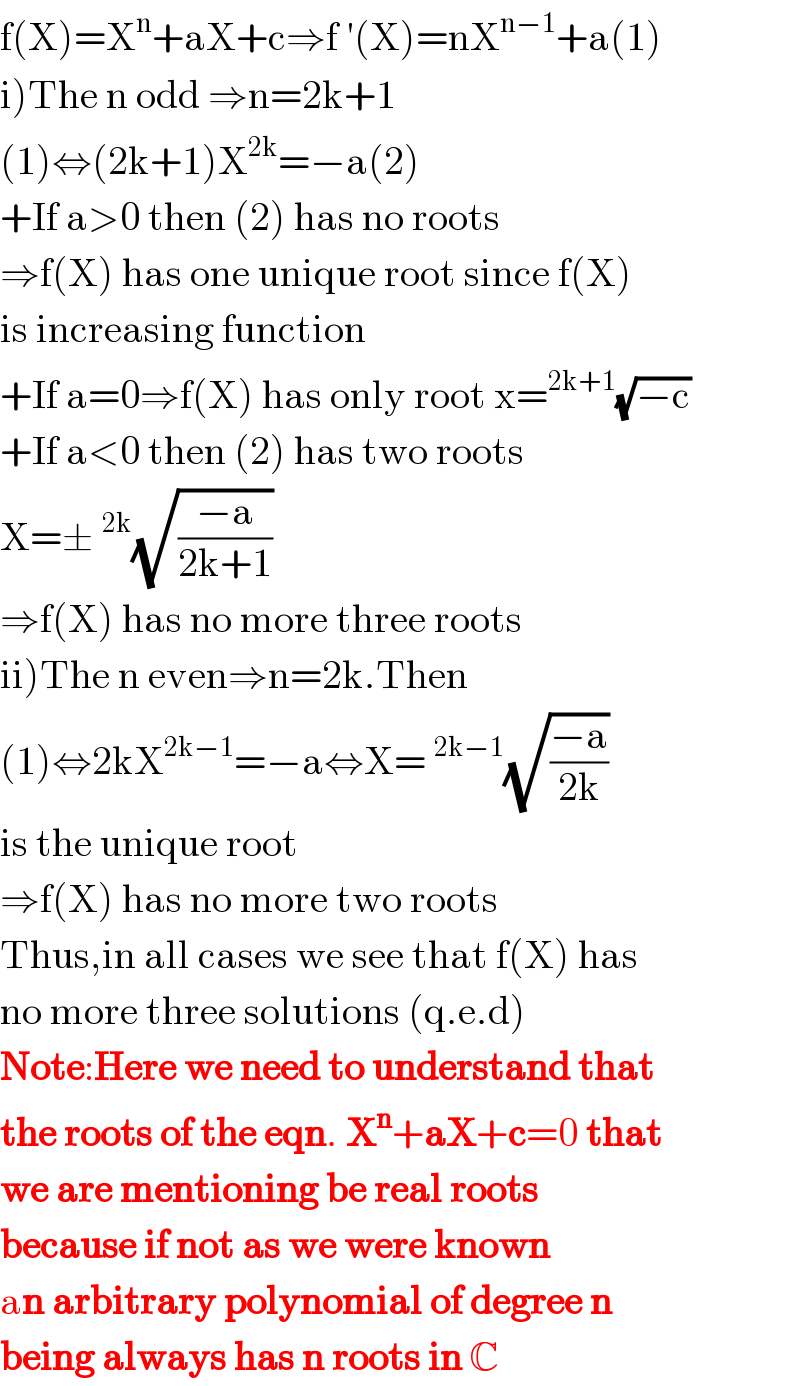
$$\mathrm{f}\left(\mathrm{X}\right)=\mathrm{X}^{\mathrm{n}} +\mathrm{aX}+\mathrm{c}\Rightarrow\mathrm{f}\:'\left(\mathrm{X}\right)=\mathrm{nX}^{\mathrm{n}−\mathrm{1}} +\mathrm{a}\left(\mathrm{1}\right) \\ $$$$\left.\mathrm{i}\right)\mathrm{The}\:\mathrm{n}\:\mathrm{odd}\:\Rightarrow\mathrm{n}=\mathrm{2k}+\mathrm{1} \\ $$$$\left(\mathrm{1}\right)\Leftrightarrow\left(\mathrm{2k}+\mathrm{1}\right)\mathrm{X}^{\mathrm{2k}} =−\mathrm{a}\left(\mathrm{2}\right) \\ $$$$+\mathrm{If}\:\mathrm{a}>\mathrm{0}\:\mathrm{then}\:\left(\mathrm{2}\right)\:\mathrm{has}\:\mathrm{no}\:\mathrm{roots} \\ $$$$\Rightarrow\mathrm{f}\left(\mathrm{X}\right)\:\mathrm{has}\:\mathrm{one}\:\mathrm{unique}\:\mathrm{root}\:\mathrm{since}\:\mathrm{f}\left(\mathrm{X}\right) \\ $$$$\mathrm{is}\:\mathrm{increasing}\:\mathrm{function} \\ $$$$+\mathrm{If}\:\mathrm{a}=\mathrm{0}\Rightarrow\mathrm{f}\left(\mathrm{X}\right)\:\mathrm{has}\:\mathrm{only}\:\mathrm{root}\:\mathrm{x}=^{\mathrm{2k}+\mathrm{1}} \sqrt{−\mathrm{c}} \\ $$$$+\mathrm{If}\:\mathrm{a}<\mathrm{0}\:\mathrm{then}\:\left(\mathrm{2}\right)\:\mathrm{has}\:\mathrm{two}\:\mathrm{roots} \\ $$$$\mathrm{X}=\pm\:^{\mathrm{2k}} \sqrt{\frac{−\mathrm{a}}{\mathrm{2k}+\mathrm{1}}} \\ $$$$\Rightarrow\mathrm{f}\left(\mathrm{X}\right)\:\mathrm{has}\:\mathrm{no}\:\mathrm{more}\:\mathrm{three}\:\mathrm{roots} \\ $$$$\left.\mathrm{ii}\right)\mathrm{The}\:\mathrm{n}\:\mathrm{even}\Rightarrow\mathrm{n}=\mathrm{2k}.\mathrm{Then} \\ $$$$\left(\mathrm{1}\right)\Leftrightarrow\mathrm{2kX}^{\mathrm{2k}−\mathrm{1}} =−\mathrm{a}\Leftrightarrow\mathrm{X}=\:^{\mathrm{2k}−\mathrm{1}} \sqrt{\frac{−\mathrm{a}}{\mathrm{2k}}} \\ $$$$\mathrm{is}\:\mathrm{the}\:\mathrm{unique}\:\mathrm{root} \\ $$$$\Rightarrow\mathrm{f}\left(\mathrm{X}\right)\:\mathrm{has}\:\mathrm{no}\:\mathrm{more}\:\mathrm{two}\:\mathrm{roots} \\ $$$$\mathrm{Thus},\mathrm{in}\:\mathrm{all}\:\mathrm{cases}\:\mathrm{we}\:\mathrm{see}\:\mathrm{that}\:\mathrm{f}\left(\mathrm{X}\right)\:\mathrm{has} \\ $$$$\mathrm{no}\:\mathrm{more}\:\mathrm{three}\:\mathrm{solutions}\:\left(\mathrm{q}.\mathrm{e}.\mathrm{d}\right) \\ $$$$\boldsymbol{\mathrm{Note}}:\boldsymbol{\mathrm{Here}}\:\boldsymbol{\mathrm{we}}\:\boldsymbol{\mathrm{need}}\:\boldsymbol{\mathrm{to}}\:\boldsymbol{\mathrm{understand}}\:\boldsymbol{\mathrm{that}}\: \\ $$$$\boldsymbol{\mathrm{the}}\:\boldsymbol{\mathrm{roots}}\:\boldsymbol{\mathrm{of}}\:\boldsymbol{\mathrm{the}}\:\boldsymbol{\mathrm{eqn}}.\:\boldsymbol{\mathrm{X}}^{\boldsymbol{\mathrm{n}}} +\boldsymbol{\mathrm{aX}}+\boldsymbol{\mathrm{c}}=\mathrm{0}\:\boldsymbol{\mathrm{that}} \\ $$$$\boldsymbol{\mathrm{we}}\:\boldsymbol{\mathrm{are}}\:\boldsymbol{\mathrm{mentioning}}\:\boldsymbol{\mathrm{be}}\:\boldsymbol{\mathrm{real}}\:\boldsymbol{\mathrm{roots}} \\ $$$$\boldsymbol{\mathrm{because}}\:\boldsymbol{\mathrm{if}}\:\boldsymbol{\mathrm{not}}\:\boldsymbol{\mathrm{as}}\:\boldsymbol{\mathrm{we}}\:\boldsymbol{\mathrm{were}}\:\boldsymbol{\mathrm{known}} \\ $$$$\mathrm{a}\boldsymbol{\mathrm{n}}\:\boldsymbol{\mathrm{arbitrary}}\:\boldsymbol{\mathrm{polynomial}}\:\boldsymbol{\mathrm{of}}\:\boldsymbol{\mathrm{degree}}\:\boldsymbol{\mathrm{n}}\: \\ $$$$\boldsymbol{\mathrm{being}}\:\boldsymbol{\mathrm{always}}\:\boldsymbol{\mathrm{has}}\:\boldsymbol{\mathrm{n}}\:\boldsymbol{\mathrm{roots}}\:\boldsymbol{\mathrm{in}}\:\mathbb{C} \\ $$
