
Question Number 10639 by Saham last updated on 21/Feb/17

$$\mathrm{A}\:\mathrm{manometer}\:\mathrm{wire}\:\mathrm{of}\:\mathrm{lenght}\:\mathrm{60}\:\mathrm{cm}\:\mathrm{is}\:\mathrm{maintained}\:\mathrm{under} \\ $$$$\mathrm{a}\:\mathrm{tension}\:\mathrm{of}\:\mathrm{value}\:\mathrm{20V}\:\mathrm{and}\:\mathrm{an}\:\mathrm{a}.\mathrm{c}\:\mathrm{is}\:\mathrm{passed}\:\mathrm{through} \\ $$$$\mathrm{the}\:\mathrm{wire}.\:\mathrm{If}\:\mathrm{the}\:\mathrm{density}\:\mathrm{of}\:\mathrm{the}\:\mathrm{wire}\:\mathrm{is}\:\mathrm{4000kgm}^{−\mathrm{3}} \:\mathrm{and}\:\mathrm{it}\: \\ $$$$\mathrm{diamerter}\:\mathrm{is}\:\mathrm{2mm}.\:\mathrm{Calculate}\:\mathrm{the}\:\mathrm{frequency}\:\mathrm{of}\:\mathrm{the}\:\mathrm{a}.\mathrm{c} \\ $$
Commented by Saham last updated on 21/Feb/17

$$\mathrm{please}\:\mathrm{help}\:\mathrm{with}\:\mathrm{this}\:\mathrm{too}.\:\mathrm{God}\:\mathrm{bless}\:\mathrm{you}. \\ $$
Commented by mrW1 last updated on 21/Feb/17
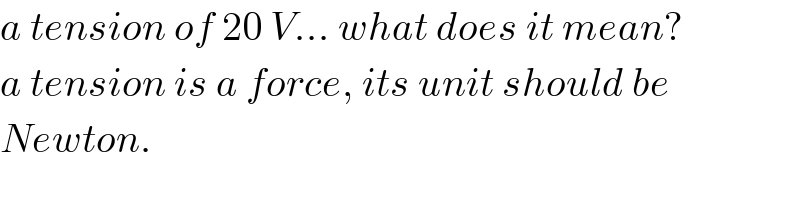
$${a}\:{tension}\:{of}\:\mathrm{20}\:{V}...\:{what}\:{does}\:{it}\:{mean}? \\ $$$${a}\:{tension}\:{is}\:{a}\:{force},\:{its}\:{unit}\:{should}\:{be} \\ $$$${Newton}. \\ $$
Commented by Saham last updated on 21/Feb/17
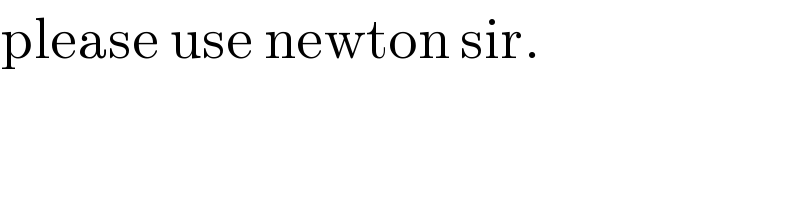
$$\mathrm{please}\:\mathrm{use}\:\mathrm{newton}\:\mathrm{sir}. \\ $$
Answered by mrW1 last updated on 21/Feb/17
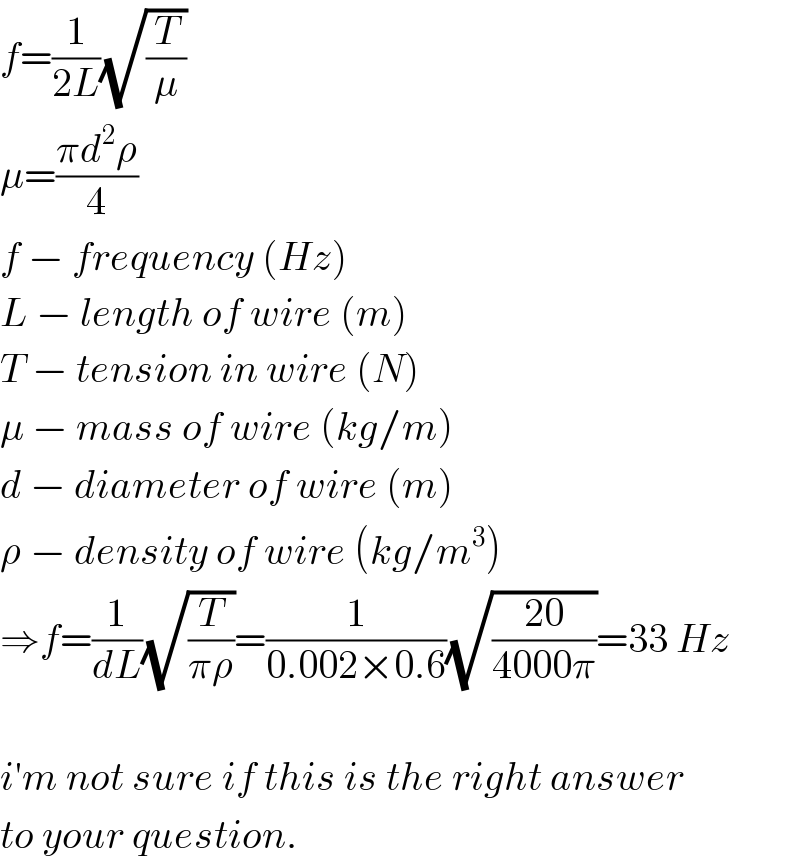
$${f}=\frac{\mathrm{1}}{\mathrm{2}{L}}\sqrt{\frac{{T}}{\mu}} \\ $$$$\mu=\frac{\pi{d}^{\mathrm{2}} \rho}{\mathrm{4}} \\ $$$${f}\:−\:{frequency}\:\left({Hz}\right) \\ $$$${L}\:−\:{length}\:{of}\:{wire}\:\left({m}\right) \\ $$$${T}\:−\:{tension}\:{in}\:{wire}\:\left({N}\right) \\ $$$$\mu\:−\:{mass}\:{of}\:{wire}\:\left({kg}/{m}\right) \\ $$$${d}\:−\:{diameter}\:{of}\:{wire}\:\left({m}\right) \\ $$$$\rho\:−\:{density}\:{of}\:{wire}\:\left({kg}/{m}^{\mathrm{3}} \right) \\ $$$$\Rightarrow{f}=\frac{\mathrm{1}}{{dL}}\sqrt{\frac{{T}}{\pi\rho}}=\frac{\mathrm{1}}{\mathrm{0}.\mathrm{002}×\mathrm{0}.\mathrm{6}}\sqrt{\frac{\mathrm{20}}{\mathrm{4000}\pi}}=\mathrm{33}\:{Hz} \\ $$$$ \\ $$$${i}'{m}\:{not}\:{sure}\:{if}\:{this}\:{is}\:{the}\:{right}\:{answer} \\ $$$${to}\:{your}\:{question}. \\ $$
Commented by Saham last updated on 21/Feb/17

$$\mathrm{Thank}\:\mathrm{you}\:\mathrm{sir}.\:\mathrm{God}\:\mathrm{bless}\:\mathrm{you}.\: \\ $$
Commented by Saham last updated on 21/Feb/17
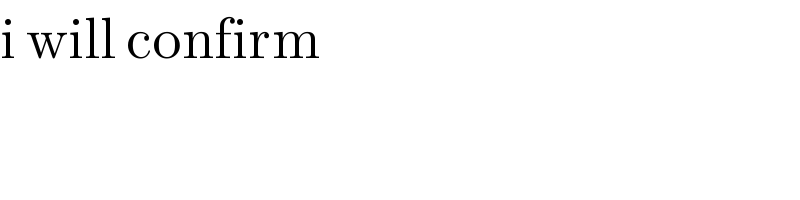
$$\mathrm{i}\:\mathrm{will}\:\mathrm{confirm} \\ $$
