
Question Number 105766 by bramlex last updated on 31/Jul/20
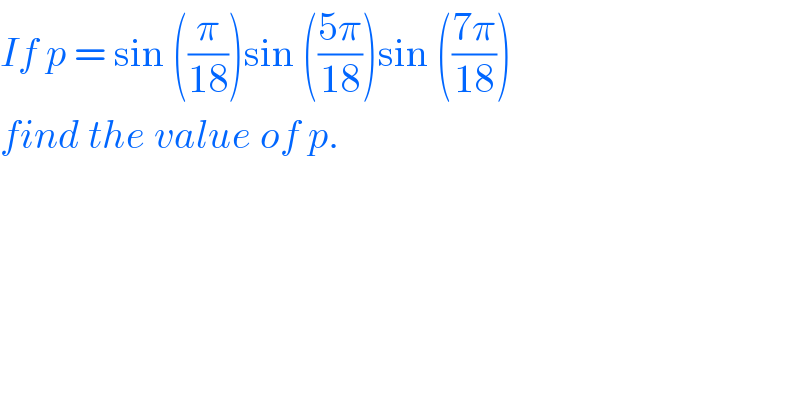
$${If}\:{p}\:=\:\mathrm{sin}\:\left(\frac{\pi}{\mathrm{18}}\right)\mathrm{sin}\:\left(\frac{\mathrm{5}\pi}{\mathrm{18}}\right)\mathrm{sin}\:\left(\frac{\mathrm{7}\pi}{\mathrm{18}}\right) \\ $$$${find}\:{the}\:{value}\:{of}\:{p}. \\ $$
Answered by som(math1967) last updated on 31/Jul/20
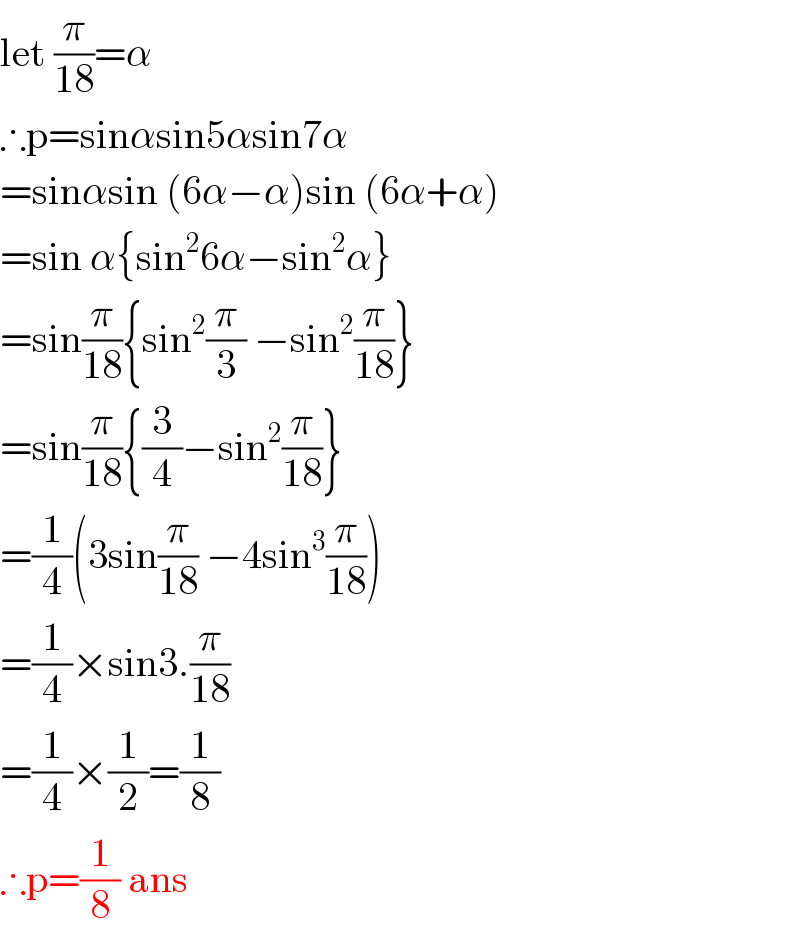
$$\mathrm{let}\:\frac{\pi}{\mathrm{18}}=\alpha \\ $$$$\therefore\mathrm{p}=\mathrm{sin}\alpha\mathrm{sin5}\alpha\mathrm{sin7}\alpha \\ $$$$=\mathrm{sin}\alpha\mathrm{sin}\:\left(\mathrm{6}\alpha−\alpha\right)\mathrm{sin}\:\left(\mathrm{6}\alpha+\alpha\right) \\ $$$$=\mathrm{sin}\:\alpha\left\{\mathrm{sin}^{\mathrm{2}} \mathrm{6}\alpha−\mathrm{sin}^{\mathrm{2}} \alpha\right\} \\ $$$$=\mathrm{sin}\frac{\pi}{\mathrm{18}}\left\{\mathrm{sin}^{\mathrm{2}} \frac{\pi}{\mathrm{3}}\:−\mathrm{sin}^{\mathrm{2}} \frac{\pi}{\mathrm{18}}\right\} \\ $$$$=\mathrm{sin}\frac{\pi}{\mathrm{18}}\left\{\frac{\mathrm{3}}{\mathrm{4}}−\mathrm{sin}^{\mathrm{2}} \frac{\pi}{\mathrm{18}}\right\} \\ $$$$=\frac{\mathrm{1}}{\mathrm{4}}\left(\mathrm{3sin}\frac{\pi}{\mathrm{18}}\:−\mathrm{4sin}^{\mathrm{3}} \frac{\pi}{\mathrm{18}}\right) \\ $$$$=\frac{\mathrm{1}}{\mathrm{4}}×\mathrm{sin3}.\frac{\pi}{\mathrm{18}} \\ $$$$=\frac{\mathrm{1}}{\mathrm{4}}×\frac{\mathrm{1}}{\mathrm{2}}=\frac{\mathrm{1}}{\mathrm{8}} \\ $$$$\therefore\mathrm{p}=\frac{\mathrm{1}}{\mathrm{8}}\:\mathrm{ans} \\ $$
Commented by 1549442205PVT last updated on 01/Aug/20

$$\mathrm{Third}\:\mathrm{line}\:\nRightarrow\mathrm{fourth}\:\mathrm{line}\:.\mathrm{Please},\mathrm{sir}\:\mathrm{see} \\ $$$$\mathrm{once}\:\mathrm{again}\:\mathrm{and}\:\mathrm{check}? \\ $$
Commented by bobhans last updated on 01/Aug/20

$$\mathrm{sin}\:\alpha\left\{\mathrm{sin}\:\mathrm{6}\alpha\:\mathrm{cos}\:\alpha−\mathrm{cos}\:\mathrm{6}\alpha\:\mathrm{sin}\:\alpha\right\}\left\{\mathrm{sin}\right. \\ $$$$\left.\mathrm{6}\alpha\:\mathrm{cos}\:\alpha+\mathrm{cos}\:\mathrm{6}\alpha\:\mathrm{sin}\:\alpha\right\}\: \\ $$$$\Leftrightarrow\:\mathrm{sin}\:\alpha\:\left\{\:\left(\mathrm{sin}\:\mathrm{6}\alpha\:\mathrm{cos}\:\alpha\right)^{\mathrm{2}} −\left(\mathrm{cos}\:\mathrm{6}\alpha\:\mathrm{sin}\:\alpha\right)^{\mathrm{2}} \right\} \\ $$$${sorry}\:{sir}.\:{your}\:{answer}\:{something} \\ $$$${wrong} \\ $$
Commented by som(math1967) last updated on 01/Aug/20
![sin(A−B)sin(A+B) =sin^2 A−sin^2 B ∴sin(6α−α)sin (6α+α) =sin^2 6α−sin^2 α α=(π/(18)) ⇒6α=(π/3) ∴sinα(sin^2 6α−sin^2 α) =sinα(sin^2 (π/3) −sin^2 α) =sinα((3/4) −sin^2 α) =sinα(((3−4sin^2 α)/4)) =((3sinα−4sin^3 α)/4) =((sin3α)/4) =((sin(π/6))/4) [∵3α=3×(π/(18))=(π/6)] =(1/4)×(1/2)=(1/8)](Q105829.png)
$$\mathrm{sin}\left(\mathrm{A}−\mathrm{B}\right)\mathrm{sin}\left(\mathrm{A}+\mathrm{B}\right) \\ $$$$=\mathrm{sin}^{\mathrm{2}} \mathrm{A}−\mathrm{sin}^{\mathrm{2}} \mathrm{B} \\ $$$$\therefore\mathrm{sin}\left(\mathrm{6}\alpha−\alpha\right)\mathrm{sin}\:\left(\mathrm{6}\alpha+\alpha\right) \\ $$$$=\mathrm{sin}^{\mathrm{2}} \mathrm{6}\alpha−\mathrm{sin}^{\mathrm{2}} \alpha \\ $$$$\alpha=\frac{\pi}{\mathrm{18}}\:\Rightarrow\mathrm{6}\alpha=\frac{\pi}{\mathrm{3}} \\ $$$$\therefore\mathrm{sin}\alpha\left(\mathrm{sin}^{\mathrm{2}} \mathrm{6}\alpha−\mathrm{sin}^{\mathrm{2}} \alpha\right) \\ $$$$=\mathrm{sin}\alpha\left(\mathrm{sin}^{\mathrm{2}} \frac{\pi}{\mathrm{3}}\:−\mathrm{sin}^{\mathrm{2}} \alpha\right) \\ $$$$=\mathrm{sin}\alpha\left(\frac{\mathrm{3}}{\mathrm{4}}\:−\mathrm{sin}^{\mathrm{2}} \alpha\right) \\ $$$$=\mathrm{sin}\alpha\left(\frac{\mathrm{3}−\mathrm{4sin}^{\mathrm{2}} \alpha}{\mathrm{4}}\right) \\ $$$$=\frac{\mathrm{3sin}\alpha−\mathrm{4sin}^{\mathrm{3}} \alpha}{\mathrm{4}} \\ $$$$=\frac{\mathrm{sin3}\alpha}{\mathrm{4}} \\ $$$$=\frac{\mathrm{sin}\frac{\pi}{\mathrm{6}}}{\mathrm{4}}\:\:\:\:\left[\because\mathrm{3}\alpha=\mathrm{3}×\frac{\pi}{\mathrm{18}}=\frac{\pi}{\mathrm{6}}\right] \\ $$$$=\frac{\mathrm{1}}{\mathrm{4}}×\frac{\mathrm{1}}{\mathrm{2}}=\frac{\mathrm{1}}{\mathrm{8}} \\ $$
Commented by som(math1967) last updated on 01/Aug/20
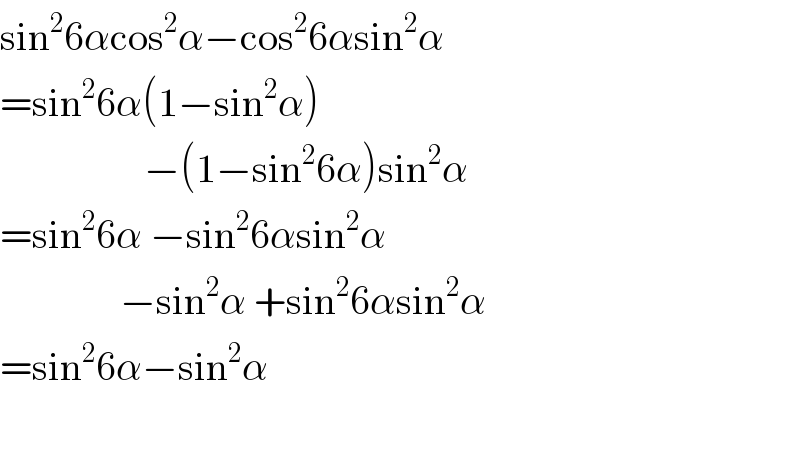
$$\mathrm{sin}^{\mathrm{2}} \mathrm{6}\alpha\mathrm{cos}^{\mathrm{2}} \alpha−\mathrm{cos}^{\mathrm{2}} \mathrm{6}\alpha\mathrm{sin}^{\mathrm{2}} \alpha \\ $$$$=\mathrm{sin}^{\mathrm{2}} \mathrm{6}\alpha\left(\mathrm{1}−\mathrm{sin}^{\mathrm{2}} \alpha\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:−\left(\mathrm{1}−\mathrm{sin}^{\mathrm{2}} \mathrm{6}\alpha\right)\mathrm{sin}^{\mathrm{2}} \alpha \\ $$$$=\mathrm{sin}^{\mathrm{2}} \mathrm{6}\alpha\:−\mathrm{sin}^{\mathrm{2}} \mathrm{6}\alpha\mathrm{sin}^{\mathrm{2}} \alpha \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:−\mathrm{sin}^{\mathrm{2}} \alpha\:+\mathrm{sin}^{\mathrm{2}} \mathrm{6}\alpha\mathrm{sin}^{\mathrm{2}} \alpha \\ $$$$=\mathrm{sin}^{\mathrm{2}} \mathrm{6}\alpha−\mathrm{sin}^{\mathrm{2}} \alpha \\ $$$$ \\ $$
Commented by bobhans last updated on 01/Aug/20

$$\mathrm{sin}\:\left({A}−{B}\right)\mathrm{sin}\:\left({A}+{B}\right)\:=\:−\frac{\mathrm{1}}{\mathrm{2}}\left(−\mathrm{2sin}\left({A}−{B}\right)\mathrm{sin}\:\left({A}+{B}\right)\right) \\ $$$$−\frac{\mathrm{1}}{\mathrm{2}}\left(\mathrm{cos}\:\mathrm{2}{A}−\mathrm{cos}\:\mathrm{2}{B}\:\right)\:=\:−\frac{\mathrm{1}}{\mathrm{2}}\left(\mathrm{1}−\mathrm{2sin}\:^{\mathrm{2}} {A}−\left(\mathrm{1}−\mathrm{2sin}\:^{\mathrm{2}} {B}\right)\right) \\ $$$$=\:−\frac{\mathrm{1}}{\mathrm{2}}\left(−\mathrm{2sin}\:^{\mathrm{2}} {A}+\mathrm{2sin}\:^{\mathrm{2}} {B}\right) \\ $$$$=\:\mathrm{sin}\:^{\mathrm{2}} {A}−\mathrm{sin}\:^{\mathrm{2}} {B}.\: \\ $$$${hahaha}..{yes}\:{sir}\:.\:{you}\:{are}\:{right} \\ $$
Commented by 1549442205PVT last updated on 03/Aug/20

$$\mathrm{Thank}\:\mathrm{both}\:\mathrm{sirs}. \\ $$
Commented by som(math1967) last updated on 01/Aug/20

$$\mathrm{Welcome}\: \\ $$$$ \\ $$
