
Question Number 105243 by bemath last updated on 27/Jul/20
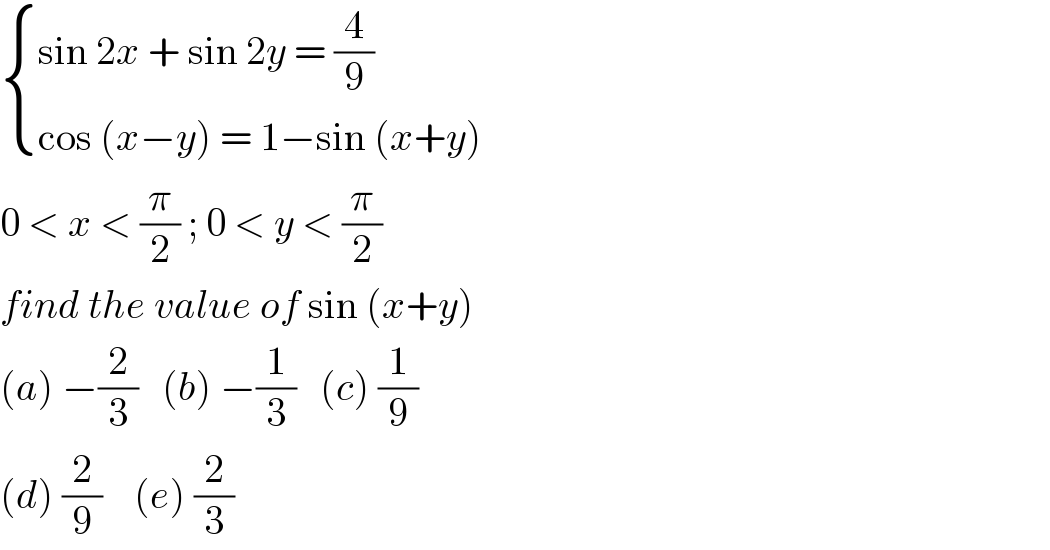
$$\begin{cases}{\mathrm{sin}\:\mathrm{2}{x}\:+\:\mathrm{sin}\:\mathrm{2}{y}\:=\:\frac{\mathrm{4}}{\mathrm{9}}}\\{\mathrm{cos}\:\left({x}−{y}\right)\:=\:\mathrm{1}−\mathrm{sin}\:\left({x}+{y}\right)}\end{cases} \\ $$ $$\mathrm{0}\:<\:{x}\:<\:\frac{\pi}{\mathrm{2}}\:;\:\mathrm{0}\:<\:{y}\:<\:\frac{\pi}{\mathrm{2}} \\ $$ $${find}\:{the}\:{value}\:{of}\:\mathrm{sin}\:\left({x}+{y}\right) \\ $$ $$\left({a}\right)\:−\frac{\mathrm{2}}{\mathrm{3}}\:\:\:\left({b}\right)\:−\frac{\mathrm{1}}{\mathrm{3}}\:\:\:\left({c}\right)\:\frac{\mathrm{1}}{\mathrm{9}} \\ $$ $$\left({d}\right)\:\frac{\mathrm{2}}{\mathrm{9}}\:\:\:\:\left({e}\right)\:\frac{\mathrm{2}}{\mathrm{3}} \\ $$
Answered by bramlex last updated on 27/Jul/20
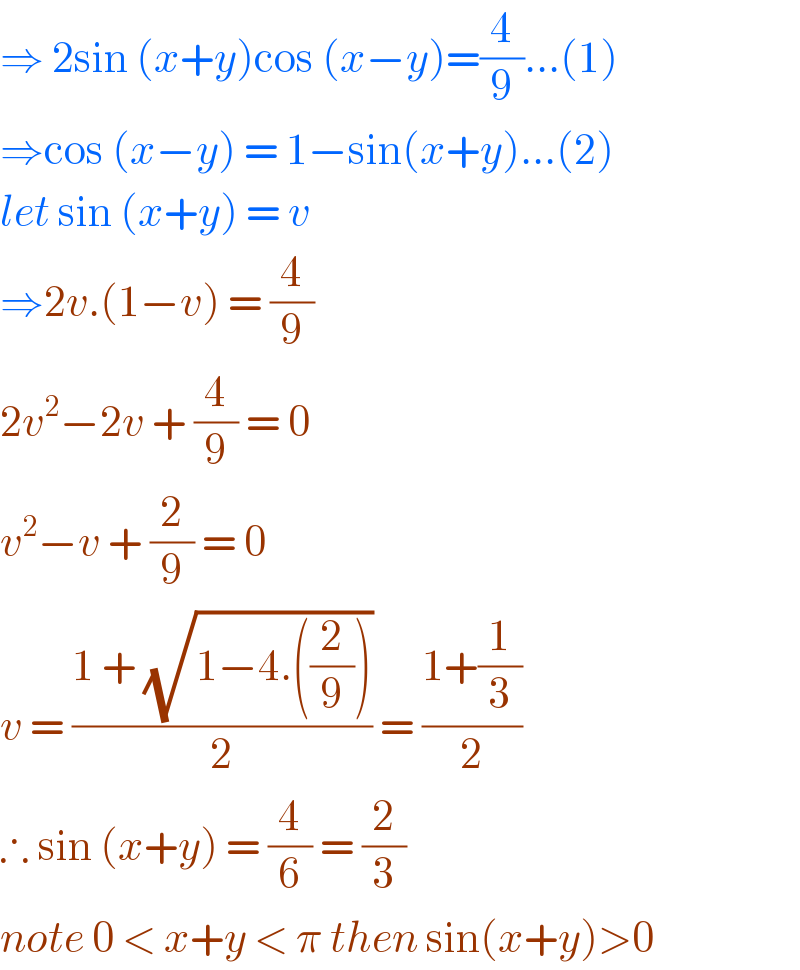
$$\Rightarrow\:\mathrm{2sin}\:\left({x}+{y}\right)\mathrm{cos}\:\left({x}−{y}\right)=\frac{\mathrm{4}}{\mathrm{9}}...\left(\mathrm{1}\right) \\ $$ $$\Rightarrow\mathrm{cos}\:\left({x}−{y}\right)\:=\:\mathrm{1}−\mathrm{sin}\left({x}+{y}\right)...\left(\mathrm{2}\right) \\ $$ $${let}\:\mathrm{sin}\:\left({x}+{y}\right)\:=\:{v} \\ $$ $$\Rightarrow\mathrm{2}{v}.\left(\mathrm{1}−{v}\right)\:=\:\frac{\mathrm{4}}{\mathrm{9}} \\ $$ $$\mathrm{2}{v}^{\mathrm{2}} −\mathrm{2}{v}\:+\:\frac{\mathrm{4}}{\mathrm{9}}\:=\:\mathrm{0} \\ $$ $${v}^{\mathrm{2}} −{v}\:+\:\frac{\mathrm{2}}{\mathrm{9}}\:=\:\mathrm{0} \\ $$ $${v}\:=\:\frac{\mathrm{1}\:+\:\sqrt{\mathrm{1}−\mathrm{4}.\left(\frac{\mathrm{2}}{\mathrm{9}}\right)}}{\mathrm{2}}\:=\:\frac{\mathrm{1}+\frac{\mathrm{1}}{\mathrm{3}}}{\mathrm{2}} \\ $$ $$\therefore\:\mathrm{sin}\:\left({x}+{y}\right)\:=\:\frac{\mathrm{4}}{\mathrm{6}}\:=\:\frac{\mathrm{2}}{\mathrm{3}} \\ $$ $${note}\:\mathrm{0}\:<\:{x}+{y}\:<\:\pi\:{then}\:\mathrm{sin}\left({x}+{y}\right)>\mathrm{0} \\ $$
Commented bybemath last updated on 27/Jul/20

$${afdolll} \\ $$
Answered by Dwaipayan Shikari last updated on 27/Jul/20
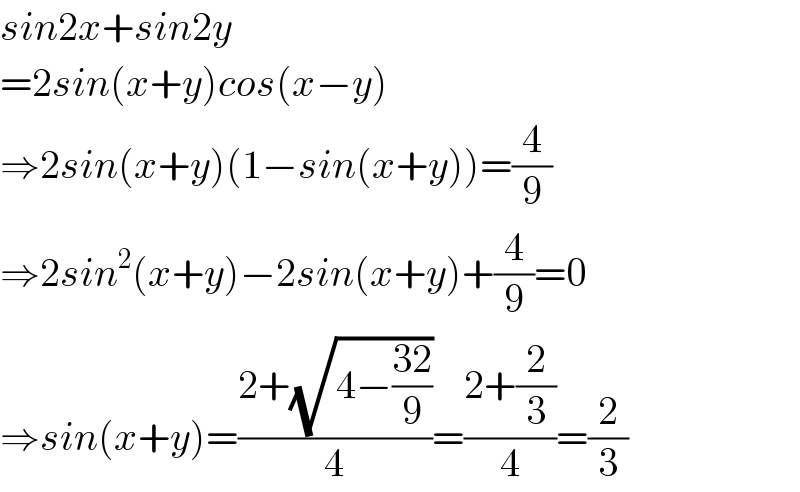
$${sin}\mathrm{2}{x}+{sin}\mathrm{2}{y} \\ $$ $$=\mathrm{2}{sin}\left({x}+{y}\right){cos}\left({x}−{y}\right) \\ $$ $$\Rightarrow\mathrm{2}{sin}\left({x}+{y}\right)\left(\mathrm{1}−{sin}\left({x}+{y}\right)\right)=\frac{\mathrm{4}}{\mathrm{9}} \\ $$ $$\Rightarrow\mathrm{2}{sin}^{\mathrm{2}} \left({x}+{y}\right)−\mathrm{2}{sin}\left({x}+{y}\right)+\frac{\mathrm{4}}{\mathrm{9}}=\mathrm{0} \\ $$ $$\Rightarrow{sin}\left({x}+{y}\right)=\frac{\mathrm{2}+\sqrt{\mathrm{4}−\frac{\mathrm{32}}{\mathrm{9}}}}{\mathrm{4}}=\frac{\mathrm{2}+\frac{\mathrm{2}}{\mathrm{3}}}{\mathrm{4}}=\frac{\mathrm{2}}{\mathrm{3}} \\ $$
