Question and Answers Forum

All Questions      Topic List

Others Questions

Previous in All Question      Next in All Question      

Previous in Others      Next in Others      

Question Number 104940 by 175mohamed last updated on 24/Jul/20

Commented by bramlex last updated on 25/Jul/20

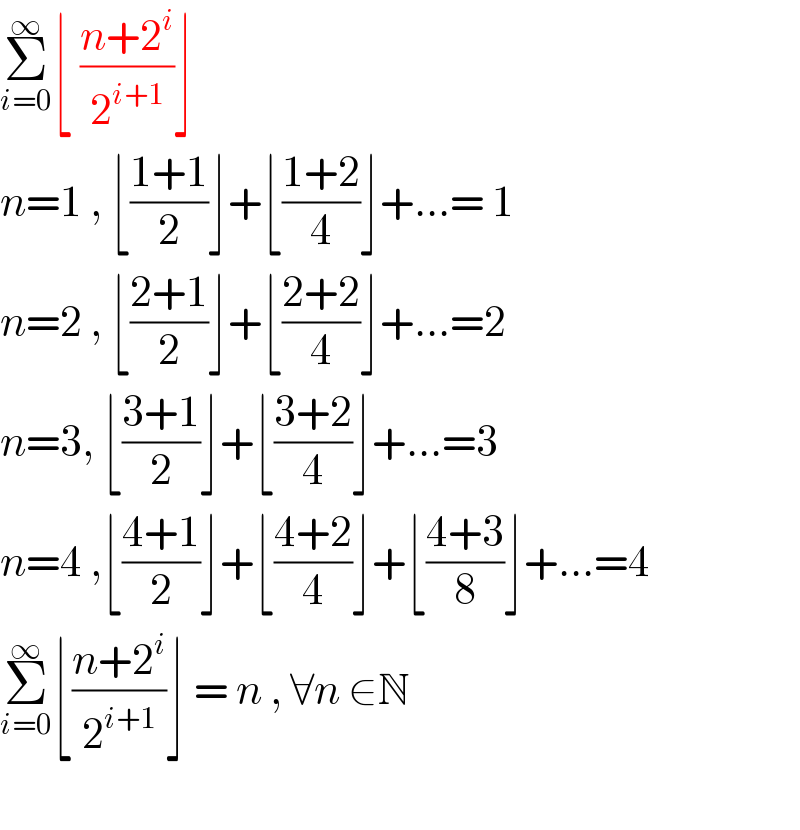
Σ_(i=0) ^∞ ⌊ ((n+2^i )/2^(i+1) )⌋ n=1 , ⌊((1+1)/2)⌋+⌊((1+2)/4)⌋+...= 1 n=2 , ⌊((2+1)/2)⌋+⌊((2+2)/4)⌋+...=2 n=3, ⌊((3+1)/2)⌋+⌊((3+2)/4)⌋+...=3 n=4 ,⌊((4+1)/2)⌋+⌊((4+2)/4)⌋+⌊((4+3)/8)⌋+...=4 Σ_(i=0) ^∞ ⌊((n+2^i )/2^(i+1) )⌋ = n , ∀n ∈N

$$\underset{{i}=\mathrm{0}} {\overset{\infty} {\sum}}\lfloor\:\frac{{n}+\mathrm{2}^{{i}} }{\mathrm{2}^{{i}+\mathrm{1}} }\rfloor\: \\ $$$${n}=\mathrm{1}\:,\:\lfloor\frac{\mathrm{1}+\mathrm{1}}{\mathrm{2}}\rfloor+\lfloor\frac{\mathrm{1}+\mathrm{2}}{\mathrm{4}}\rfloor+...=\:\mathrm{1} \\ $$$${n}=\mathrm{2}\:,\:\lfloor\frac{\mathrm{2}+\mathrm{1}}{\mathrm{2}}\rfloor+\lfloor\frac{\mathrm{2}+\mathrm{2}}{\mathrm{4}}\rfloor+...=\mathrm{2} \\ $$$${n}=\mathrm{3},\:\lfloor\frac{\mathrm{3}+\mathrm{1}}{\mathrm{2}}\rfloor+\lfloor\frac{\mathrm{3}+\mathrm{2}}{\mathrm{4}}\rfloor+...=\mathrm{3} \\ $$$${n}=\mathrm{4}\:,\lfloor\frac{\mathrm{4}+\mathrm{1}}{\mathrm{2}}\rfloor+\lfloor\frac{\mathrm{4}+\mathrm{2}}{\mathrm{4}}\rfloor+\lfloor\frac{\mathrm{4}+\mathrm{3}}{\mathrm{8}}\rfloor+...=\mathrm{4} \\ $$$$\underset{{i}=\mathrm{0}} {\overset{\infty} {\sum}}\lfloor\frac{{n}+\mathrm{2}^{{i}} }{\mathrm{2}^{{i}+\mathrm{1}} }\rfloor\:=\:{n}\:,\:\forall{n}\:\in\mathbb{N} \\ $$$$ \\ $$

Answered by Ar Brandon last updated on 25/Jul/20

A_i =Σ_(i=0) ^∞ [((n+2^i )/2^(i+1) )]=Σ_(i=0) ^∞ {(n/2^(i+1) )+(1/2)} =(n/2)Σ_(i=0) ^∞ (1/2^i )+Σ_(i=0) ^∞ (1/2)

$$\mathrm{A}_{{i}} =\underset{{i}=\mathrm{0}} {\overset{\infty} {\sum}}\left[\frac{\mathrm{n}+\mathrm{2}^{{i}} }{\mathrm{2}^{{i}+\mathrm{1}} }\right]=\underset{{i}=\mathrm{0}} {\overset{\infty} {\sum}}\left\{\frac{\mathrm{n}}{\mathrm{2}^{{i}+\mathrm{1}} }+\frac{\mathrm{1}}{\mathrm{2}}\right\} \\ $$$$\:\:\:\:\:=\frac{\mathrm{n}}{\mathrm{2}}\underset{{i}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{\mathrm{2}^{{i}} }+\underset{{i}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{\mathrm{2}} \\ $$

Commented by mathmax by abdo last updated on 25/Jul/20

no sir [...] mean integr parts(floor) you answer another question..!

$$\mathrm{no}\:\mathrm{sir}\:\:\left[...\right]\:\mathrm{mean}\:\mathrm{integr}\:\mathrm{parts}\left(\mathrm{floor}\right)\:\mathrm{you}\:\mathrm{answer}\:\mathrm{another}\:\mathrm{question}..! \\ $$

Commented by Ar Brandon last updated on 25/Jul/20

Oh ! OK Thanks

Terms of Service

Privacy Policy

Contact: info@tinkutara.com