Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 104459 by bemath last updated on 21/Jul/20

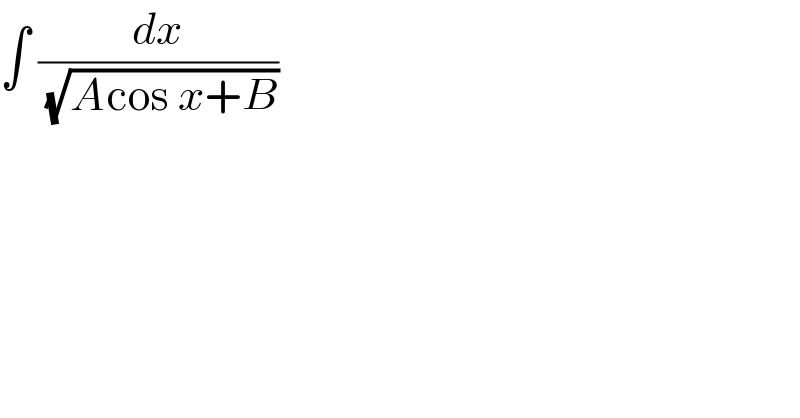
∫ (dx/(√(Acos x+B)))

$$\int\:\frac{{dx}}{\sqrt{{A}\mathrm{cos}\:{x}+{B}}} \\ $$

Commented by Dwaipayan Shikari last updated on 21/Jul/20

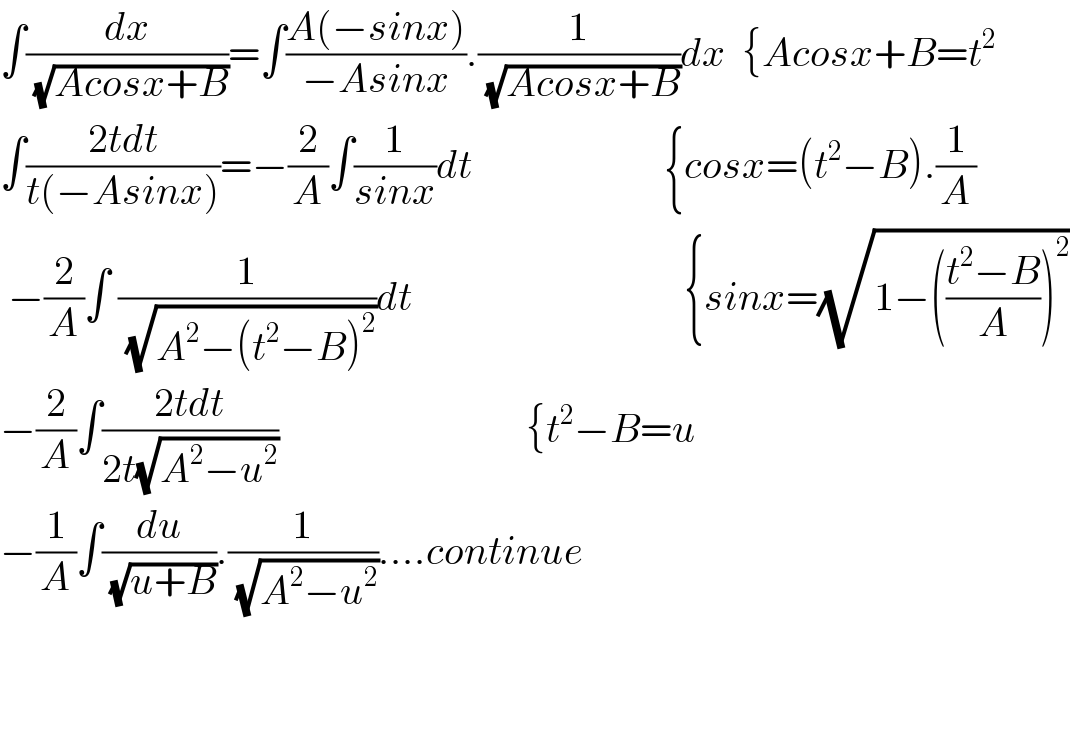
∫(dx/(√(Acosx+B)))=∫((A(−sinx))/(−Asinx)).(1/(√(Acosx+B)))dx {Acosx+B=t^2 ∫((2tdt)/(t(−Asinx)))=−(2/A)∫(1/(sinx))dt {cosx=(t^2 −B).(1/A) −(2/A)∫ (1/(√(A^2 −(t^2 −B)^2 )))dt {sinx=(√(1−(((t^2 −B)/A))^2 )) −(2/A)∫((2tdt)/(2t(√(A^2 −u^2 )))) {t^2 −B=u −(1/A)∫(du/(√(u+B))).(1/(√(A^2 −u^2 )))....continue

$$\int\frac{{dx}}{\sqrt{{Acosx}+{B}}}=\int\frac{{A}\left(−{sinx}\right)}{−{Asinx}}.\frac{\mathrm{1}}{\sqrt{{Acosx}+{B}}}{dx}\:\:\left\{{Acosx}+{B}={t}^{\mathrm{2}} \right. \\ $$$$\int\frac{\mathrm{2}{tdt}}{{t}\left(−{Asinx}\right)}=−\frac{\mathrm{2}}{{A}}\int\frac{\mathrm{1}}{{sinx}}{dt}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left\{{cosx}=\left({t}^{\mathrm{2}} −{B}\right).\frac{\mathrm{1}}{{A}}\right. \\ $$$$\:−\frac{\mathrm{2}}{{A}}\int\:\frac{\mathrm{1}}{\sqrt{{A}^{\mathrm{2}} −\left({t}^{\mathrm{2}} −{B}\right)^{\mathrm{2}} }}{dt}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left\{{sinx}=\sqrt{\mathrm{1}−\left(\frac{{t}^{\mathrm{2}} −{B}}{{A}}\right)^{\mathrm{2}} }\right. \\ $$$$−\frac{\mathrm{2}}{{A}}\int\frac{\mathrm{2}{tdt}}{\mathrm{2}{t}\sqrt{{A}^{\mathrm{2}} −{u}^{\mathrm{2}} }}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left\{{t}^{\mathrm{2}} −{B}={u}\right. \\ $$$$−\frac{\mathrm{1}}{{A}}\int\frac{{du}}{\sqrt{{u}+{B}}}.\frac{\mathrm{1}}{\sqrt{{A}^{\mathrm{2}} −{u}^{\mathrm{2}} }}....{continue} \\ $$$$ \\ $$$$ \\ $$

Answered by bobhans last updated on 21/Jul/20

I = ∫ (dx/(√(Acos x+B))) . let x = π−t I = ∫ ((−dt)/((√(−Acos t+B)) )) = ∫ ((−dx)/(√(−Acos x+B))) 2I = ∫ (1/(√(Acos x+B))) −(1/(√(−Acos x+B))) dx 2I= ∫ (((√(B−Acos x))−(√(B+Acos x)))/(√(B^2 −A^2 cos^2 x))) dx

$${I}\:=\:\int\:\frac{{dx}}{\sqrt{{A}\mathrm{cos}\:{x}+{B}}}\:.\:{let}\:{x}\:=\:\pi−{t}\: \\ $$$${I}\:=\:\int\:\frac{−{dt}}{\sqrt{−{A}\mathrm{cos}\:{t}+{B}}\:}\:=\:\int\:\frac{−{dx}}{\sqrt{−{A}\mathrm{cos}\:{x}+{B}}} \\ $$$$\mathrm{2}{I}\:=\:\int\:\frac{\mathrm{1}}{\sqrt{{A}\mathrm{cos}\:{x}+{B}}}\:−\frac{\mathrm{1}}{\sqrt{−{A}\mathrm{cos}\:{x}+{B}}}\:{dx} \\ $$$$\mathrm{2}{I}=\:\int\:\frac{\sqrt{{B}−{A}\mathrm{cos}\:{x}}−\sqrt{{B}+{A}\mathrm{cos}\:{x}}}{\sqrt{{B}^{\mathrm{2}} −{A}^{\mathrm{2}} \mathrm{cos}\:^{\mathrm{2}} {x}}}\:{dx} \\ $$$$ \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com