
Previous in Differential Equation Next in Differential Equation
Question Number 100323 by bobhans last updated on 26/Jun/20

$$\mathbb{S}\mathrm{olve}\:\mathrm{x}^{\mathrm{2}} \mathrm{y}''+\mathrm{2xy}'−\mathrm{2y}=\mathrm{0} \\ $$
Commented by bemath last updated on 26/Jun/20

$$\mathrm{since}\:\mathrm{diff}\:\mathrm{a}\:\mathrm{power}\:\mathrm{pushes}\:\mathrm{down}\:\mathrm{the} \\ $$$$\mathrm{exponent}\:\mathrm{by}\:\mathrm{one}\:\mathrm{unit},\:\mathrm{the}\:\mathrm{form}\:\mathrm{of} \\ $$$$\mathrm{this}\:\mathrm{eq}\:\mathrm{suggest}\:\mathrm{that}\:\mathrm{we}\:\mathrm{look}\:\mathrm{for} \\ $$$$\mathrm{possible}\:\mathrm{solutions}\:\mathrm{of}\:\mathrm{the}\:\mathrm{type}\:\mathrm{y}=\mathrm{x}^{\mathrm{n}} . \\ $$$$\mathrm{we}\:\mathrm{optain}\:\mathrm{the}\:\mathrm{quadratic}\:\mathrm{eq} \\ $$$$\mathrm{n}\left(\mathrm{n}−\mathrm{1}\right)+\mathrm{2n}−\mathrm{2}=\mathrm{0},\:\mathrm{this}\:\mathrm{has}\:\mathrm{roots} \\ $$$$\mathrm{n}=\mathrm{1};\:−\mathrm{2}\:\mathrm{so}\:\mathrm{are}\:\mathrm{solutions}\:\mathrm{is}\: \\ $$$$\mathrm{y}\:=\:\mathrm{C}_{\mathrm{1}} {x}\:+\:\mathrm{C}_{\mathrm{2}} {x}^{−\mathrm{2}} \:\bigstar\: \\ $$
Answered by mathmax by abdo last updated on 27/Jun/20
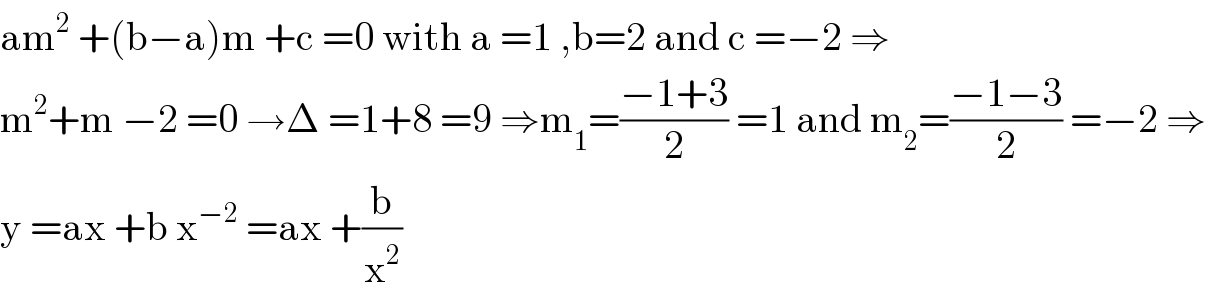
$$\mathrm{am}^{\mathrm{2}} \:+\left(\mathrm{b}−\mathrm{a}\right)\mathrm{m}\:+\mathrm{c}\:=\mathrm{0}\:\mathrm{with}\:\mathrm{a}\:=\mathrm{1}\:,\mathrm{b}=\mathrm{2}\:\mathrm{and}\:\mathrm{c}\:=−\mathrm{2}\:\Rightarrow \\ $$$$\mathrm{m}^{\mathrm{2}} +\mathrm{m}\:−\mathrm{2}\:=\mathrm{0}\:\rightarrow\Delta\:=\mathrm{1}+\mathrm{8}\:=\mathrm{9}\:\Rightarrow\mathrm{m}_{\mathrm{1}} =\frac{−\mathrm{1}+\mathrm{3}}{\mathrm{2}}\:=\mathrm{1}\:\mathrm{and}\:\mathrm{m}_{\mathrm{2}} =\frac{−\mathrm{1}−\mathrm{3}}{\mathrm{2}}\:=−\mathrm{2}\:\Rightarrow \\ $$$$\mathrm{y}\:=\mathrm{ax}\:+\mathrm{b}\:\mathrm{x}^{−\mathrm{2}} \:=\mathrm{ax}\:+\frac{\mathrm{b}}{\mathrm{x}^{\mathrm{2}} } \\ $$
Commented by mathmax by abdo last updated on 27/Jun/20
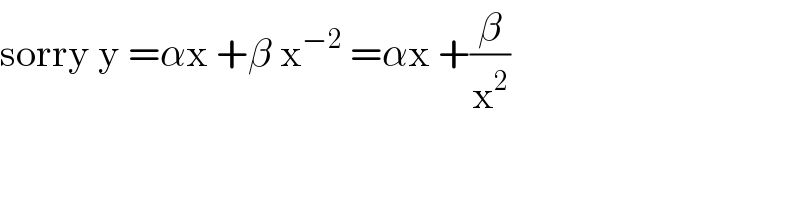
$$\mathrm{sorry}\:\mathrm{y}\:=\alpha\mathrm{x}\:+\beta\:\mathrm{x}^{−\mathrm{2}} \:=\alpha\mathrm{x}\:+\frac{\beta}{\mathrm{x}^{\mathrm{2}} } \\ $$
