
Question Number 6885 by Tawakalitu. last updated on 01/Aug/16
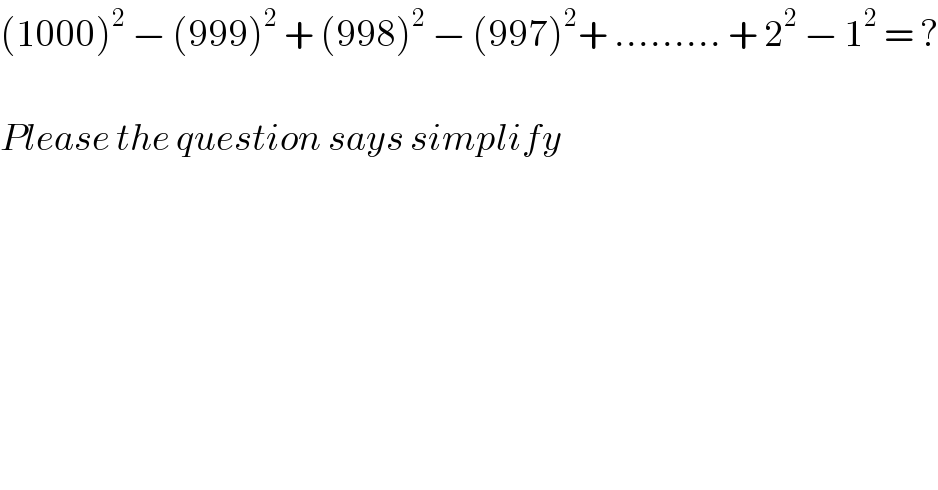
$$\left(\mathrm{1000}\right)^{\mathrm{2}} \:−\:\left(\mathrm{999}\right)^{\mathrm{2}} \:+\:\left(\mathrm{998}\right)^{\mathrm{2}} \:−\:\left(\mathrm{997}\right)^{\mathrm{2}} +\:.........\:+\:\mathrm{2}^{\mathrm{2}} \:−\:\mathrm{1}^{\mathrm{2}} \:=\:? \\ $$$$ \\ $$$${Please}\:{the}\:{question}\:{says}\:{simplify} \\ $$
Answered by sou1618 last updated on 01/Aug/16

$${S}=\left(\mathrm{1000}^{\mathrm{2}} −\mathrm{999}^{\mathrm{2}} \right)+...+\left\{\left(\mathrm{2}{k}\right)^{\mathrm{2}} −\left(\mathrm{2}{k}−\mathrm{1}\right)^{\mathrm{2}} \right\}+...+\left(\mathrm{2}^{\mathrm{2}} −\mathrm{1}^{\mathrm{2}} \right) \\ $$$$\mathrm{1}<={k}<=\mathrm{500} \\ $$$${S}=\left(\mathrm{1000}+\mathrm{999}\right)\left(\mathrm{1000}−\mathrm{999}\right)+...+\left(\mathrm{2}{k}+\mathrm{2}{k}−\mathrm{1}\right)\left(\mathrm{2}{k}−\mathrm{2}{k}+\mathrm{1}\right)+...+\left(\mathrm{2}+\mathrm{1}\right)\left(\mathrm{2}−\mathrm{1}\right) \\ $$$${S}=\mathrm{1999}×\mathrm{1}+...+\left(\mathrm{4}{k}−\mathrm{1}\right)×\mathrm{1}+...+\mathrm{3}×\mathrm{1} \\ $$$${S}=\mathrm{1999}+\mathrm{1995}+...+\left(\mathrm{4}{k}−\mathrm{1}\right)+...+\mathrm{7}+\mathrm{3} \\ $$$$\left({i}\right) \\ $$$${S}=\underset{{k}=\mathrm{1}} {\overset{\mathrm{500}} {\sum}}\left(\mathrm{4}{k}−\mathrm{1}\right) \\ $$$${S}=\mathrm{2}×\mathrm{500}×\left(\mathrm{500}+\mathrm{1}\right)−\mathrm{500} \\ $$$${S}=\mathrm{501000}−\mathrm{500} \\ $$$${S}=\mathrm{500500} \\ $$$$ \\ $$$$\left({ii}\right) \\ $$$${S}=\left(\mathrm{1999}+\mathrm{3}\right)+\left(\mathrm{1995}+\mathrm{7}\right)+......+\left(\mathrm{1003}+\mathrm{999}\right) \\ $$$$\begin{cases}{\mathrm{1003}=\mathrm{4}×\mathrm{251}−\mathrm{1}}\\{\mathrm{999}=\mathrm{4}×\mathrm{250}−\mathrm{1}}\end{cases} \\ $$$${S}=\mathrm{2002}+\mathrm{2002}+....+\mathrm{2002}\left(\mathrm{250}{times}\right) \\ $$$$\begin{cases}{\mathrm{1999}=\mathrm{4}×\mathrm{500}−\mathrm{1}}\\{\mathrm{1003}=\mathrm{4}×\mathrm{251}−\mathrm{1}}\end{cases} \\ $$$${S}=\mathrm{2002}×\mathrm{250} \\ $$$${S}=\mathrm{500500} \\ $$
Commented by sou1618 last updated on 01/Aug/16

$$\left({iii}\right){other}\:{way} \\ $$$${S}=\underset{{k}=\mathrm{1}} {\overset{\mathrm{500}} {\sum}}\left(\mathrm{2}{k}\right)^{\mathrm{2}} −\underset{{k}=\mathrm{1}} {\overset{\mathrm{500}} {\sum}}\left(\mathrm{2}{k}−\mathrm{1}\right)^{\mathrm{2}} \\ $$$${S}=\underset{{k}=\mathrm{1}} {\overset{\mathrm{500}} {\sum}}\left\{\mathrm{4}{k}^{\mathrm{2}} −\left(\mathrm{4}{k}^{\mathrm{2}} −\mathrm{4}{k}+\mathrm{1}\right)\right\} \\ $$$${S}=\underset{{k}=\mathrm{1}} {\overset{\mathrm{500}} {\sum}}\left(\mathrm{4}{k}−\mathrm{1}\right) \\ $$$${S}=\mathrm{2}×\mathrm{500}×\mathrm{501}−\mathrm{500} \\ $$$${S}=\mathrm{500500} \\ $$$$ \\ $$$$ \\ $$$$ \\ $$
Commented by Tawakalitu. last updated on 01/Aug/16
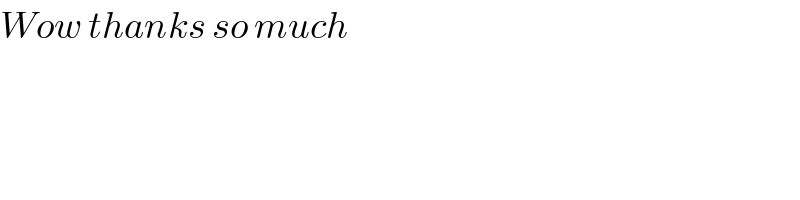
$${Wow}\:{thanks}\:{so}\:{much} \\ $$
Commented by Tawakalitu. last updated on 01/Aug/16
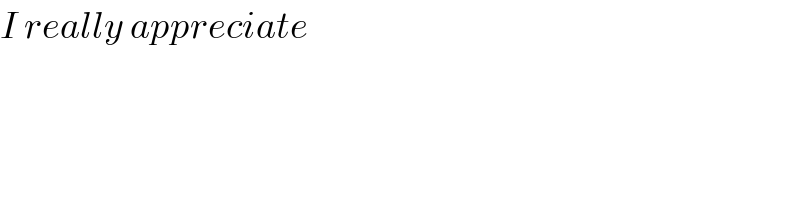
$${I}\:{really}\:{appreciate} \\ $$
