Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 117342 by bemath last updated on 11/Oct/20

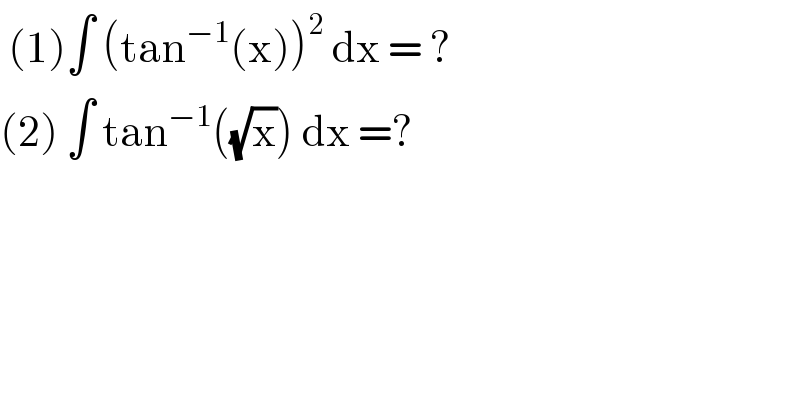
(1)∫ (tan^(−1) (x))^2 dx = ? (2) ∫ tan^(−1) ((√x)) dx =?

$$\:\left(\mathrm{1}\right)\int\:\left(\mathrm{tan}^{−\mathrm{1}} \left(\mathrm{x}\right)\right)^{\mathrm{2}} \:\mathrm{dx}\:=\:? \\ $$$$\left(\mathrm{2}\right)\:\int\:\mathrm{tan}^{−\mathrm{1}} \left(\sqrt{\mathrm{x}}\right)\:\mathrm{dx}\:=? \\ $$

Answered by mathmax by abdo last updated on 11/Oct/20

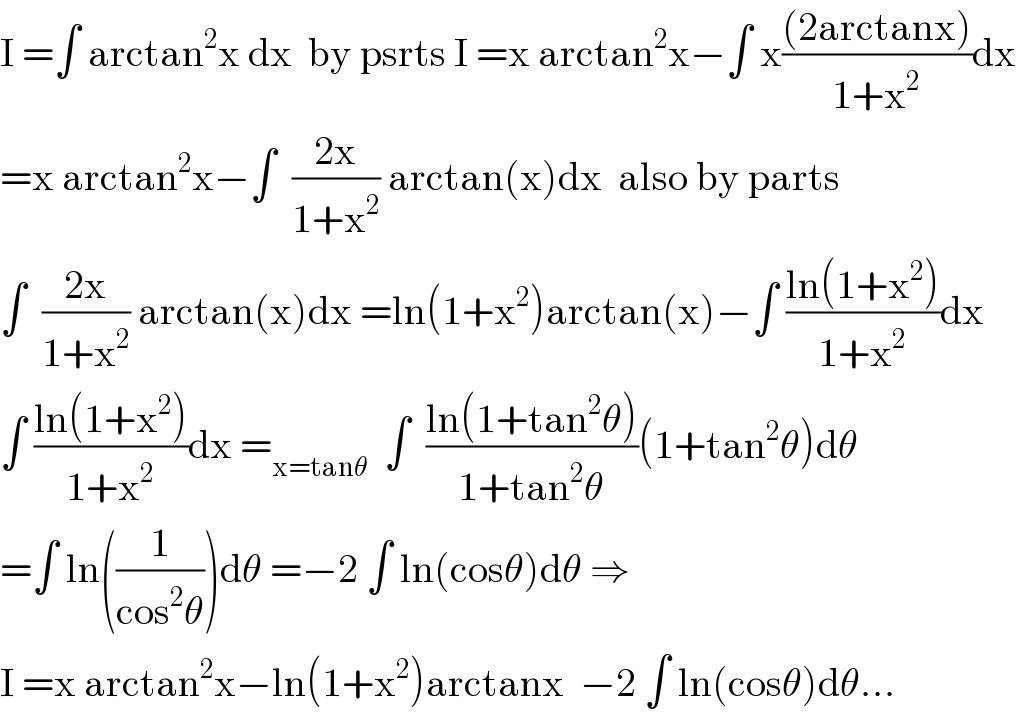
I =∫ arctan^2 x dx by psrts I =x arctan^2 x−∫ x(((2arctanx))/(1+x^2 ))dx =x arctan^2 x−∫ ((2x)/(1+x^2 )) arctan(x)dx also by parts ∫ ((2x)/(1+x^2 )) arctan(x)dx =ln(1+x^2 )arctan(x)−∫ ((ln(1+x^2 ))/(1+x^2 ))dx ∫ ((ln(1+x^2 ))/(1+x^2 ))dx =_(x=tanθ) ∫ ((ln(1+tan^2 θ))/(1+tan^2 θ))(1+tan^2 θ)dθ =∫ ln((1/(cos^2 θ)))dθ =−2 ∫ ln(cosθ)dθ ⇒ I =x arctan^2 x−ln(1+x^2 )arctanx −2 ∫ ln(cosθ)dθ...

$$\mathrm{I}\:=\int\:\mathrm{arctan}^{\mathrm{2}} \mathrm{x}\:\mathrm{dx}\:\:\mathrm{by}\:\mathrm{psrts}\:\mathrm{I}\:=\mathrm{x}\:\mathrm{arctan}^{\mathrm{2}} \mathrm{x}−\int\:\mathrm{x}\frac{\left(\mathrm{2arctanx}\right)}{\mathrm{1}+\mathrm{x}^{\mathrm{2}} }\mathrm{dx} \\ $$$$=\mathrm{x}\:\mathrm{arctan}^{\mathrm{2}} \mathrm{x}−\int\:\:\frac{\mathrm{2x}}{\mathrm{1}+\mathrm{x}^{\mathrm{2}} }\:\mathrm{arctan}\left(\mathrm{x}\right)\mathrm{dx}\:\:\mathrm{also}\:\mathrm{by}\:\mathrm{parts} \\ $$$$\int\:\:\frac{\mathrm{2x}}{\mathrm{1}+\mathrm{x}^{\mathrm{2}} }\:\mathrm{arctan}\left(\mathrm{x}\right)\mathrm{dx}\:=\mathrm{ln}\left(\mathrm{1}+\mathrm{x}^{\mathrm{2}} \right)\mathrm{arctan}\left(\mathrm{x}\right)−\int\:\frac{\mathrm{ln}\left(\mathrm{1}+\mathrm{x}^{\mathrm{2}} \right)}{\mathrm{1}+\mathrm{x}^{\mathrm{2}} }\mathrm{dx} \\ $$$$\int\:\frac{\mathrm{ln}\left(\mathrm{1}+\mathrm{x}^{\mathrm{2}} \right)}{\mathrm{1}+\mathrm{x}^{\mathrm{2}} }\mathrm{dx}\:=_{\mathrm{x}=\mathrm{tan}\theta} \:\:\int\:\:\frac{\mathrm{ln}\left(\mathrm{1}+\mathrm{tan}^{\mathrm{2}} \theta\right)}{\mathrm{1}+\mathrm{tan}^{\mathrm{2}} \theta}\left(\mathrm{1}+\mathrm{tan}^{\mathrm{2}} \theta\right)\mathrm{d}\theta \\ $$$$=\int\:\mathrm{ln}\left(\frac{\mathrm{1}}{\mathrm{cos}^{\mathrm{2}} \theta}\right)\mathrm{d}\theta\:=−\mathrm{2}\:\int\:\mathrm{ln}\left(\mathrm{cos}\theta\right)\mathrm{d}\theta\:\Rightarrow \\ $$$$\mathrm{I}\:=\mathrm{x}\:\mathrm{arctan}^{\mathrm{2}} \mathrm{x}−\mathrm{ln}\left(\mathrm{1}+\mathrm{x}^{\mathrm{2}} \right)\mathrm{arctanx}\:\:−\mathrm{2}\:\int\:\mathrm{ln}\left(\mathrm{cos}\theta\right)\mathrm{d}\theta... \\ $$

Commented by mathmax by abdo last updated on 11/Oct/20

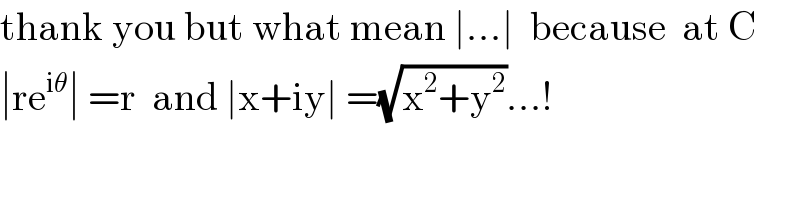
thank you but what mean ∣...∣ because at C ∣re^(iθ) ∣ =r and ∣x+iy∣ =(√(x^2 +y^2 ))...!

$$\mathrm{thank}\:\mathrm{you}\:\mathrm{but}\:\mathrm{what}\:\mathrm{mean}\:\mid...\mid\:\:\mathrm{because}\:\:\mathrm{at}\:\mathrm{C} \\ $$$$\mid\mathrm{re}^{\mathrm{i}\theta} \mid\:=\mathrm{r}\:\:\mathrm{and}\:\mid\mathrm{x}+\mathrm{iy}\mid\:=\sqrt{\mathrm{x}^{\mathrm{2}} +\mathrm{y}^{\mathrm{2}} }...! \\ $$

Commented by bemath last updated on 11/Oct/20

the last term ∫ ln (cos θ) dθ =?

$$\mathrm{the}\:\mathrm{last}\:\mathrm{term}\: \\ $$$$\int\:\mathrm{ln}\:\left(\mathrm{cos}\:\theta\right)\:\mathrm{d}\theta\:=? \\ $$

Commented by Lordose last updated on 11/Oct/20

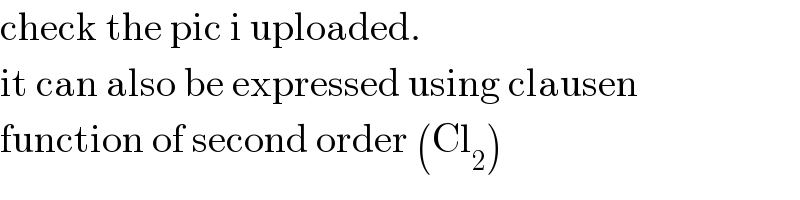
check the pic i uploaded. it can also be expressed using clausen function of second order (Cl_2 )

$$\mathrm{check}\:\mathrm{the}\:\mathrm{pic}\:\mathrm{i}\:\mathrm{uploaded}. \\ $$$$\mathrm{it}\:\mathrm{can}\:\mathrm{also}\:\mathrm{be}\:\mathrm{expressed}\:\mathrm{using}\:\mathrm{clausen} \\ $$$$\mathrm{function}\:\mathrm{of}\:\mathrm{second}\:\mathrm{order}\:\left(\mathrm{Cl}_{\mathrm{2}} \right) \\ $$

Answered by bemath last updated on 11/Oct/20

Terms of Service

Privacy Policy

Contact: info@tinkutara.com