
Question Number 161285 by cortano last updated on 15/Dec/21
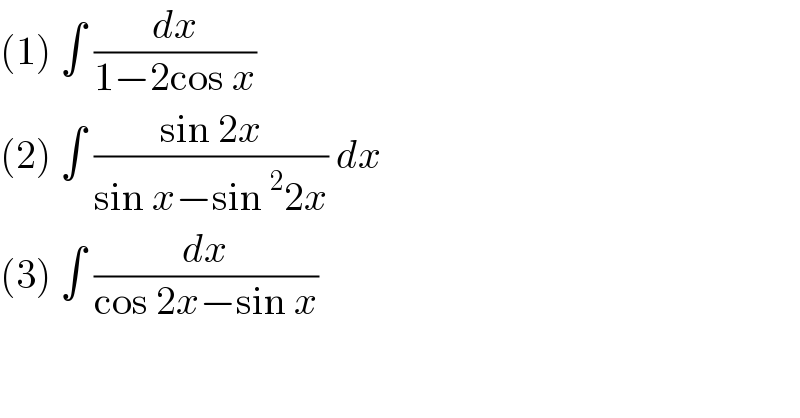
$$\left(\mathrm{1}\right)\:\int\:\frac{{dx}}{\mathrm{1}−\mathrm{2cos}\:{x}} \\ $$$$\left(\mathrm{2}\right)\:\int\:\frac{\mathrm{sin}\:\mathrm{2}{x}}{\mathrm{sin}\:{x}−\mathrm{sin}\:^{\mathrm{2}} \mathrm{2}{x}}\:{dx} \\ $$$$\left(\mathrm{3}\right)\:\int\:\frac{{dx}}{\mathrm{cos}\:\mathrm{2}{x}−\mathrm{sin}\:{x}} \\ $$
Answered by bobhans last updated on 15/Dec/21
![(1) ∫ (dx/(1−2cos x)) = ∫ (dx/(1−2(2cos^2 ((x/2))−1))) = ∫ (dx/(3−4cos^2 ((x/2)))) ; [tan (x/2)=t → { ((cos (x/2)=(1/( (√(1+t^2 )))))),((dx=(2/(1+t^2 )) dt)) :}] = ∫ (2/(1+t^2 )) ((1/(3−(4/(1+t^2 )))))dt = ∫ (2/(3t^2 −1)) dt = ∫ (2/((t(√3)−1)(t(√3)+1))) dt = ∫ ((1/(t(√3)−1)) −(1/(t(√3)+1)))dt = (1/( (√3))) ln ∣t(√3)−1∣−(1/( (√3))) ln ∣t(√3)+1∣ + c = (1/( (√3))) ln ∣(((√3) tan ((x/2))−1)/( (√3) tan ((x/2))+1))∣ + c](Q161287.png)
$$\left(\mathrm{1}\right)\:\int\:\frac{\mathrm{dx}}{\mathrm{1}−\mathrm{2cos}\:\mathrm{x}}\:=\:\int\:\frac{\mathrm{dx}}{\mathrm{1}−\mathrm{2}\left(\mathrm{2cos}\:^{\mathrm{2}} \left(\frac{\mathrm{x}}{\mathrm{2}}\right)−\mathrm{1}\right)} \\ $$$$\:\:\:\:=\:\int\:\frac{\mathrm{dx}}{\mathrm{3}−\mathrm{4cos}\:^{\mathrm{2}} \left(\frac{\mathrm{x}}{\mathrm{2}}\right)}\:;\:\left[\mathrm{tan}\:\frac{\mathrm{x}}{\mathrm{2}}=\mathrm{t}\:\rightarrow\begin{cases}{\mathrm{cos}\:\frac{\mathrm{x}}{\mathrm{2}}=\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}+\mathrm{t}^{\mathrm{2}} }}}\\{\mathrm{dx}=\frac{\mathrm{2}}{\mathrm{1}+\mathrm{t}^{\mathrm{2}} }\:\mathrm{dt}}\end{cases}\right] \\ $$$$\:\:\:=\:\int\:\frac{\mathrm{2}}{\mathrm{1}+\mathrm{t}^{\mathrm{2}} }\:\left(\frac{\mathrm{1}}{\mathrm{3}−\frac{\mathrm{4}}{\mathrm{1}+\mathrm{t}^{\mathrm{2}} }}\right)\mathrm{dt} \\ $$$$\:\:=\:\int\:\frac{\mathrm{2}}{\mathrm{3t}^{\mathrm{2}} −\mathrm{1}}\:\mathrm{dt}\:=\:\int\:\frac{\mathrm{2}}{\left(\mathrm{t}\sqrt{\mathrm{3}}−\mathrm{1}\right)\left(\mathrm{t}\sqrt{\mathrm{3}}+\mathrm{1}\right)}\:\mathrm{dt} \\ $$$$\:\:=\:\int\:\left(\frac{\mathrm{1}}{\mathrm{t}\sqrt{\mathrm{3}}−\mathrm{1}}\:−\frac{\mathrm{1}}{\mathrm{t}\sqrt{\mathrm{3}}+\mathrm{1}}\right)\mathrm{dt} \\ $$$$\:\:=\:\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}\:\mathrm{ln}\:\mid\mathrm{t}\sqrt{\mathrm{3}}−\mathrm{1}\mid−\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}\:\mathrm{ln}\:\mid\mathrm{t}\sqrt{\mathrm{3}}+\mathrm{1}\mid\:+\:\mathrm{c} \\ $$$$\:\:=\:\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}\:\mathrm{ln}\:\mid\frac{\sqrt{\mathrm{3}}\:\mathrm{tan}\:\left(\frac{\mathrm{x}}{\mathrm{2}}\right)−\mathrm{1}}{\:\sqrt{\mathrm{3}}\:\mathrm{tan}\:\left(\frac{\mathrm{x}}{\mathrm{2}}\right)+\mathrm{1}}\mid\:+\:\mathrm{c}\: \\ $$
Answered by bobhans last updated on 17/Dec/21
![(Q) ∫ (dx/(cos 2x−sin x)) =? (⇒ ) ∫ (dx/(1−2sin^2 x−sin x)) = ∫ (dx/(−2sin^2 x−sin x+1)) =−∫ (dx/(2sin^2 x+sin x−1)) = −∫ (dx/((2sin x−1)(sin x+1))) = −(1/3)∫ ((2/(2sin x−1))−(1/(sin x+1)))dx [ tan (x/2)=u → { ((sin x = ((2u)/(1+u^2 )))),((dx=(2/(1+u^2 )))) :} ] I_1 =−(2/3) ∫ (2/(1+u^2 )) .(1/(((4u)/(1+u^2 ))−1)) du I_1 = −(4/3)∫ (du/(4u−u^2 −1))= (4/3)∫ (du/((u−2)^2 −5)) [ let u−2 =(√5) sec t ] I_1 = (4/3) ∫ (((√5) sec t tan t dt)/(5 tan^2 t))=(4/(3(√5)))∫csc t dt I_1 = (4/(3(√5))) ln ∣ csc t−cot t ∣ + c_(1 ) I_2 = (1/3)∫ (dx/(sin x+1)) = (1/3)∫ (2/(1+u^2 )).(1/(((2u)/(1+u^2 ))+1)) du I_2 = (2/3)∫ (du/((u+1)^2 )) = (2/3)∫ (u+1)^(−2) du I_2 = −(2/(3(u+1))) + c_2 =−(2/(3(tan ((x/2))+1))) + c_2 ∴ I = I_1 +I_2](Q161290.png)
$$\:\left(\mathrm{Q}\right)\:\int\:\frac{\mathrm{dx}}{\mathrm{cos}\:\mathrm{2x}−\mathrm{sin}\:\mathrm{x}}\:=? \\ $$$$\left(\Rightarrow\:\:\right)\:\int\:\frac{\mathrm{dx}}{\mathrm{1}−\mathrm{2sin}\:^{\mathrm{2}} \mathrm{x}−\mathrm{sin}\:\mathrm{x}}\:=\:\int\:\frac{\mathrm{dx}}{−\mathrm{2sin}^{\mathrm{2}} \mathrm{x}−\mathrm{sin}\:\mathrm{x}+\mathrm{1}} \\ $$$$\:=−\int\:\frac{\mathrm{dx}}{\mathrm{2sin}\:^{\mathrm{2}} \mathrm{x}+\mathrm{sin}\:\mathrm{x}−\mathrm{1}}\:=\:−\int\:\frac{\mathrm{dx}}{\left(\mathrm{2sin}\:\mathrm{x}−\mathrm{1}\right)\left(\mathrm{sin}\:\mathrm{x}+\mathrm{1}\right)} \\ $$$$\:=\:−\frac{\mathrm{1}}{\mathrm{3}}\int\:\left(\frac{\mathrm{2}}{\mathrm{2sin}\:\mathrm{x}−\mathrm{1}}−\frac{\mathrm{1}}{\mathrm{sin}\:\mathrm{x}+\mathrm{1}}\right)\mathrm{dx} \\ $$$$\:\:\left[\:\mathrm{tan}\:\frac{\mathrm{x}}{\mathrm{2}}=\mathrm{u}\:\rightarrow\begin{cases}{\mathrm{sin}\:\mathrm{x}\:=\:\frac{\mathrm{2u}}{\mathrm{1}+\mathrm{u}^{\mathrm{2}} }}\\{\mathrm{dx}=\frac{\mathrm{2}}{\mathrm{1}+\mathrm{u}^{\mathrm{2}} }}\end{cases}\:\right] \\ $$$$\:\mathrm{I}_{\mathrm{1}} \:=−\frac{\mathrm{2}}{\mathrm{3}}\:\int\:\frac{\mathrm{2}}{\mathrm{1}+\mathrm{u}^{\mathrm{2}} }\:.\frac{\mathrm{1}}{\frac{\mathrm{4u}}{\mathrm{1}+\mathrm{u}^{\mathrm{2}} }−\mathrm{1}}\:\mathrm{du}\: \\ $$$$\:\mathrm{I}_{\mathrm{1}} \:=\:−\frac{\mathrm{4}}{\mathrm{3}}\int\:\:\frac{\mathrm{du}}{\mathrm{4u}−\mathrm{u}^{\mathrm{2}} −\mathrm{1}}=\:\frac{\mathrm{4}}{\mathrm{3}}\int\:\frac{\mathrm{du}}{\left(\mathrm{u}−\mathrm{2}\right)^{\mathrm{2}} −\mathrm{5}} \\ $$$$\:\left[\:\mathrm{let}\:\mathrm{u}−\mathrm{2}\:=\sqrt{\mathrm{5}}\:\mathrm{sec}\:\mathrm{t}\:\right]\: \\ $$$$\:\mathrm{I}_{\mathrm{1}} \:=\:\frac{\mathrm{4}}{\mathrm{3}}\:\int\:\frac{\sqrt{\mathrm{5}}\:\mathrm{sec}\:\mathrm{t}\:\mathrm{tan}\:\mathrm{t}\:\mathrm{dt}}{\mathrm{5}\:\mathrm{tan}\:^{\mathrm{2}} \mathrm{t}}=\frac{\mathrm{4}}{\mathrm{3}\sqrt{\mathrm{5}}}\int\mathrm{csc}\:\mathrm{t}\:\mathrm{dt} \\ $$$$\:\mathrm{I}_{\mathrm{1}} \:=\:\frac{\mathrm{4}}{\mathrm{3}\sqrt{\mathrm{5}}}\:\mathrm{ln}\:\mid\:\mathrm{csc}\:\mathrm{t}−\mathrm{cot}\:\mathrm{t}\:\mid\:+\:\mathrm{c}_{\mathrm{1}\:} \\ $$$$\:\mathrm{I}_{\mathrm{2}} \:=\:\frac{\mathrm{1}}{\mathrm{3}}\int\:\frac{\mathrm{dx}}{\mathrm{sin}\:\mathrm{x}+\mathrm{1}}\:=\:\frac{\mathrm{1}}{\mathrm{3}}\int\:\frac{\mathrm{2}}{\mathrm{1}+\mathrm{u}^{\mathrm{2}} }.\frac{\mathrm{1}}{\frac{\mathrm{2u}}{\mathrm{1}+\mathrm{u}^{\mathrm{2}} }+\mathrm{1}}\:\mathrm{du} \\ $$$$\:\mathrm{I}_{\mathrm{2}} \:=\:\frac{\mathrm{2}}{\mathrm{3}}\int\:\frac{\mathrm{du}}{\left(\mathrm{u}+\mathrm{1}\right)^{\mathrm{2}} }\:=\:\frac{\mathrm{2}}{\mathrm{3}}\int\:\left(\mathrm{u}+\mathrm{1}\right)^{−\mathrm{2}} \:\mathrm{du} \\ $$$$\:\mathrm{I}_{\mathrm{2}} =\:−\frac{\mathrm{2}}{\mathrm{3}\left(\mathrm{u}+\mathrm{1}\right)}\:+\:\mathrm{c}_{\mathrm{2}} =−\frac{\mathrm{2}}{\mathrm{3}\left(\mathrm{tan}\:\left(\frac{\mathrm{x}}{\mathrm{2}}\right)+\mathrm{1}\right)}\:+\:\mathrm{c}_{\mathrm{2}} \\ $$$$\:\:\:\therefore\:\mathrm{I}\:=\:\mathrm{I}_{\mathrm{1}} +\mathrm{I}_{\mathrm{2}} \\ $$$$\: \\ $$
Answered by Ar Brandon last updated on 15/Dec/21

$${I}=\int\frac{{dx}}{\mathrm{1}−\mathrm{2cos}{x}}\:,\:{t}=\mathrm{tan}\frac{{x}}{\mathrm{2}} \\ $$$$\:\:\:=\int\frac{\mathrm{2}}{\mathrm{1}−\mathrm{2}\frac{\mathrm{1}−{t}^{\mathrm{2}} }{\mathrm{1}+{t}^{\mathrm{2}} }}\centerdot\frac{{dt}}{\mathrm{1}+{t}^{\mathrm{2}} }=\mathrm{2}\int\frac{{dt}}{\mathrm{3}{t}^{\mathrm{2}} −\mathrm{1}} \\ $$$$\:\:\:=−\frac{\mathrm{2}}{\:\sqrt{\mathrm{3}}}\mathrm{argth}\left(\sqrt{\mathrm{3}}{t}\right)+{C}=−\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}\mathrm{ln}\mid\frac{\mathrm{1}+\sqrt{\mathrm{3}}{t}}{\mathrm{1}−\sqrt{\mathrm{3}}{t}}\mid+{C} \\ $$$$\:\:\:=\frac{\sqrt{\mathrm{3}}}{\mathrm{3}}\mathrm{ln}\mid\frac{\mathrm{1}−\sqrt{\mathrm{3}}\mathrm{tan}\frac{{x}}{\mathrm{2}}}{\mathrm{1}+\sqrt{\mathrm{3}}\mathrm{tan}\frac{{x}}{\mathrm{2}}}\mid+{C}\: \\ $$
Answered by Ar Brandon last updated on 15/Dec/21
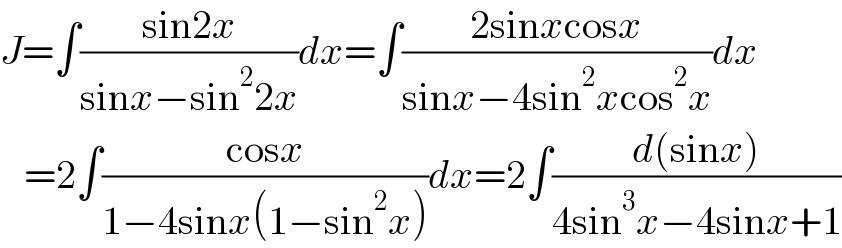
$${J}=\int\frac{\mathrm{sin2}{x}}{\mathrm{sin}{x}−\mathrm{sin}^{\mathrm{2}} \mathrm{2}{x}}{dx}=\int\frac{\mathrm{2sin}{x}\mathrm{cos}{x}}{\mathrm{sin}{x}−\mathrm{4sin}^{\mathrm{2}} {x}\mathrm{cos}^{\mathrm{2}} {x}}{dx} \\ $$$$\:\:\:=\mathrm{2}\int\frac{\mathrm{cos}{x}}{\mathrm{1}−\mathrm{4sin}{x}\left(\mathrm{1}−\mathrm{sin}^{\mathrm{2}} {x}\right)}{dx}=\mathrm{2}\int\frac{{d}\left(\mathrm{sin}{x}\right)}{\mathrm{4sin}^{\mathrm{3}} {x}−\mathrm{4sin}{x}+\mathrm{1}} \\ $$
Answered by Tyller last updated on 19/Dec/21
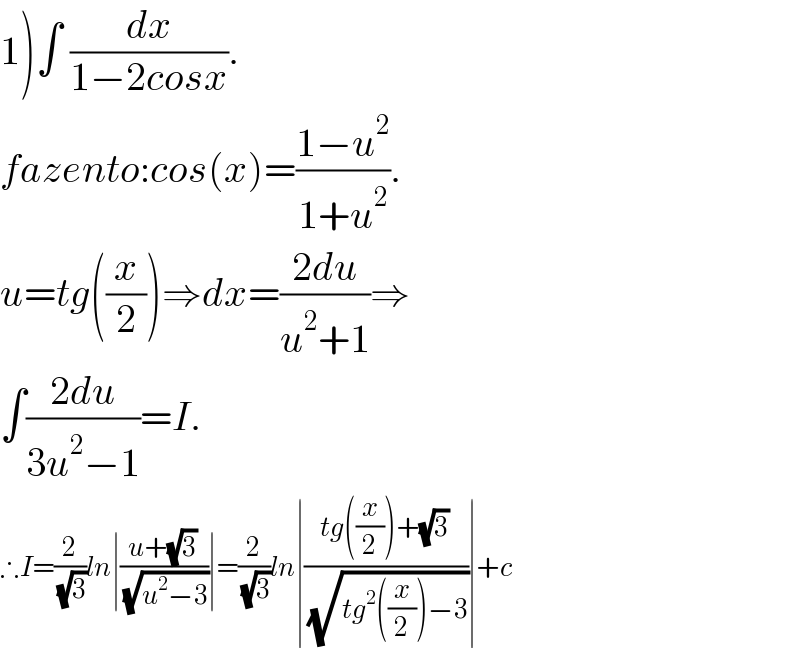
$$\left.\mathrm{1}\right)\int\:\frac{{dx}}{\mathrm{1}−\mathrm{2}{cosx}}. \\ $$$${fazento}:{cos}\left({x}\right)=\frac{\mathrm{1}−{u}^{\mathrm{2}} }{\mathrm{1}+{u}^{\mathrm{2}} }. \\ $$$${u}={tg}\left(\frac{{x}}{\mathrm{2}}\right)\Rightarrow{dx}=\frac{\mathrm{2}{du}}{{u}^{\mathrm{2}} +\mathrm{1}}\Rightarrow \\ $$$$\int\frac{\mathrm{2}{du}}{\mathrm{3}{u}^{\mathrm{2}} −\mathrm{1}}={I}. \\ $$$$\therefore{I}=\frac{\mathrm{2}}{\:\sqrt{\mathrm{3}}}{ln}\mid\frac{{u}+\sqrt{\mathrm{3}}}{\:\sqrt{{u}^{\mathrm{2}} −\mathrm{3}}}\mid=\frac{\mathrm{2}}{\:\sqrt{\mathrm{3}}}{ln}\mid\frac{{tg}\left(\frac{{x}}{\mathrm{2}}\right)+\sqrt{\mathrm{3}}}{\:\sqrt{{tg}^{\mathrm{2}} \left(\frac{{x}}{\mathrm{2}}\right)−\mathrm{3}}}\mid+{c} \\ $$
