
Question Number 72492 by petrochengula last updated on 29/Oct/19
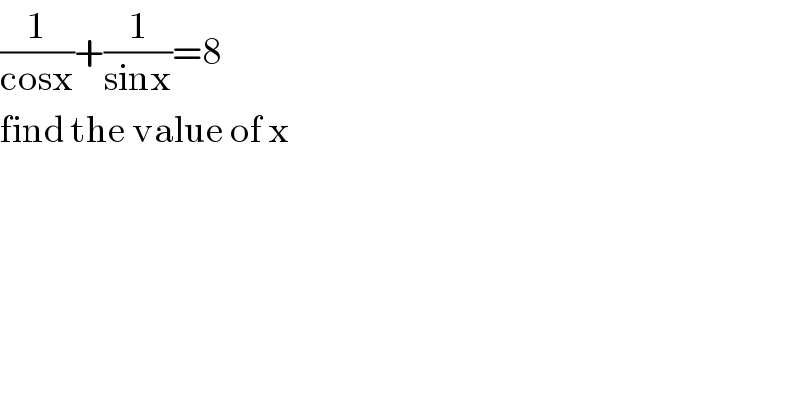
$$\frac{\mathrm{1}}{\mathrm{cosx}}+\frac{\mathrm{1}}{\mathrm{sinx}}=\mathrm{8} \\ $$$$\mathrm{find}\:\mathrm{the}\:\mathrm{value}\:\mathrm{of}\:\mathrm{x} \\ $$
Commented by petrochengula last updated on 29/Oct/19

$$\mathrm{help}\:\mathrm{please} \\ $$
Answered by behi83417@gmail.com last updated on 29/Oct/19
![sinx+cosx=8sinx.cosx (sinx+cosx)^2 =(8sinx.cosx)^2 1+2sinx.cosx=64sin^2 xcos^2 x [let:sinxcosx=y] 65y^2 =y^2 +2y+1=(y+1)^2 ⇒(√(65))y=±(2y+1)⇒ { ((y=(1/((√(65))−2)))),((y=((−1)/((√(65))+2)))) :} 1)sinx.cosx=(1/((√(65))−2))⇒sin2x=(2/((√(65))−2)) ⇒x=kπ±(1/2)sin^(−1) [(2/((√(65))−2))] (k∈z) 2)sinx.cosx=((−1)/((√(65))+2))⇒sin2x=((−2)/((√(65))+2)) ⇒x=lπ±(1/2)sin^(−1) [((−2)/((√(65))+2))] (l∈z)](Q72503.png)
$$\mathrm{sinx}+\mathrm{cosx}=\mathrm{8sinx}.\mathrm{cosx} \\ $$$$\left(\mathrm{sinx}+\mathrm{cosx}\right)^{\mathrm{2}} =\left(\mathrm{8sinx}.\mathrm{cosx}\right)^{\mathrm{2}} \\ $$$$\mathrm{1}+\mathrm{2sinx}.\mathrm{cosx}=\mathrm{64sin}^{\mathrm{2}} \mathrm{xcos}^{\mathrm{2}} \mathrm{x}\:\:\:\left[\mathrm{let}:\mathrm{sinxcosx}=\mathrm{y}\right] \\ $$$$\mathrm{65y}^{\mathrm{2}} =\mathrm{y}^{\mathrm{2}} +\mathrm{2y}+\mathrm{1}=\left(\mathrm{y}+\mathrm{1}\right)^{\mathrm{2}} \\ $$$$\Rightarrow\sqrt{\mathrm{65}}\mathrm{y}=\pm\left(\mathrm{2y}+\mathrm{1}\right)\Rightarrow\begin{cases}{\mathrm{y}=\frac{\mathrm{1}}{\sqrt{\mathrm{65}}−\mathrm{2}}}\\{\mathrm{y}=\frac{−\mathrm{1}}{\sqrt{\mathrm{65}}+\mathrm{2}}}\end{cases} \\ $$$$\left.\mathrm{1}\right)\mathrm{sinx}.\mathrm{cosx}=\frac{\mathrm{1}}{\sqrt{\mathrm{65}}−\mathrm{2}}\Rightarrow\mathrm{sin2x}=\frac{\mathrm{2}}{\sqrt{\mathrm{65}}−\mathrm{2}} \\ $$$$\Rightarrow\mathrm{x}=\mathrm{k}\pi\pm\frac{\mathrm{1}}{\mathrm{2}}\mathrm{sin}^{−\mathrm{1}} \left[\frac{\mathrm{2}}{\sqrt{\mathrm{65}}−\mathrm{2}}\right]\:\:\left(\mathrm{k}\in\mathrm{z}\right) \\ $$$$\left.\mathrm{2}\right)\mathrm{sinx}.\mathrm{cosx}=\frac{−\mathrm{1}}{\sqrt{\mathrm{65}}+\mathrm{2}}\Rightarrow\mathrm{sin2x}=\frac{−\mathrm{2}}{\sqrt{\mathrm{65}}+\mathrm{2}} \\ $$$$\Rightarrow\mathrm{x}=\mathrm{l}\pi\pm\frac{\mathrm{1}}{\mathrm{2}}\mathrm{sin}^{−\mathrm{1}} \left[\frac{−\mathrm{2}}{\sqrt{\mathrm{65}}+\mathrm{2}}\right]\:\:\:\:\left(\mathrm{l}\in\mathrm{z}\right) \\ $$
Commented by petrochengula last updated on 29/Oct/19

$$\mathrm{thanks} \\ $$
Answered by Tanmay chaudhury last updated on 29/Oct/19
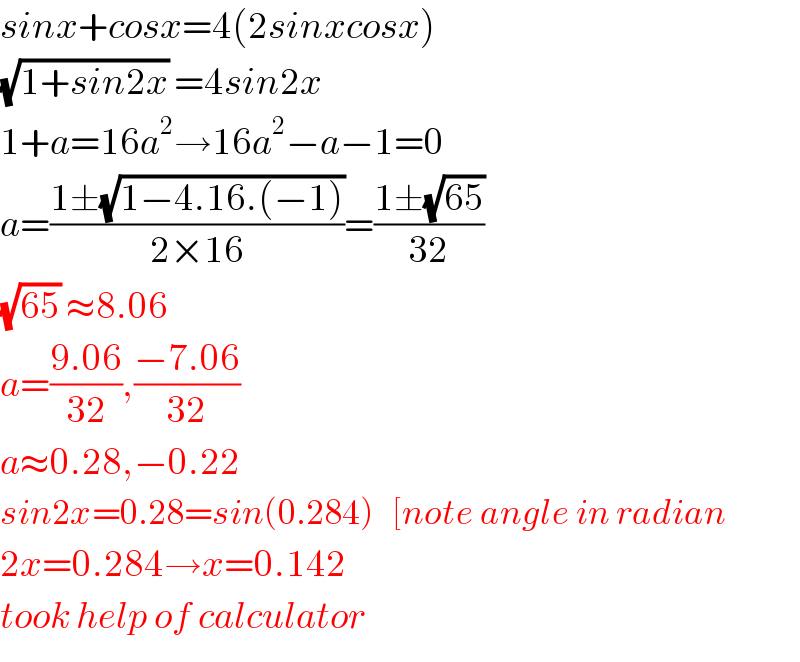
$${sinx}+{cosx}=\mathrm{4}\left(\mathrm{2}{sinxcosx}\right) \\ $$$$\sqrt{\mathrm{1}+{sin}\mathrm{2}{x}}\:=\mathrm{4}{sin}\mathrm{2}{x} \\ $$$$\mathrm{1}+{a}=\mathrm{16}{a}^{\mathrm{2}} \rightarrow\mathrm{16}{a}^{\mathrm{2}} −{a}−\mathrm{1}=\mathrm{0} \\ $$$${a}=\frac{\mathrm{1}\pm\sqrt{\mathrm{1}−\mathrm{4}.\mathrm{16}.\left(−\mathrm{1}\right)}}{\mathrm{2}×\mathrm{16}}=\frac{\mathrm{1}\pm\sqrt{\mathrm{65}}}{\mathrm{32}} \\ $$$$\sqrt{\mathrm{65}}\:\approx\mathrm{8}.\mathrm{06}\: \\ $$$${a}=\frac{\mathrm{9}.\mathrm{06}}{\mathrm{32}},\frac{−\mathrm{7}.\mathrm{06}}{\mathrm{32}} \\ $$$${a}\approx\mathrm{0}.\mathrm{28},−\mathrm{0}.\mathrm{22} \\ $$$${sin}\mathrm{2}{x}=\mathrm{0}.\mathrm{28}={sin}\left(\mathrm{0}.\mathrm{284}\right)\:\:\:\left[{note}\:{angle}\:{in}\:{radian}\right. \\ $$$$\mathrm{2}{x}=\mathrm{0}.\mathrm{284}\rightarrow{x}=\mathrm{0}.\mathrm{142} \\ $$$${took}\:{help}\:{of}\:{calculator} \\ $$
Commented by petrochengula last updated on 29/Oct/19

$$\mathrm{thanks} \\ $$
Commented by Tanmay chaudhury last updated on 29/Oct/19

$${most}\:{welcome}... \\ $$
Answered by MJS last updated on 29/Oct/19

$${x}=\mathrm{2arctan}\:{t}\:\Rightarrow\:\mathrm{cos}\:{x}\:=\frac{\mathrm{1}−{t}^{\mathrm{2}} }{\mathrm{1}+{t}^{\mathrm{2}} };\:\mathrm{sin}\:{x}\:=\frac{\mathrm{2}{t}}{\mathrm{1}+{t}^{\mathrm{2}} } \\ $$$$\frac{\mathrm{1}+{t}^{\mathrm{2}} }{\mathrm{1}−{t}^{\mathrm{2}} }+\frac{\mathrm{1}+{t}^{\mathrm{2}} }{\mathrm{2}{t}}=\mathrm{8} \\ $$$$\mathrm{transforming}\:\Rightarrow \\ $$$${t}^{\mathrm{4}} −\mathrm{18}{t}^{\mathrm{3}} +\mathrm{14}{t}−\mathrm{1}=\mathrm{0} \\ $$$$\mathrm{we}\:\mathrm{can}\:\mathrm{exactly}\:\mathrm{solve}\:\mathrm{this} \\ $$$$\left({t}^{\mathrm{2}} −\left(\mathrm{9}+\sqrt{\mathrm{65}}\right){t}−\left(\mathrm{8}+\sqrt{\mathrm{65}}\right)\right)\left({t}^{\mathrm{2}} −\left(\mathrm{9}−\sqrt{\mathrm{65}}\right){t}−\left(\mathrm{8}{r}−\sqrt{\mathrm{65}}\right)\right)=\mathrm{0} \\ $$$${t}_{\mathrm{1},\:\mathrm{2}} =\frac{\mathrm{9}+\sqrt{\mathrm{65}}}{\mathrm{2}}\pm\frac{\sqrt{\mathrm{178}+\mathrm{22}\sqrt{\mathrm{65}}}}{\mathrm{2}} \\ $$$${t}_{\mathrm{3},\:\mathrm{4}} =\frac{\mathrm{9}−\sqrt{\mathrm{65}}}{\mathrm{2}}\pm\frac{\sqrt{\mathrm{178}−\mathrm{22}\sqrt{\mathrm{65}}}}{\mathrm{2}} \\ $$$${x}_{{i}} =\mathrm{2}\pi{n}+\mathrm{2arctan}\:{t}\:\mathrm{with}\:{n}\in\mathbb{Z} \\ $$$$\Rightarrow \\ $$$${x}_{\mathrm{1}} \approx−\mathrm{1}.\mathrm{45953}+\mathrm{2}\pi{n} \\ $$$${x}_{\mathrm{2}} \approx\mathrm{3}.\mathrm{03033}+\mathrm{2}\pi{n} \\ $$$${x}_{\mathrm{3}} \approx.\mathrm{143562}+\mathrm{2}\pi{n} \\ $$$${x}_{\mathrm{4}} \approx\mathrm{1}.\mathrm{42723}+\mathrm{2}\pi{n} \\ $$
