
Question Number 53271 by Abdo msup. last updated on 19/Jan/19
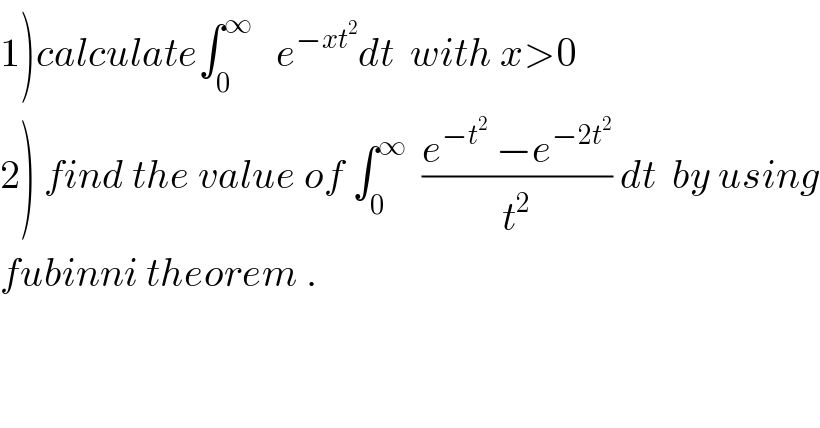
$$\left.\mathrm{1}\right){calculate}\int_{\mathrm{0}} ^{\infty} \:\:\:{e}^{−{xt}^{\mathrm{2}} } {dt}\:\:{with}\:{x}>\mathrm{0} \\ $$$$\left.\mathrm{2}\right)\:{find}\:{the}\:{value}\:{of}\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{{e}^{−{t}^{\mathrm{2}} } \:−{e}^{−\mathrm{2}{t}^{\mathrm{2}} } }{{t}^{\mathrm{2}} }\:{dt}\:\:{by}\:{using} \\ $$$${fubinni}\:{theorem}\:. \\ $$
Commented by maxmathsup by imad last updated on 22/Jan/19
![1) we have ∫_0 ^∞ e^(−xt^2 ) dt =_(t(√x)=u) ∫_0 ^∞ e^(−u^2 ) (du/(√x)) =(1/(√x)) ∫_0 ^∞ e^(−u^2 ) du =(1/(√x)) ((√π)/2) =((√π)/(2(√x))) 2) we have ∫_1 ^2 ((√π)/(2(√x))) dx =(√π)[(√x)]_1 ^2 =(√π)((√2)−1) but ∫_1 ^2 ((√π)/(2(√x))) dx =∫_1 ^2 (∫_0 ^∞ e^(−xt^2 ) dt)dx =∫_0 ^∞ ( ∫_1 ^2 e^(−xt^2 ) dx)dt (by fubini) =∫_0 ^∞ ( [−(1/t^2 ) e^(−xt^2 ) ]_(x=1) ^(x=2) )dt =∫_0 ^∞ ((e^(−t^2 ) −e^(−2t^2 ) )/t^2 ) dt ⇒ ∫_0 ^∞ ((e^(−t^2 ) −e^(−2t^2 ) )/t^2 ) dt =(√π)((√2)−1)](Q53457.png)
$$\left.\mathrm{1}\right)\:{we}\:{have}\:\int_{\mathrm{0}} ^{\infty} \:{e}^{−{xt}^{\mathrm{2}} } {dt}\:=_{{t}\sqrt{{x}}={u}} \:\:\int_{\mathrm{0}} ^{\infty} \:\:{e}^{−{u}^{\mathrm{2}} } \frac{{du}}{\sqrt{{x}}}\:=\frac{\mathrm{1}}{\sqrt{{x}}}\:\int_{\mathrm{0}} ^{\infty} \:{e}^{−{u}^{\mathrm{2}} } {du} \\ $$$$=\frac{\mathrm{1}}{\sqrt{{x}}}\:\frac{\sqrt{\pi}}{\mathrm{2}}\:=\frac{\sqrt{\pi}}{\mathrm{2}\sqrt{{x}}} \\ $$$$\left.\mathrm{2}\right)\:{we}\:{have}\:\int_{\mathrm{1}} ^{\mathrm{2}} \:\:\frac{\sqrt{\pi}}{\mathrm{2}\sqrt{{x}}}\:{dx}\:=\sqrt{\pi}\left[\sqrt{{x}}\right]_{\mathrm{1}} ^{\mathrm{2}} =\sqrt{\pi}\left(\sqrt{\mathrm{2}}−\mathrm{1}\right)\:\:{but} \\ $$$$\int_{\mathrm{1}} ^{\mathrm{2}} \:\frac{\sqrt{\pi}}{\mathrm{2}\sqrt{{x}}}\:{dx}\:=\int_{\mathrm{1}} ^{\mathrm{2}} \left(\int_{\mathrm{0}} ^{\infty} \:{e}^{−{xt}^{\mathrm{2}} } {dt}\right){dx}\:=\int_{\mathrm{0}} ^{\infty} \:\left(\:\int_{\mathrm{1}} ^{\mathrm{2}} \:{e}^{−{xt}^{\mathrm{2}} } {dx}\right){dt}\:\left({by}\:{fubini}\right) \\ $$$$=\int_{\mathrm{0}} ^{\infty} \:\left(\:\:\left[−\frac{\mathrm{1}}{{t}^{\mathrm{2}} }\:{e}^{−{xt}^{\mathrm{2}} } \right]_{{x}=\mathrm{1}} ^{{x}=\mathrm{2}} \right){dt}\:=\int_{\mathrm{0}} ^{\infty} \:\frac{{e}^{−{t}^{\mathrm{2}} } \:−{e}^{−\mathrm{2}{t}^{\mathrm{2}} } }{{t}^{\mathrm{2}} }\:{dt}\:\Rightarrow \\ $$$$\int_{\mathrm{0}} ^{\infty} \:\:\frac{{e}^{−{t}^{\mathrm{2}} } −{e}^{−\mathrm{2}{t}^{\mathrm{2}} } }{{t}^{\mathrm{2}} }\:{dt}\:=\sqrt{\pi}\left(\sqrt{\mathrm{2}}−\mathrm{1}\right) \\ $$$$ \\ $$
Commented by maxmathsup by imad last updated on 22/Jan/19

$${anther}\:{method}\:{leta}>\mathrm{0}\:{and}\:{b}>\mathrm{0}{and}\:{f}\left({a},{b}\right)\:=\int_{\mathrm{0}} ^{\infty} \:\:\frac{{e}^{−{ax}^{\mathrm{2}} } −{e}^{−{bx}^{\mathrm{2}} } }{{x}^{\mathrm{2}} }\:{dx}\:\Rightarrow\frac{\partial{f}}{\partial{a}}\left({a},{b}\right)=−\int_{\mathrm{0}} ^{\infty} \:{e}^{−{ax}^{\mathrm{2}} } {dx} \\ $$$$=−\int_{\mathrm{0}} ^{\infty} \:\:{e}^{−\left(\sqrt{{a}}{x}\right)^{\mathrm{2}} } {dx}\:=_{\sqrt{{a}}{x}\:={t}} \:\:\:−\int_{\mathrm{0}} ^{\infty} \:\:{e}^{−{t}^{\mathrm{2}} } \frac{{dt}}{\sqrt{{a}}} \\ $$$$=−\frac{\mathrm{1}}{\sqrt{{a}}}\:\frac{\sqrt{\pi}}{\mathrm{2}}\:=−\frac{\sqrt{\pi}}{\mathrm{2}\sqrt{{a}}}\:\Rightarrow{f}\left({a},{b}\right)=\:−\sqrt{\pi}\int\:\:\frac{{da}}{\mathrm{2}\sqrt{{a}}}\:=−\sqrt{\pi}\sqrt{{a}}\:+{c} \\ $$$${c}={f}\left(\mathrm{0},{b}\right)\:=\int_{\mathrm{0}} ^{\infty} \:\:\frac{\mathrm{1}−{e}^{−{bx}^{\mathrm{2}} } }{{x}^{\mathrm{2}} }\:{dx}\:=\varphi\left({b}\right)\:\Rightarrow\varphi^{'} \left({b}\right)=\int_{\mathrm{0}} ^{\infty} \:{e}^{−{bx}^{\mathrm{2}} } {dx}\:=\frac{\sqrt{\pi}}{\mathrm{2}\sqrt{{b}}}\:\Rightarrow \\ $$$$\varphi\left({b}\right)\:=\sqrt{\pi}\sqrt{{b}\:}\:+{c}_{\mathrm{0}} \:\:{but}\:\varphi\left(\mathrm{0}\right)\:={c}_{\mathrm{0}} \:\Rightarrow\varphi\left({b}\right)=\sqrt{\pi}\sqrt{{b}}\:\Rightarrow{f}\left({a},{b}\right)=−\sqrt{\pi}\sqrt{{a}}+\sqrt{\pi}\sqrt{{b}} \\ $$$$=\sqrt{\pi}\left(\sqrt{{b}\:}−\sqrt{{a}}\right)\:\:{finally}\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{{e}^{−{t}^{\mathrm{2}} } −{e}^{−\mathrm{2}{t}^{\mathrm{2}} } }{{t}^{\mathrm{2}} }{dt}\:={f}\left(\mathrm{1},\mathrm{2}\right)\:=\sqrt{\pi}\left(\sqrt{\mathrm{2}}−\mathrm{1}\right). \\ $$
