
Question Number 116253 by I want to learn more last updated on 02/Oct/20
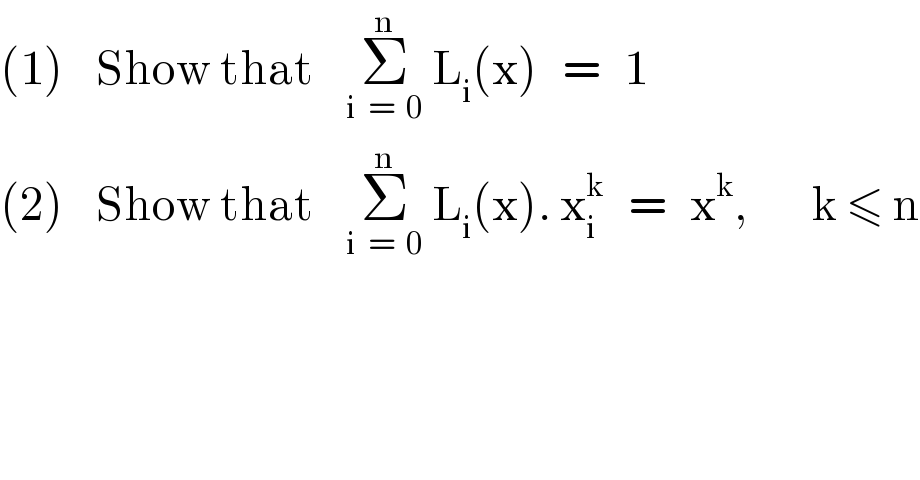
$$\left(\mathrm{1}\right)\:\:\:\:\mathrm{Show}\:\mathrm{that}\:\:\:\:\underset{\mathrm{i}\:\:=\:\:\mathrm{0}} {\overset{\mathrm{n}} {\sum}}\:\mathrm{L}_{\mathrm{i}} \left(\mathrm{x}\right)\:\:\:=\:\:\:\mathrm{1} \\ $$$$\left(\mathrm{2}\right)\:\:\:\:\mathrm{Show}\:\mathrm{that}\:\:\:\:\underset{\mathrm{i}\:\:=\:\:\mathrm{0}} {\overset{\mathrm{n}} {\sum}}\:\mathrm{L}_{\mathrm{i}} \left(\mathrm{x}\right).\:\mathrm{x}_{\mathrm{i}} ^{\mathrm{k}} \:\:\:=\:\:\:\mathrm{x}^{\mathrm{k}} ,\:\:\:\:\:\:\:\:\mathrm{k}\:\leqslant\:\mathrm{n} \\ $$
