
Question Number 139800 by bramlexs22 last updated on 01/May/21

$$\frac{\mathrm{1}}{\mathrm{3}}+\frac{\mathrm{6}}{\mathrm{21}}+\frac{\mathrm{11}}{\mathrm{147}}+\frac{\mathrm{16}}{\mathrm{1029}}+\frac{\mathrm{21}}{\mathrm{7203}}+\frac{\mathrm{26}}{\mathrm{50421}}+\ldots=? \\ $$
Answered by EDWIN88 last updated on 01/May/21
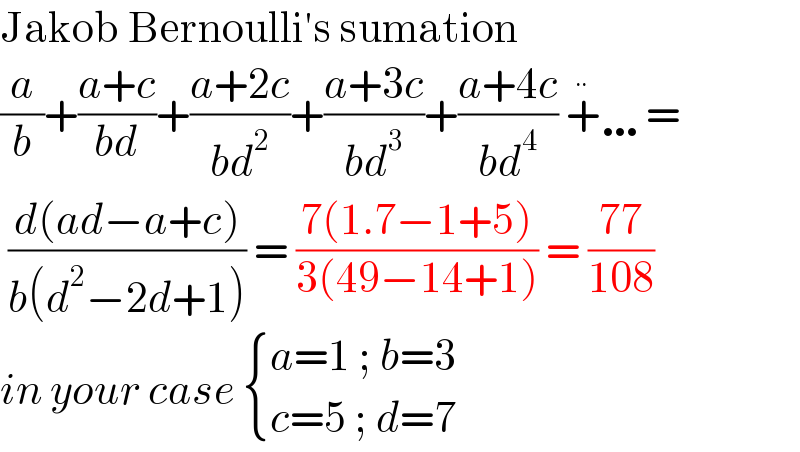
$$\mathrm{Jakob}\:\mathrm{Bernoulli}'\mathrm{s}\:\mathrm{sumation} \\ $$$$\frac{{a}}{{b}}+\frac{{a}+{c}}{{bd}}+\frac{{a}+\mathrm{2}{c}}{{bd}^{\mathrm{2}} }+\frac{{a}+\mathrm{3}{c}}{{bd}^{\mathrm{3}} }+\frac{{a}+\mathrm{4}{c}}{{bd}^{\mathrm{4}} }\:\ddot {+}\ldots= \\ $$$$\:\frac{{d}\left({ad}−{a}+{c}\right)}{{b}\left({d}^{\mathrm{2}} −\mathrm{2}{d}+\mathrm{1}\right)}\:=\:\frac{\mathrm{7}\left(\mathrm{1}.\mathrm{7}−\mathrm{1}+\mathrm{5}\right)}{\mathrm{3}\left(\mathrm{49}−\mathrm{14}+\mathrm{1}\right)}\:=\:\frac{\mathrm{77}}{\mathrm{108}} \\ $$$${in}\:{your}\:{case}\:\begin{cases}{{a}=\mathrm{1}\:;\:{b}=\mathrm{3}}\\{{c}=\mathrm{5}\:;\:{d}=\mathrm{7}}\end{cases} \\ $$
Commented by mohammad17 last updated on 01/May/21

$${sir}\:{whats}\:{low}\:{lf}\:{Jakob}\:{Bernoullis}\: \\ $$$${i}\:{want}\:{this}\:{low}\:{can}\:{you}\:{help}\:{me} \\ $$$$ \\ $$
Answered by qaz last updated on 01/May/21

$${S}=\frac{\mathrm{1}}{\mathrm{3}}+\frac{\mathrm{6}}{\mathrm{3}\centerdot\mathrm{7}}+\frac{\mathrm{11}}{\mathrm{3}\centerdot\mathrm{7}^{\mathrm{2}} }+\frac{\mathrm{16}}{\mathrm{3}\centerdot\mathrm{7}^{\mathrm{3}} }+... \\ $$$$=\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{\mathrm{5}{n}+\mathrm{1}}{\mathrm{3}\centerdot\mathrm{7}^{{n}} }=\frac{\mathrm{5}{xD}+\mathrm{1}}{\mathrm{3}}\mid_{{x}=\frac{\mathrm{1}}{\mathrm{7}}} \underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}{x}^{{n}} \\ $$$$=\frac{\mathrm{5}{xD}+\mathrm{1}}{\mathrm{3}}\mid_{{x}=\frac{\mathrm{1}}{\mathrm{7}}} \frac{\mathrm{1}}{\mathrm{1}−{x}}=\frac{\mathrm{1}}{\mathrm{3}}\mid_{{x}=\frac{\mathrm{1}}{\mathrm{7}}} \left(\frac{\mathrm{5}{x}}{\left(\mathrm{1}−{x}\right)^{\mathrm{2}} }+\frac{\mathrm{1}}{\mathrm{1}−{x}}\right) \\ $$$$=\frac{\mathrm{77}}{\mathrm{108}} \\ $$
Commented by bramlexs22 last updated on 01/May/21

$$\mathrm{answer}\:\frac{\mathrm{77}}{\mathrm{108}} \\ $$
Commented by qaz last updated on 01/May/21

$${i}\:{got}\:{a}\:{mistake} \\ $$
