
Question Number 121739 by Dwaipayan Shikari last updated on 11/Nov/20
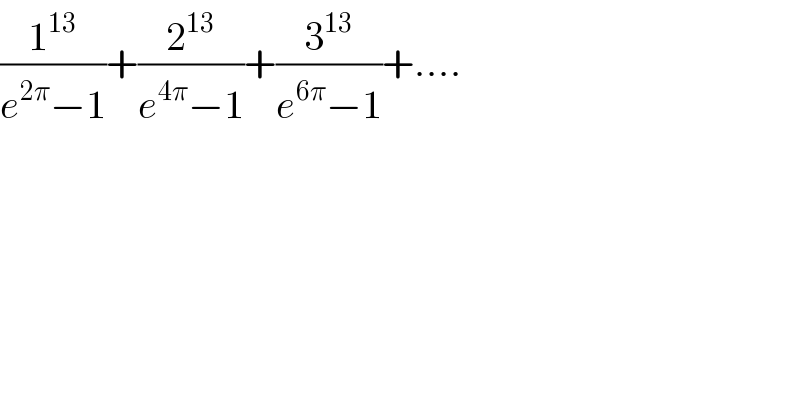
$$\frac{\mathrm{1}^{\mathrm{13}} }{{e}^{\mathrm{2}\pi} −\mathrm{1}}+\frac{\mathrm{2}^{\mathrm{13}} }{{e}^{\mathrm{4}\pi} −\mathrm{1}}+\frac{\mathrm{3}^{\mathrm{13}} }{{e}^{\mathrm{6}\pi} −\mathrm{1}}+.... \\ $$
Answered by mindispower last updated on 13/Nov/20
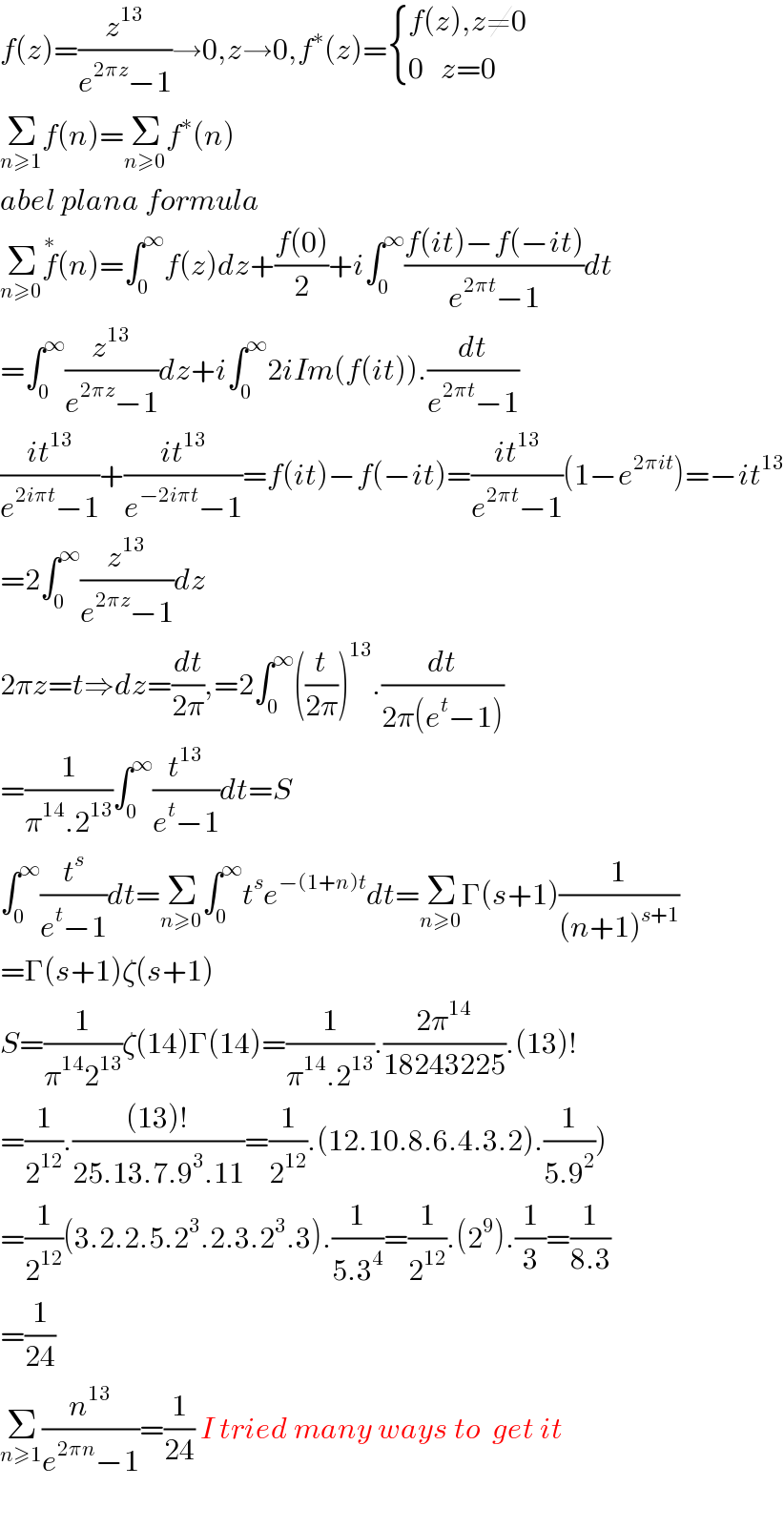
$${f}\left({z}\right)=\frac{{z}^{\mathrm{13}} }{{e}^{\mathrm{2}\pi{z}} −\mathrm{1}}\rightarrow\mathrm{0},{z}\rightarrow\mathrm{0},{f}^{\ast} \left({z}\right)=\begin{cases}{{f}\left({z}\right),{z}\neq\mathrm{0}}\\{\mathrm{0}\:\:\:{z}=\mathrm{0}}\end{cases} \\ $$$$\underset{{n}\geqslant\mathrm{1}} {\sum}{f}\left({n}\right)=\underset{{n}\geqslant\mathrm{0}} {\sum}{f}^{\ast} \left({n}\right) \\ $$$${abel}\:{plana}\:{formula} \\ $$$$\underset{{n}\geqslant\mathrm{0}} {\sum}\overset{\ast} {{f}}\left({n}\right)=\int_{\mathrm{0}} ^{\infty} {f}\left({z}\right){dz}+\frac{{f}\left(\mathrm{0}\right)}{\mathrm{2}}+{i}\int_{\mathrm{0}} ^{\infty} \frac{{f}\left({it}\right)−{f}\left(−{it}\right)}{{e}^{\mathrm{2}\pi{t}} −\mathrm{1}}{dt} \\ $$$$=\int_{\mathrm{0}} ^{\infty} \frac{{z}^{\mathrm{13}} }{{e}^{\mathrm{2}\pi{z}} −\mathrm{1}}{dz}+{i}\int_{\mathrm{0}} ^{\infty} \mathrm{2}{iIm}\left({f}\left({it}\right)\right).\frac{{dt}}{{e}^{\mathrm{2}\pi{t}} −\mathrm{1}} \\ $$$$\frac{{it}^{\mathrm{13}} }{{e}^{\mathrm{2}{i}\pi{t}} −\mathrm{1}}+\frac{{it}^{\mathrm{13}} }{{e}^{−\mathrm{2}{i}\pi{t}} −\mathrm{1}}={f}\left({it}\right)−{f}\left(−{it}\right)=\frac{{it}^{\mathrm{13}} }{{e}^{\mathrm{2}\pi{t}} −\mathrm{1}}\left(\mathrm{1}−{e}^{\mathrm{2}\pi{it}} \right)=−{it}^{\mathrm{13}} \\ $$$$=\mathrm{2}\int_{\mathrm{0}} ^{\infty} \frac{{z}^{\mathrm{13}} }{{e}^{\mathrm{2}\pi{z}} −\mathrm{1}}{dz} \\ $$$$\mathrm{2}\pi{z}={t}\Rightarrow{dz}=\frac{{dt}}{\mathrm{2}\pi},=\mathrm{2}\int_{\mathrm{0}} ^{\infty} \left(\frac{{t}}{\mathrm{2}\pi}\right)^{\mathrm{13}} .\frac{{dt}}{\mathrm{2}\pi\left({e}^{{t}} −\mathrm{1}\right)} \\ $$$$=\frac{\mathrm{1}}{\pi^{\mathrm{14}} .\mathrm{2}^{\mathrm{13}} }\int_{\mathrm{0}} ^{\infty} \frac{{t}^{\mathrm{13}} }{{e}^{{t}} −\mathrm{1}}{dt}={S} \\ $$$$\int_{\mathrm{0}} ^{\infty} \frac{{t}^{{s}} }{{e}^{{t}} −\mathrm{1}}{dt}=\underset{{n}\geqslant\mathrm{0}} {\sum}\int_{\mathrm{0}} ^{\infty} {t}^{{s}} {e}^{−\left(\mathrm{1}+{n}\right){t}} {dt}=\underset{{n}\geqslant\mathrm{0}} {\sum}\Gamma\left({s}+\mathrm{1}\right)\frac{\mathrm{1}}{\left({n}+\mathrm{1}\right)^{{s}+\mathrm{1}} } \\ $$$$=\Gamma\left({s}+\mathrm{1}\right)\zeta\left({s}+\mathrm{1}\right) \\ $$$${S}=\frac{\mathrm{1}}{\pi^{\mathrm{14}} \mathrm{2}^{\mathrm{13}} }\zeta\left(\mathrm{14}\right)\Gamma\left(\mathrm{14}\right)=\frac{\mathrm{1}}{\pi^{\mathrm{14}} .\mathrm{2}^{\mathrm{13}} }.\frac{\mathrm{2}\pi^{\mathrm{14}} }{\mathrm{18243225}}.\left(\mathrm{13}\right)! \\ $$$$\left.=\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{12}} }.\frac{\left(\mathrm{13}\right)!}{\mathrm{25}.\mathrm{13}.\mathrm{7}.\mathrm{9}^{\mathrm{3}} .\mathrm{11}}=\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{12}} }.\left(\mathrm{12}.\mathrm{10}.\mathrm{8}.\mathrm{6}.\mathrm{4}.\mathrm{3}.\mathrm{2}\right).\frac{\mathrm{1}}{\mathrm{5}.\mathrm{9}^{\mathrm{2}} }\right) \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{12}} }\left(\mathrm{3}.\mathrm{2}.\mathrm{2}.\mathrm{5}.\mathrm{2}^{\mathrm{3}} .\mathrm{2}.\mathrm{3}.\mathrm{2}^{\mathrm{3}} .\mathrm{3}\right).\frac{\mathrm{1}}{\mathrm{5}.\mathrm{3}^{\mathrm{4}} }=\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{12}} }.\left(\mathrm{2}^{\mathrm{9}} \right).\frac{\mathrm{1}}{\mathrm{3}}=\frac{\mathrm{1}}{\mathrm{8}.\mathrm{3}} \\ $$$$=\frac{\mathrm{1}}{\mathrm{24}} \\ $$$$\underset{{n}\geqslant\mathrm{1}} {\sum}\frac{{n}^{\mathrm{13}} }{{e}^{\mathrm{2}\pi{n}} −\mathrm{1}}=\frac{\mathrm{1}}{\mathrm{24}}\:{I}\:{tried}\:{many}\:{ways}\:{to}\:\:{get}\:{it} \\ $$$$ \\ $$
Commented by Dwaipayan Shikari last updated on 14/Nov/20

$${Great}\:{sir}!\:{Thanking}\:{you} \\ $$
